Electrode potential for Mg electrode varies according to the equation
EMg2+∣Mg=EϴMg2+∣Mg−0.0592log1[Mg2+]
The graph of EMg2+∣Mgvslog[Mg2+] is
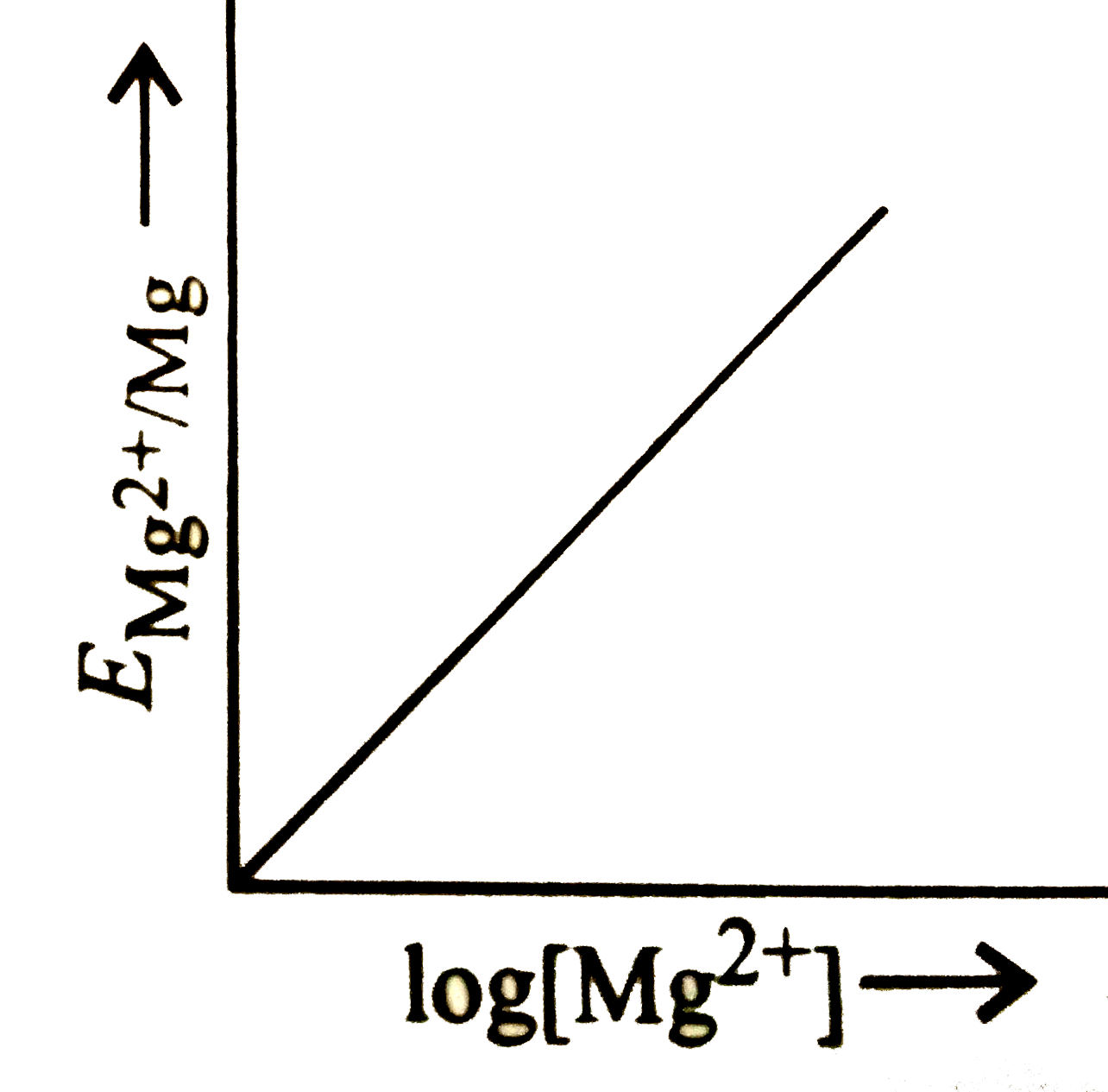
The correct Answer is:B
To solve the problem, we need to analyze the given equation for the electrode potential of the magnesium electrode and determine the nature of the graph of EMg2+|Mg versus log(Mg2+).
1. Understanding the Nernst Equation:
The Nernst equation for the magnesium electrode is given as:
EMg2+|Mg=E∘Mg2+|Mg−0.0592log(1(Mg2+))
This can be rewritten as:
EMg2+|Mg=E∘Mg2+|Mg+0.0592log(Mg2+)
2. Identifying the Variables:
In this equation, we can identify:
- E (the electrode potential) corresponds to EMg2+|Mg
- log(Mg2+) is the independent variable (let's denote it as x)
- The slope m of the line is 0.0592
- The y-intercept c is E∘Mg2+|Mg
3. Formulating the Linear Equation:
The equation can be expressed in the form of a linear equation y=mx+c:
E=0.0592log(Mg2+)+E∘Mg2+|Mg
Here, y is E, m is 0.0592, x is log(Mg2+), and c is E∘Mg2+|Mg.
4. Graph Characteristics:
- The graph of E versus log(Mg2+) will be a straight line.
- The slope of the line is positive since 0.0592 is a positive value.
- The y-intercept will be at E∘Mg2+|Mg.
5. Conclusion:
Therefore, the graph of EMg2+|Mg versus log(Mg2+) is a straight line with a positive slope.
The Nernst equation for the magnesium electrode is given as:
EMg2+|Mg=E∘Mg2+|Mg−0.0592log(1(Mg2+))
This can be rewritten as:
EMg2+|Mg=E∘Mg2+|Mg+0.0592log(Mg2+)
In this equation, we can identify:
- E (the electrode potential) corresponds to EMg2+|Mg
- log(Mg2+) is the independent variable (let's denote it as x)
- The slope m of the line is 0.0592
- The y-intercept c is E∘Mg2+|Mg
The equation can be expressed in the form of a linear equation y=mx+c:
E=0.0592log(Mg2+)+E∘Mg2+|Mg
Here, y is E, m is 0.0592, x is log(Mg2+), and c is E∘Mg2+|Mg.
- The graph of E versus log(Mg2+) will be a straight line.
- The slope of the line is positive since 0.0592 is a positive value.
- The y-intercept will be at E∘Mg2+|Mg.
Therefore, the graph of EMg2+|Mg versus log(Mg2+) is a straight line with a positive slope.
Topper's Solved these Questions
- ELECTROCHEMISTRYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - ASSERTION & REASON
15 Videos
- ELECTROCHEMISTRYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - Electrochemical Cell
1 Videos
- ELECTROCHEMISTRYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - HOTS
10 Videos
- COORDINATION COMPOUNDS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - COORDINATION COMPOUNDS EXERCISE - Assertion And Reason
15 Videos
- GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - GENERAL PRINCIPLES AND PROCESSES OF ISOLATION OF ELEMENTSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
Knowledge Check
Electrode potential for Mg electrode varies according to the equation
EMg2+/Mg=EΘMg2+/Mg−0.0592log1[Mg2+]
The graph of EMg2+/Mgvslog[Mg2+] isA B
B C
C D
D
Mg liberates H2 on reaction with
View SolutionMg liberates H2 on reaction with
View SolutionWhy does magnesium form Mg2+ and not Mg+?
View SolutionWhy does magnesium from Mg2+ and not Mg+?
View SolutionSelect the basic and acidic radicals in the Mg(OH)2.
View SolutionBalance the equation
Mg+HNO3→Mg(NO3)2+N2O+H2O
View SolutionHow would you determine the standard reduction potential of the system Mg2+∣Mg?
View Solution
