The capacitance of a parallel plate capacitor with air as medium is with the introduction of a dielectric medium between the plates, the capacitance becomes The permittivity of the medium is
The correct Answer is:C
To find the permittivity of the dielectric medium introduced between the plates of a parallel plate capacitor, we can follow these steps:
Step 1: Understand the relationship between capacitance and dielectric constant
The capacitance C of a parallel plate capacitor is given by the formula:
C=Aϵ0d
where:
- A is the area of the plates,
- ϵ0 is the permittivity of free space (approximately 8.85×10−12F/m),
- d is the separation between the plates.
When a dielectric material is introduced, the capacitance increases to:
C′=Aϵd=K⋅C
where K is the dielectric constant of the material, and ϵ=Kϵ0.
Step 2: Set up the equations with given values
From the problem, we know:
- The initial capacitance C=3μF=3×10−6F
- The capacitance with the dielectric C′=15μF=15×10−6F
Using the relationship between the capacitances:
K=C′C=15×10−63×10−6=5
Step 3: Calculate the permittivity of the dielectric medium
The permittivity ϵ of the dielectric medium can be expressed as:
ϵ=Kϵ0
Substituting the values:
ϵ=5⋅(8.85×10−12F/m)
Step 4: Perform the calculation
Calculating ϵ:
ϵ=5⋅8.85×10−12=44.25×10−12F/m
or
ϵ=0.4425×10−10F/m
Final Result
The permittivity of the dielectric medium is:
ϵ≈4.425×10−11F/m
The capacitance C of a parallel plate capacitor is given by the formula:
C=Aϵ0d
where:
- A is the area of the plates,
- ϵ0 is the permittivity of free space (approximately 8.85×10−12F/m),
- d is the separation between the plates.
C′=Aϵd=K⋅C
where K is the dielectric constant of the material, and ϵ=Kϵ0.
From the problem, we know:
- The initial capacitance C=3μF=3×10−6F
- The capacitance with the dielectric C′=15μF=15×10−6F
K=C′C=15×10−63×10−6=5
The permittivity ϵ of the dielectric medium can be expressed as:
ϵ=Kϵ0
Substituting the values:
ϵ=5⋅(8.85×10−12F/m)
Calculating ϵ:
ϵ=5⋅8.85×10−12=44.25×10−12F/m
or
ϵ=0.4425×10−10F/m
The permittivity of the dielectric medium is:
ϵ≈4.425×10−11F/m
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:NCERT FINGERTPS ENGLISHChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:HOTS
Explore 5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:NCERT FINGERTPS ENGLISHChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:EXEMPLAR PROBLEMS
Explore 3 Videos
- ELECTROMAGNETIC WAVESBook:NCERT FINGERTPS ENGLISHChapter:ELECTROMAGNETIC WAVESExercise:Assertion And Reason
Explore 15 Videos
- MAGNETISM AND MATTER Book:NCERT FINGERTPS ENGLISHChapter:MAGNETISM AND MATTER Exercise:NCERT Exemplar
Explore 5 Videos
Similar Questions
The capacitance of a parallel plate capacitor with air as Medium is 6μF. With the introduction of a dielectric medium,the capacitance becomes 30μF. The permittivity of the medium is:
View SolutionTo reduce the capacitance of parallel plate capacitor, the space between the plate is
View SolutionThe capacitance of a parallel plate capacitor is 12μF. If the distance between the plates is doubled and area is halved, then new capacitance will be
View SolutionThe capacitance of a parallel plate capacitor is 2μF and the charge on its positive plate is 2μC. If the charge on its plates is doubled, the capacitance of the capacitor
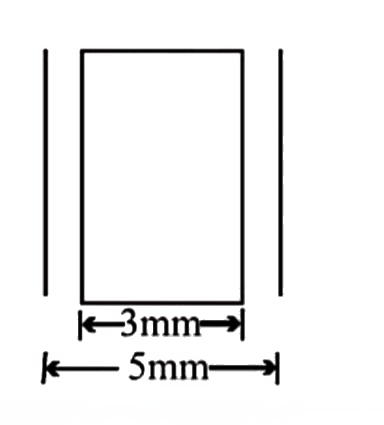
View SolutionSeparation between the plates of parallel plate capacitor is 5 mm. this capacitor, having air as the dielectric medium between the plates, is charged to a potential difference 25 V using a battery. The battery is then disconnected and a dielectric slab of thickness 3mm and dielectric constant K=10 is placed between the plates as shown. potential difference between the plates after the dielectric slab has been introduced is-
View SolutionA parallel plate capacitor, with plate area A and plate separation d, is filled with a dielectric slabe as shown. What is the capacitance of the arrangement ?
View SolutionThe capacitance of a parallel plate capacitor is 16μF. When a glass slab is placed between the plates, the potential difference reduces to 1/8th of the original value. What is dielectric constant of glass ?
View Solution(b) Obtain the expression for the capacitance of a parallel plate capacitor.
View SolutionA parallel plate air capacitor has a capacitance of 5μF. It becomes 50μF when a dielectric medium occupies the entire space between its two plates. What is the dielectric constant of the medium ?
View SolutionSeparation between the plates of a parallel plate capacitor is d and the area of each plates is A. When a slab of material of dielectric constant k and thickness t(t<d) is introduced between the plates. Its capacitance becomes
View Solution