The work done in carrying a charge q once round a circle of radius r with a charge Q at the centre is
qQ4πε0a
qQ4πε0a2
q4πε0a
zero
The correct Answer is:D
To solve the problem of finding the work done in carrying a charge q once around a circle of radius r with a charge Q at the center, we can follow these steps:
1. Understanding the Setup:
- We have a charge Q located at the center of a circle.
- We are moving a charge q along the circumference of this circle.
2. Electric Potential Due to Charge Q:
- The electric potential V at any point on the circumference of the circle (distance r from charge Q) is given by the formula:
V=kQr
where k is Coulomb's constant.
3. Potential at Different Points:
- Since the charge q is moved along a circular path, every point on the circumference is at the same distance r from the charge Q.
- Therefore, the electric potential V is constant along the entire circular path.
4. Calculating Potential Difference:
- The potential difference ΔV between any two points on the circle is:
ΔV=Va−Vb=0
because Va and Vb are equal (both equal to kQr).
5. Work Done Calculation:
- The work done W in moving the charge q around the circle is given by:
W=q⋅ΔV
- Substituting ΔV=0:
W=q⋅0=0
6. Conclusion:
- The work done in carrying the charge q once around the circle is 0.
Final Answer:
The work done in carrying a charge q once round a circle of radius r with a charge Q at the center is 0.
---
- We have a charge Q located at the center of a circle.
- We are moving a charge q along the circumference of this circle.
- The electric potential V at any point on the circumference of the circle (distance r from charge Q) is given by the formula:
V=kQr
where k is Coulomb's constant.
- Since the charge q is moved along a circular path, every point on the circumference is at the same distance r from the charge Q.
- Therefore, the electric potential V is constant along the entire circular path.
- The potential difference ΔV between any two points on the circle is:
ΔV=Va−Vb=0
because Va and Vb are equal (both equal to kQr).
- The work done W in moving the charge q around the circle is given by:
W=q⋅ΔV
- Substituting ΔV=0:
W=q⋅0=0
- The work done in carrying the charge q once around the circle is 0.
The work done in carrying a charge q once round a circle of radius r with a charge Q at the center is 0.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - HOTS
5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - EXEMPLAR PROBLEMS
3 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason
15 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar
5 Videos
Similar Questions
What is the amount of work done in moving a point charge around a circular are of radius r at the centre where another point charge is located ?
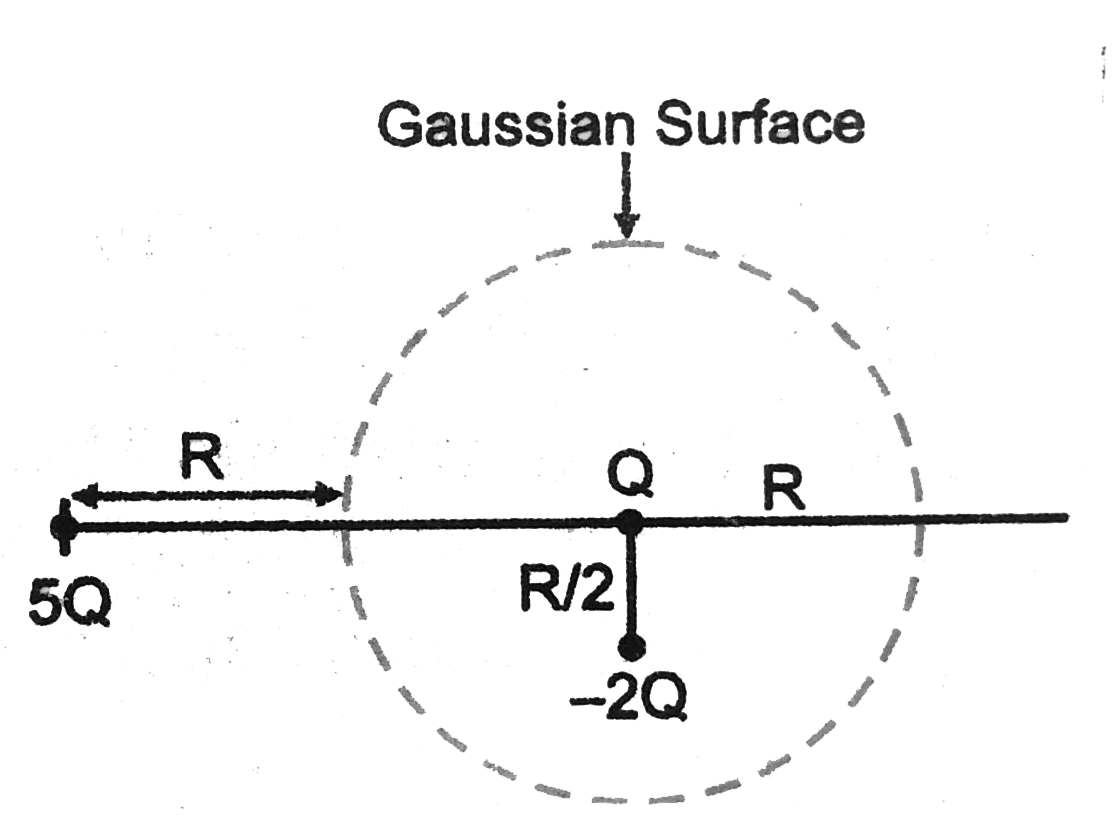
View SolutionRefer to the arrangement of charges in Fig and a Gaussian surface of radius R with Q at the centre. Then
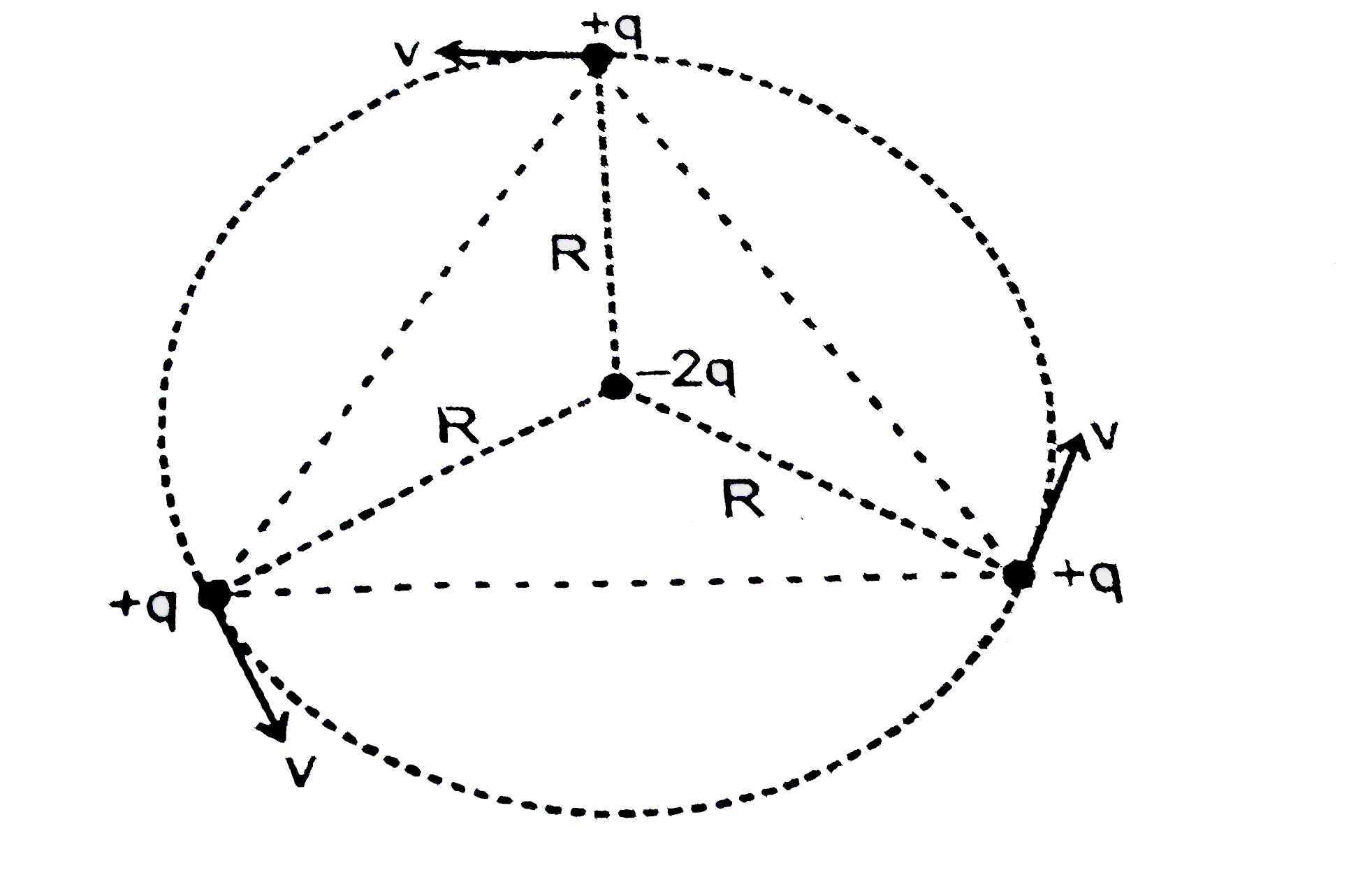
View SolutionThree equal point charges of charge +q each are moving along a circle of radius R and a point charge -2q is also placed at the centre of circle (as shown in figure). If charge are revolving with constant and same speed in the circle then calculate speed of charges
View Solutionn charged drops, each of radius r and charge q, coalesce to from a big drop of radius R and charge Q. If V is the electric potential and E is the electric field at the surface of a drop, then.
View Solutiona. A circle is drawn with center as a charge +q. What is the work done in moving a charge +q from B to C along the circumferebce of the circle ?
B In the above question, if the charge +q is first taken from B to A and then from A to C, on which path is the magnitude of work greater?
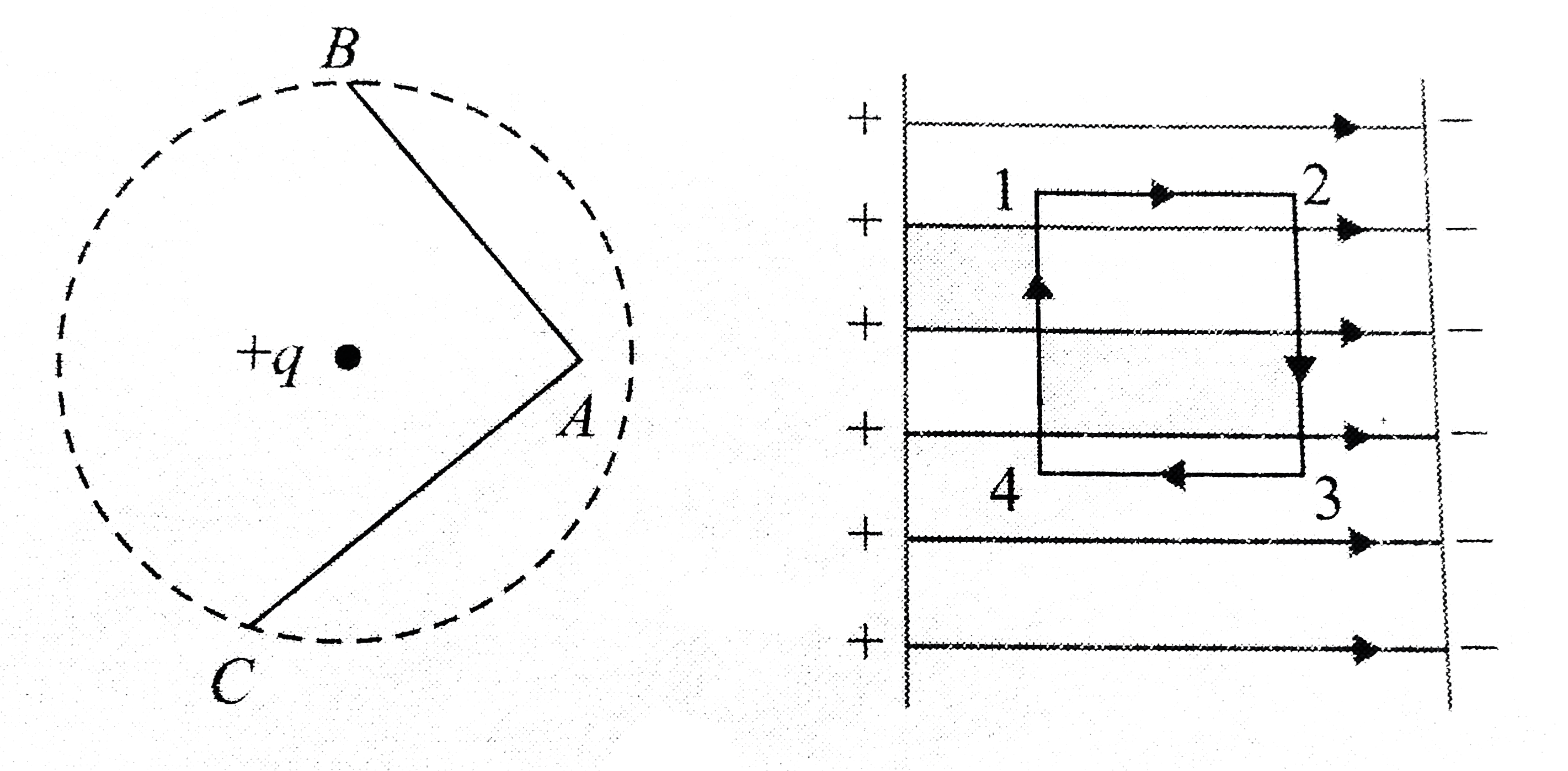
View SolutionTwo identical thin ring, each of radius R meters, are coaxially placed a distance R metres apart. If Q1 coulomb, and Q2 coulomb, are repectively the charges uniformly spread on the two rings, the work done in moving a charge q from the centre of one ring to that of the other is
View SolutionTwo identical thin ring, each of radius R meters, are coaxially placed a distance R metres apart. If Q1 coulomb, and Q2 coulomb, are repectively the charges uniformly spread on the two rings, the work done in moving a charge q from the centre of one ring to that of the other is
View SolutionFind the electric work done in bringing a charge q from A to B in a sphere of charge Q distributed uniformly throughout its volume.
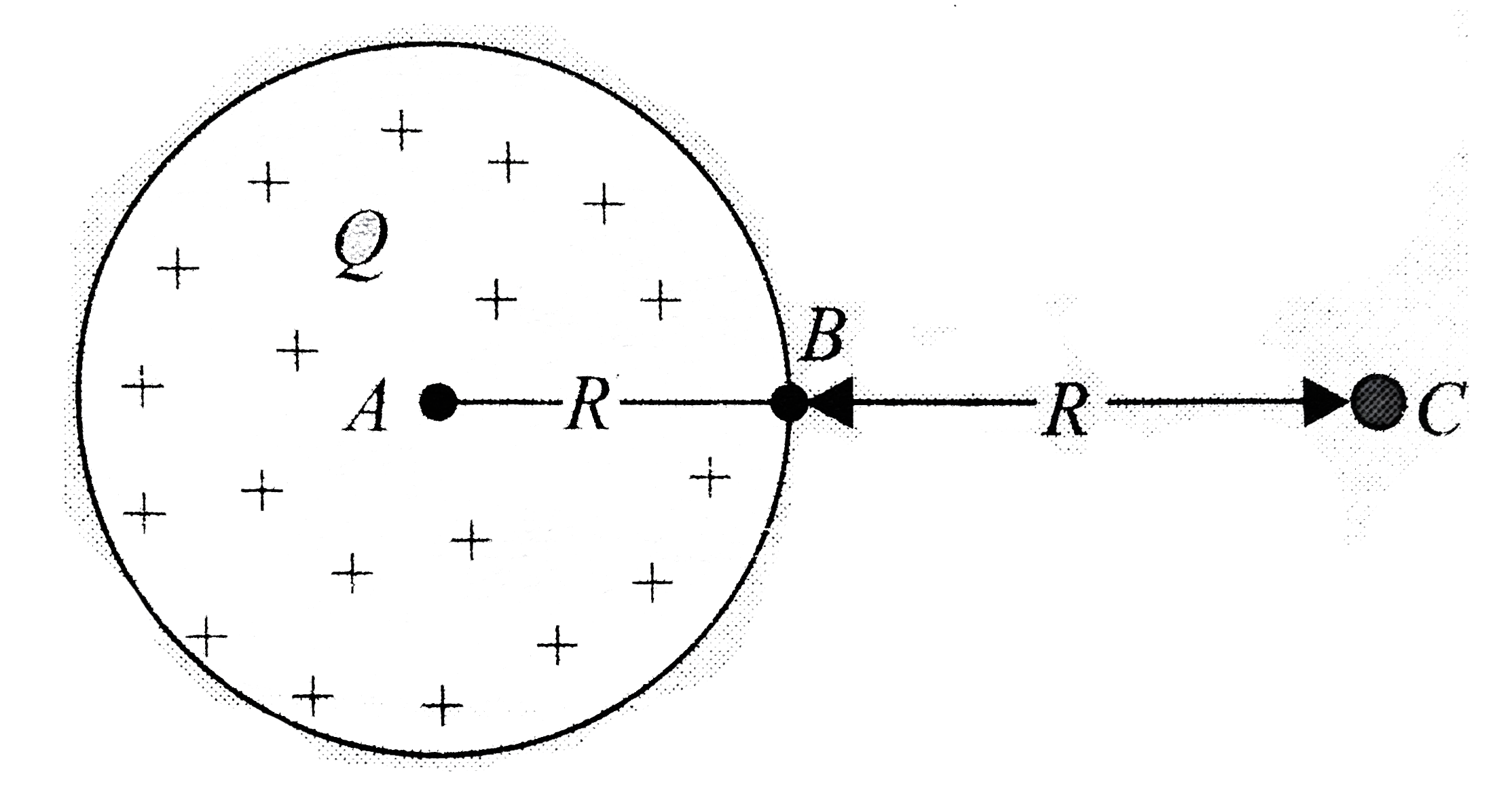 .
.
View SolutionWork done in moving a charge q coulomb on the surface of given charged conductor of potential V is
View SolutionA charged particle (charge q) is moving in a circle of radius R with unifrom speed v. The associated magnetic moment μ is given by
View Solution
