Equipotential surfaces
are closer in regions of large electric fields compared to regions of lower electric fields
will be more crowded near sharp edges of a conductor
will always be equally spaced
both (a) and (b) are correct
The correct Answer is:D
Electric field,E=−dVdrordr∝1E
i.e., equipotential surfaces are closer in regions of large electric fields compared to regions of lower electric field. At sharp edges of a conductor, charge density is more . Therefore electric field is stronger. Hence equipotential surfaces are more crowded.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:NCERT FINGERTPS ENGLISHChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:HOTS
Explore 5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE Book:NCERT FINGERTPS ENGLISHChapter:ELECTROSTATIC POTENTIAL AND CAPACITANCE Exercise:EXEMPLAR PROBLEMS
Explore 3 Videos
- ELECTROMAGNETIC WAVESBook:NCERT FINGERTPS ENGLISHChapter:ELECTROMAGNETIC WAVESExercise:Assertion And Reason
Explore 15 Videos
- MAGNETISM AND MATTER Book:NCERT FINGERTPS ENGLISHChapter:MAGNETISM AND MATTER Exercise:NCERT Exemplar
Explore 5 Videos
Similar Questions
(i) Two isolated metal spheres A and B have radii R and 2R respectively and same charge q. Find which of the two spheres have
(a) greater capacitance
(b) greater energy density just outside the surface of the spheres.
(ii) (a) Show that the equipotential surfaces are closed together in the regions of strong field and far apart in the region of weak field. Draw equipotential surfaces for an electric dipole.
(b) Concentric equipotential surfaces due to a charged body placed at the centre are shown. Identify the polarity of the charge and draw the electric field lines due to it.
View SolutionThree equal charges are placed at the corners of an equilateral triangle. Which of the graph below correctly depicts the equally-spaced equipotential surfaces in the plane of the triangle? (All graph have the same scale).
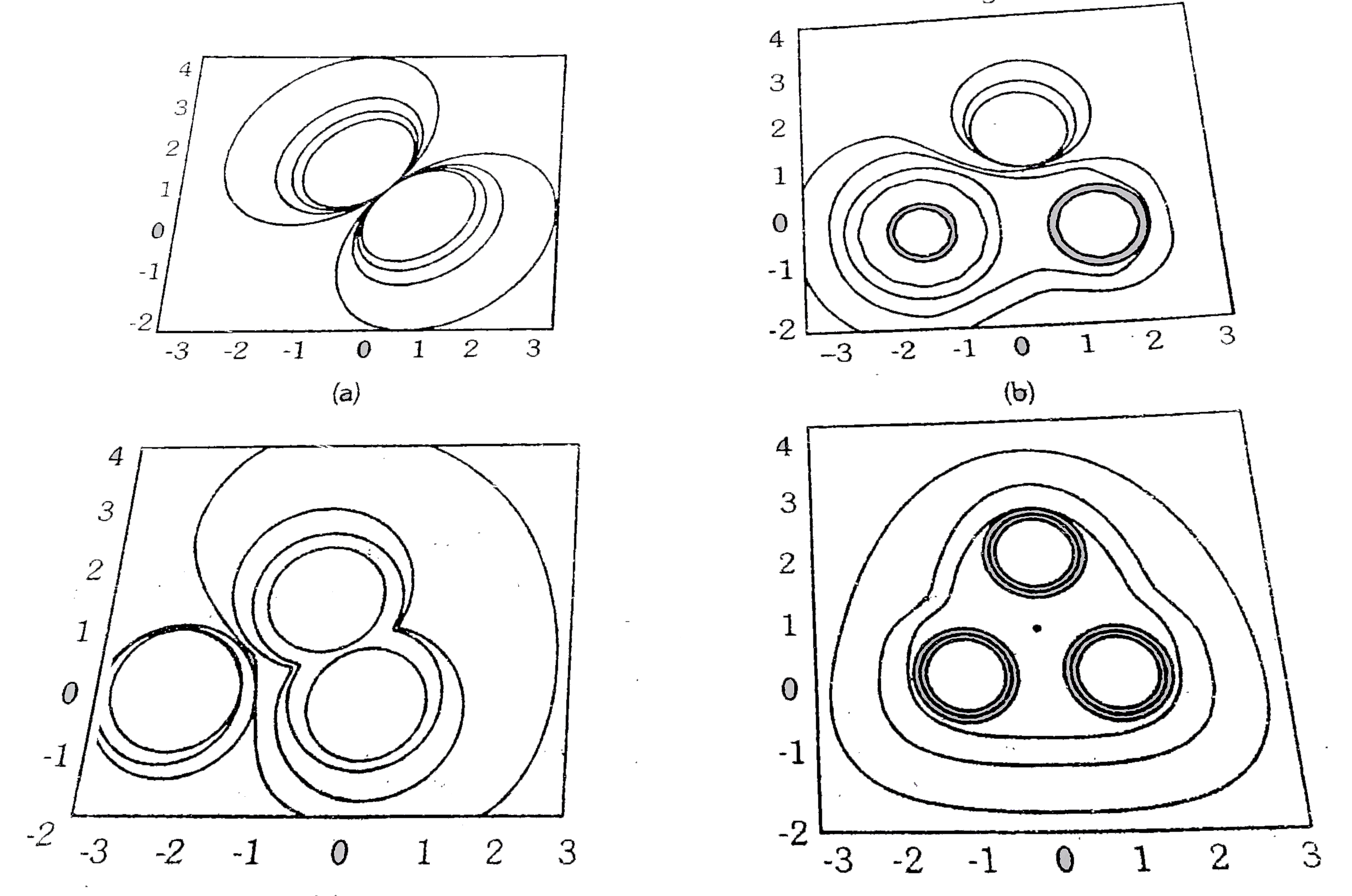
View Solution
Knowledge Check
The angle between the equipotential surface and the electric field (or line of force) at any point on the equipotential surface is
A90∘ alwaysB0∘ alwaysC0∘ to 90∘D0∘ to 180∘
In an electric field shown in figure, three equipotential figure, three equipotential surfaces are shown. If function are shown. If function of electric field is E=2x2Vm−1, and given that V1−V2=V2−V3, then we have

View Solution(a) Define electric dipole moment. Is it a scalar or a vector ? Derive the expression for the electric field of a dipole at a point on the equatorial plane of the dipole.
(b) Draw the equipotential surfaces due to an electric dipole. Locate the points where the potential due to the dipole is zero.
View SolutionS1: When a positively charged particle is released in an electric field , in its subsequent motion,it may or may not move along the electric field line passing through the point it has been released.
S2: In electrostatic, conductors are always equipotential surfaces.
S3: The flux through a closed Gaussian surface is non-zero. The electric field at some point on the Gaussian surface may be zero.
View SolutionElectric lines of force always leave an equipotential surface
View SolutionAssertion (A) A spherical equipotential surface is not possible for a point charge.
Reason (R ) A spherical equipotential surface is possible inside a spherical capacitor.
View SolutionNature of equipotential surface for a point charge is
View SolutionAssertion: Electric field is aways directed perpendicular to an equipotential surface.
Reason: Equipotential surface is a surface on which at each point potential is same.
View Solution
