A hydrogen atom initially in the ground level absorbs a photon and is excited to n=4 level then the wavelength of photon is
790Å
870Å
970Å
1070Å
The correct Answer is:C
To find the wavelength of the photon absorbed by a hydrogen atom to excite it from the ground state (n=1) to the n=4 level, we can use the Rydberg formula for hydrogen:
1λ=R(1n21−1n22)
Where:
- λ is the wavelength of the emitted or absorbed photon,
- R is the Rydberg constant (R≈1.097×107m−1),
- n1 is the lower energy level (initial state),
- n2 is the higher energy level (final state).
1. Identify the initial and final energy levels:
- The hydrogen atom starts at the ground state, so n1=1.
- The atom is excited to the n=4 level, so n2=4.
2. Substitute the values into the Rydberg formula:
1λ=R(112−142)
3. Calculate the terms:
- 112=1
- 142=116
4. Combine the fractions:
1λ=R(1−116)=R(1616−116)=R(1516)
5. Substitute the value of R:
1λ=R⋅1516=1.097×107⋅1516
6. Calculate 1λ:
1λ=1.097×107⋅1516=1.097×107⋅0.9375≈1.028×107m−1
7. Find λ:
λ=11.028×107≈9.73×10−8m
8. Convert to Angstroms:
- Since 1m=1010Å:
λ≈9.73×10−8m×1010Å/m≈973Å
Final Answer:
The wavelength of the photon absorbed by the hydrogen atom is approximately 970Å.
- λ is the wavelength of the emitted or absorbed photon,
- R is the Rydberg constant (R≈1.097×107m−1),
- n1 is the lower energy level (initial state),
- n2 is the higher energy level (final state).
- The hydrogen atom starts at the ground state, so n1=1.
- The atom is excited to the n=4 level, so n2=4.
1λ=R(112−142)
- 112=1
- 142=116
1λ=R(1−116)=R(1616−116)=R(1516)
1λ=R⋅1516=1.097×107⋅1516
1λ=1.097×107⋅1516=1.097×107⋅0.9375≈1.028×107m−1
λ=11.028×107≈9.73×10−8m
- Since 1m=1010Å:
λ≈9.73×10−8m×1010Å/m≈973Å
The wavelength of the photon absorbed by the hydrogen atom is approximately 970Å.
Topper's Solved these Questions
- ATOMS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ATOMS EXERCISE - Higher order thinking skills
8 Videos
- ATOMS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ATOMS EXERCISE - NCERT Examplar problems
7 Videos
- ALTERNATING CURRENT BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ALTERNATING CURRENT EXERCISE - Assertion And Reason
15 Videos
- COMMUNITCATION SYSTEMSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - COMMUNITCATION SYSTEMSEXERCISE - Assertion And Reason
30 Videos
Similar Questions
A hydrogen atom initially in the ground level absorbs a photon, Which excites it to n=4 level. Determine the wavelength and frequency of photon.
View SolutionA hydrogen atom initially in the ground state absorbs a photon which excites it to then n = 4 level . Estimate the frequeny of the photon.
View SolutionA hydrogen atom initially in the ground state is excited by absorbing a photon of wavelenght 980 Å.The redius of the atom in the excited state ,in terms of Bohr radius a0 will be : (hc=12500eV−Å)
View SolutionThe electron in the first excited state (n1=2) of H- atom absorbs a photon and is further excited to nth shell. The de-Broglie wavelength of the electron in this excited state is 1340 pm. Find the value of n.
View SolutionIf the energy difference between the ground state of an atom and in excited state is 4.4×10−14J, the wavelength of photon required to produce the transition is:
View SolutionAn electron in the first excited state of H atom absorbed a photon and further excited. The de broglie wavelength of the electron in this state is found to be 13.4 Å. Find the wavelength of the photon absorbed by the electron in angstroms. Also find the longest and the shortest wavelength emitted when this electron de-excited back to the ground state.
View SolutionA hydrogen like atom in ground st6ate abserbs n photon having the same energy and its emit exacity n photon when electron transition tekes placed .Then the energy of the absorbed photon may be
View SolutionThe follwing diagram indicates the energy levels of a certain atom when the system moves from 2E level to E, a photon of wavelength λ is emitted. The wavelength of photon produced during its transition from 4E3 level to E is
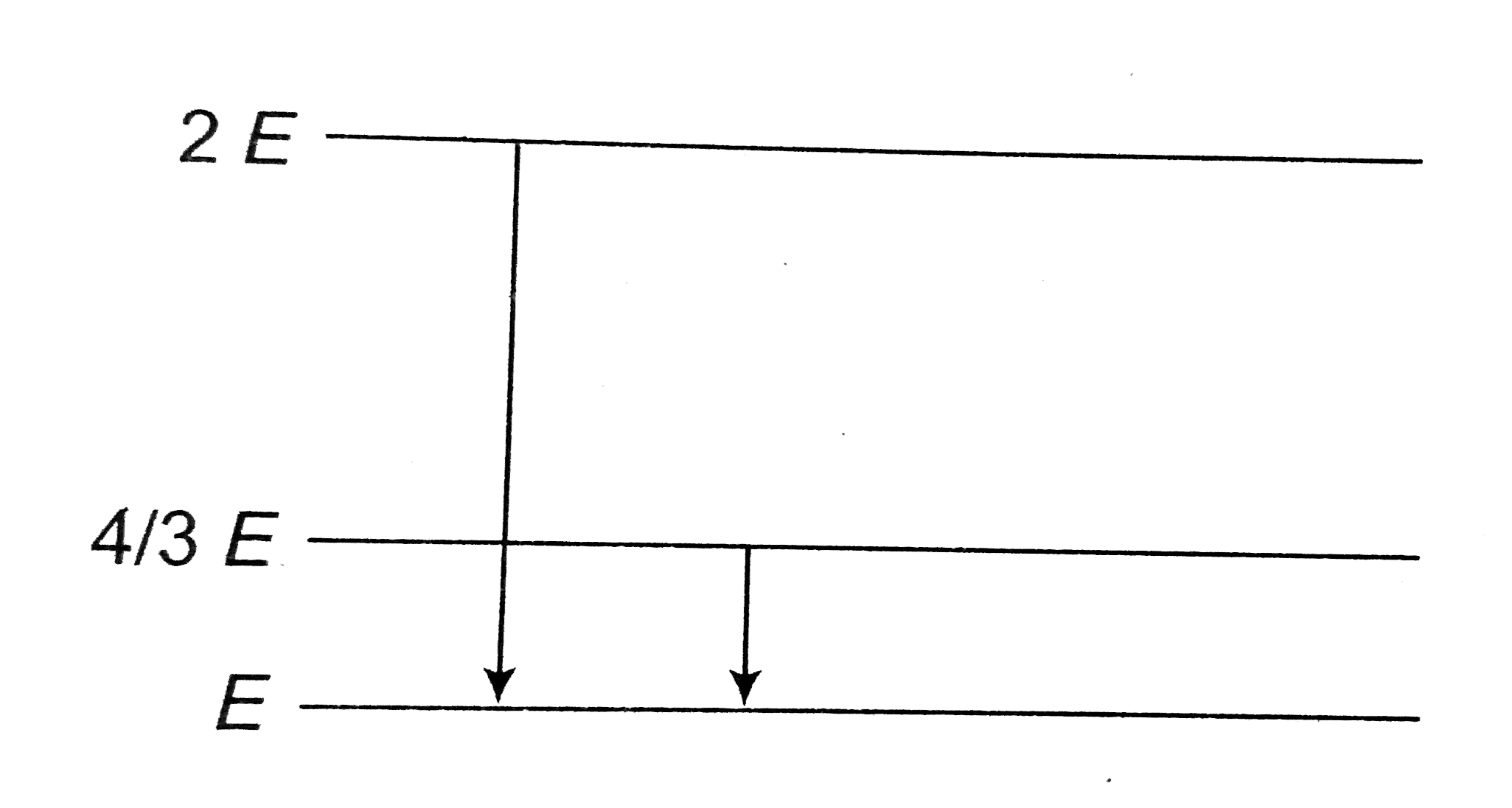
View SolutionWhen hydrogen atom is in its first excited level, its radius is
View SolutionA hydrogen atom in ground state obsebe a photon of ultraviolet radition of wavelength 50nm Assuming that the entire photon energy is taken up by the electron with what kinetic energy will the up by the electron with what kinetic energy will the electron be ejected?
View Solution
