Assertion: A rigid body not fixed in some way can have either pure translation or a combination of translation and rotation.
Reason: In rotation about a fixed axis, every particle of the rigid body moves in a circle which lies in a plane perpendicular to the axis and has its centre on the axis.
If both assertion and reason are true and reason is the correct explanation of assertion.
If both assertion and reason are true but reason is not the correct explanation of assertion
If assertion is true but reason is false.
If both assertion and reason are false.
The correct Answer is:b
To analyze the given assertion and reason, we will break down the concepts step by step.
Step 1: Understand the Assertion
The assertion states that "A rigid body not fixed in some way can have either pure translation or a combination of translation and rotation."
- A rigid body is defined as an object with a fixed shape that does not deform under the influence of forces.
- If a rigid body is not fixed, it can move freely. This means it can either:
- Move in a straight line (pure translation).
- Rotate about an axis while also translating (combination of translation and rotation).
Step 2: Understand the Reason
The reason states that "In rotation about a fixed axis, every particle of the rigid body moves in a circle which lies in a plane perpendicular to the axis and has its center on the axis."
- When a rigid body rotates about a fixed axis, each particle of the body follows a circular path.
- The plane of rotation is perpendicular to the axis of rotation, and the center of each circular path lies on the axis of rotation.
Step 3: Analyze the Relationship
Now we need to analyze the relationship between the assertion and the reason:
- The assertion talks about the motion of a rigid body that is not fixed, allowing for both translational and rotational movement.
- The reason describes the behavior of a rigid body that is fixed at an axis, which is a specific case of rotational motion.
Step 4: Conclusion
Both the assertion and the reason are true statements. However, the reason does not directly explain the assertion. The assertion discusses the general case of motion for a non-fixed rigid body, while the reason specifically addresses fixed axis rotation.
Final Answer
The correct conclusion is:
- Both the assertion and reason are true, but the reason is not the correct explanation of the assertion.
The assertion states that "A rigid body not fixed in some way can have either pure translation or a combination of translation and rotation."
- If a rigid body is not fixed, it can move freely. This means it can either:
- Move in a straight line (pure translation).
- Rotate about an axis while also translating (combination of translation and rotation).
The reason states that "In rotation about a fixed axis, every particle of the rigid body moves in a circle which lies in a plane perpendicular to the axis and has its center on the axis."
- The plane of rotation is perpendicular to the axis of rotation, and the center of each circular path lies on the axis of rotation.
Now we need to analyze the relationship between the assertion and the reason:
- The reason describes the behavior of a rigid body that is fixed at an axis, which is a specific case of rotational motion.
Both the assertion and the reason are true statements. However, the reason does not directly explain the assertion. The assertion discusses the general case of motion for a non-fixed rigid body, while the reason specifically addresses fixed axis rotation.
The correct conclusion is:
- Both the assertion and reason are true, but the reason is not the correct explanation of the assertion.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - Kinematics Of Rotational Motion About A Fixed Axis
3 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - Higher Order Thinking Skills
8 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONSEXERCISE - NCER EXAMPLAR PROBLEMS
8 Videos
- PRACTICE PAPERSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPERSEXERCISE - All Questions
150 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Assertion And Reason
10 Videos
Similar Questions
Knowledge Check
Assertion: The motion of a ceiling fan is rotational only.
Reason: The motion of a rigid body which is pivoted fixed of rotation.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertionCIf assertion is true but reason is false.DIf both assertion and reason are false.Assertion: If the head of a right handed screw rotates with the body, the screw advances in the direction of the angular velocty.
Reason: For rotation about a fixed axis, the angular velocity vector lies along the axis of rotation.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertionCIf assertion is true but reason is false.DIf both assertion and reason are false.Two thin discs each of mass M and radius r metre are attached to form a rigid body as shown in figure. The rotational inertia of this body about an axis perpendicular to the plane of disc B and passing through its centre is
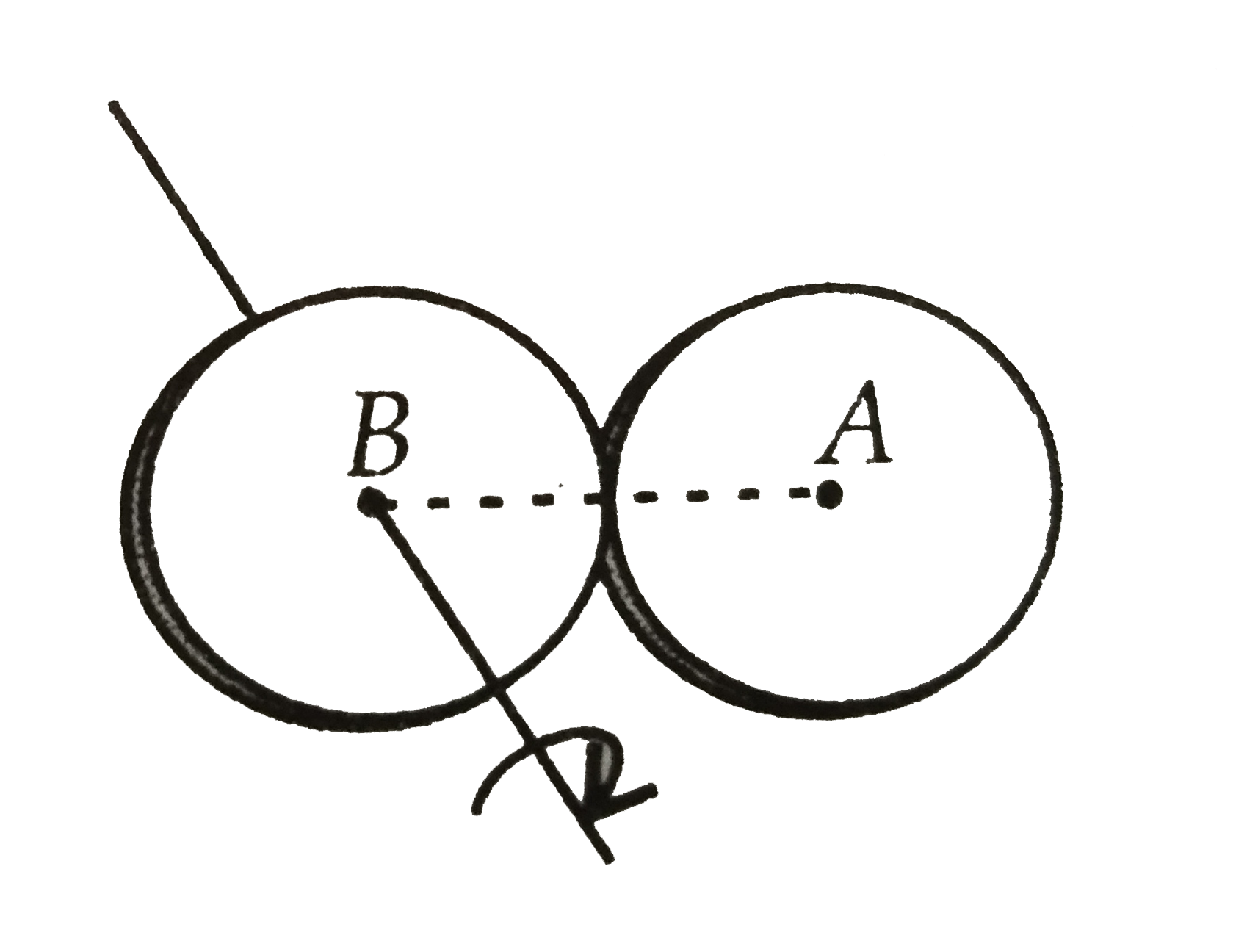 A2Mr2B3Mr2C4Mr2D5Mr2
A2Mr2B3Mr2C4Mr2D5Mr2
Assertion : If the earth stops rotating about its axis, the value of the weight of the body at equator will decrease.
Reason : The centripetal force does not act on the body at the equator.
View SolutionA : When a rigid body rotates about any fixed axis, then all the particles of it move in circles of different radii but with same angular velocity.
R : In rigid body relative position of particles are fixed.
View SolutionA body is rotating anonuniformly abut a vertical axis fixed in an inertial frame. The resultant force on a particle of the body of thebody not on the axis is
View SolutionAssertion : Speed of any point on rigid body executing rolling motion can be calculated by expression v=rω, where r is distance of point from instantaneous centre of rotation
Reason : Rolling motion of rigid body can be considered as a pure rotation about instantaneous centre of rotation.
View SolutionAssertion: Value of radius of gyration of a body depends on axis of rotation.
Reason: Radius of gyration is root mean square distance of particle of the body from the axis of rotation.
View Solution
