Question

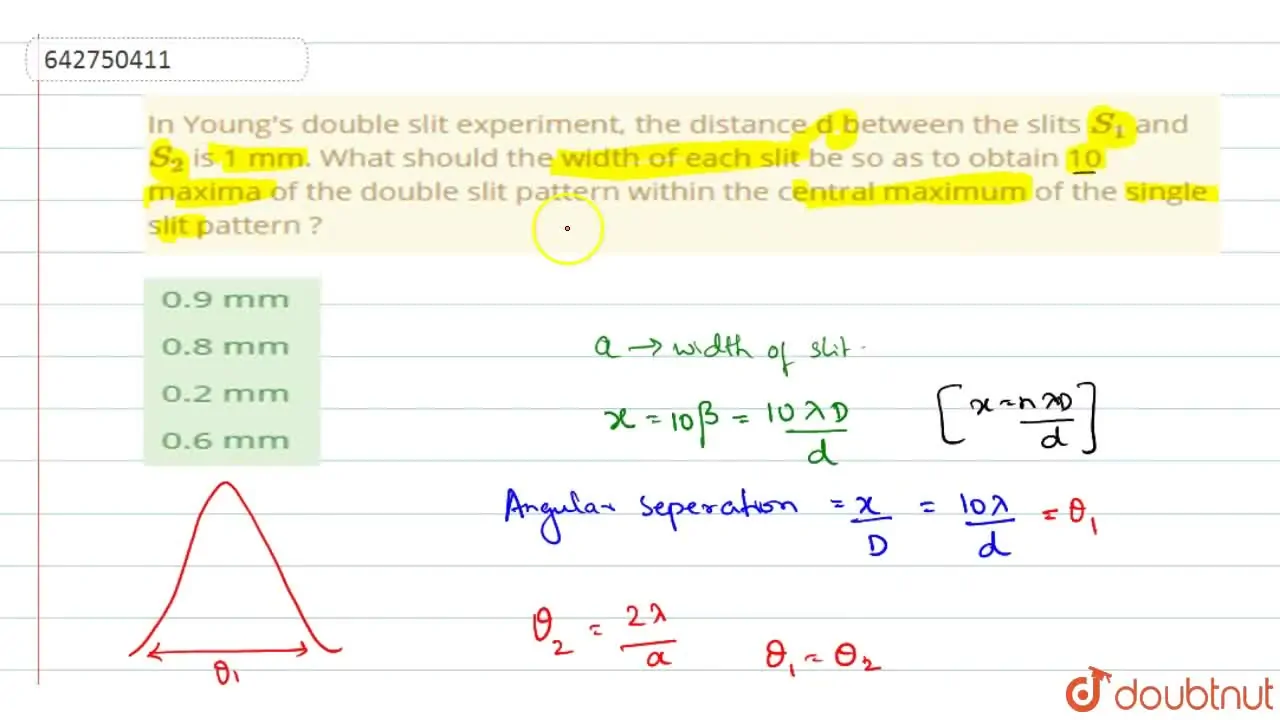
In Young's double slit experiment, the distance d between the slits $S_1$ and $S_2$ is 1 mm. What should the width of each slit be so as to obtain 10 maxima of the double slit pattern within the central maximum of the single slit pattern ?

Options

A. 0.9 mm
B. 0.8 mm
C. 0.2 mm
D. 0.6 mm

Answer

C

Explanation

To solve the problem, we need to determine the width of each slit in Young's double slit experiment such that there are 10 maxima of the double slit pattern within the central maximum of the single slit pattern.

1. Understanding the Setup:
- We have a double slit experiment with slits \( S_1 \) and \( S_2 \) separated by a distance \( d = 1 \, \text{mm} = 1 \times 10^{-3} \, \text{m} \).
- We need to find the width \( a \) of each slit such that there are 10 maxima of the double slit pattern within the central maximum of the single slit diffraction pattern.

2. Expression for Double Slit Maxima:
- The position of the \( n \)-th maximum in a double slit experiment is given by:
\( X_n = \frac{n \lambda D}{d} \)
- Here, \( n \) is the order of the maximum, \( \lambda \) is the wavelength of light, \( D \) is the distance from the slits to the screen, and \( d \) is the distance between the slits.

3. Finding the Total Width for 10 Maxima:
- For 10 maxima, we set \( n = 10 \):
\( X_{10} = \frac{10 \lambda D}{d} \)

4. Angular Width of Central Maximum in Single Slit:
- The angular width of the central maximum in a single slit diffraction pattern is given by:
\( \theta = \frac{2 \lambda}{a} \)
- This means the width of the central maximum is determined by the width of the slit \( a \).

5. Setting Angular Widths Equal:
- For the condition that the 10 maxima fit within the central maximum, we equate the angular width of the central maximum from the single slit to the angular separation of the double slit maxima:
\( \frac{2 \lambda}{a} = \frac{10 \lambda}{d} \)

6. Solving for Slit Width \( a \):
- Rearranging the equation gives:
\( 2 \lambda \cdot d = 10 \lambda \cdot a \)
- Dividing both sides by \( \lambda \) (assuming \( \lambda \neq 0 \)):
\( 2d = 10a \)
- Solving for \( a \):
\( a = \frac{2d}{10} = \frac{d}{5} \)

7. Substituting the Value of \( d \):
- Now substituting \( d = 1 \, \text{mm} = 1 \times 10^{-3} \, \text{m} \):
\( a = \frac{1 \times 10^{-3}}{5} = 0.2 \times 10^{-3} \, \text{m} = 0.2 \, \text{mm} \)

8. Final Answer:
- The width of each slit should be \( 0.2 \, \text{mm} \).

Conclusion:
The width of each slit required to obtain 10 maxima of the double slit pattern within the central maximum of the single slit pattern is 0.2 mm.