In a series LCR circuit the voltage across an inductor, capacitor and resistor are 20 V, 20 V and 40 V respectively. The phase difference between the applied voltage and the current in the circuit is
30∘
45∘
60∘
0∘
The correct Answer is:D
To find the phase difference between the applied voltage and the current in a series LCR circuit where the voltages across the inductor (V_L), capacitor (V_C), and resistor (V_R) are given, we can follow these steps:
1. Identify the Given Values:
- Voltage across the inductor, VL=20V
- Voltage across the capacitor, VC=20V
- Voltage across the resistor, VR=40V
2. Understand the Relationship in LCR Circuits:
- In a series LCR circuit, the total voltage across the circuit is the vector sum of the voltages across the inductor, capacitor, and resistor.
- The phase difference ϕ between the total voltage and the current can be calculated using the formula:
tanϕ=VL−VCVR
3. Substitute the Values into the Formula:
- Substitute the known values into the equation:
tanϕ=20V−20V40V
4. Calculate tanϕ:
- Simplifying the equation gives:
tanϕ=040=0
5. Determine the Phase Difference ϕ:
- Since tanϕ=0, we know that:
ϕ=0∘
6. Conclusion:
- The phase difference between the applied voltage and the current in the circuit is 0∘.
Final Answer:
The phase difference between the applied voltage and the current in the circuit is 0∘.
---
- Voltage across the inductor, VL=20V
- Voltage across the capacitor, VC=20V
- Voltage across the resistor, VR=40V
- In a series LCR circuit, the total voltage across the circuit is the vector sum of the voltages across the inductor, capacitor, and resistor.
- The phase difference ϕ between the total voltage and the current can be calculated using the formula:
tanϕ=VL−VCVR
- Substitute the known values into the equation:
tanϕ=20V−20V40V
- Simplifying the equation gives:
tanϕ=040=0
- Since tanϕ=0, we know that:
ϕ=0∘
- The phase difference between the applied voltage and the current in the circuit is 0∘.
The phase difference between the applied voltage and the current in the circuit is 0∘.
Topper's Solved these Questions
- ALTERNATING CURRENT Book:NCERT FINGERTPS ENGLISHChapter:ALTERNATING CURRENT Exercise:HOTS
Explore 8 Videos
- ALTERNATING CURRENT Book:NCERT FINGERTPS ENGLISHChapter:ALTERNATING CURRENT Exercise:NCERT
Explore 7 Videos
- ATOMS Book:NCERT FINGERTPS ENGLISHChapter:ATOMS Exercise:Assertion And Reason
Explore 15 Videos
Similar Questions
In a series LCR circuit, the voltages across an inductor, a capacitor and a resistor are 30 V, 30 V, 60 V respectively. What is the phase difference between the applied voltage and the current in the circuit ?
View SolutionIn a series LCR circuit, the voltages across an inductor, a capacitor and a resistor are 30 V, 30 V, 60 V respectively. What is the phase difference between the applied voltage and the current in the circuit ?
View Solution
Knowledge Check
Phase difference between voltage and current in a capacitor in an ac circuit is
AπBπ/2C0Dπ/3In a series LCR circuit, the phase difference between the voltage and the current is 45∘. Then the power factor will be
A0.607B0.707C0.808D1
The phase difference between voltage and current in series L-C circuit is
View SolutionIn a series resonant circuit, the ac, voltage across resistance R, inductance L and capacitance are 5V, 10V and 10V, respectively. The ac. voltage applied to the circuit will be
View SolutionIn a series LCR circuit the rms voltage across the inductance , capacitance and resistance are respectively 4 V, 8 V and 5 V . The RMS voltage of the AC source in the circuit is
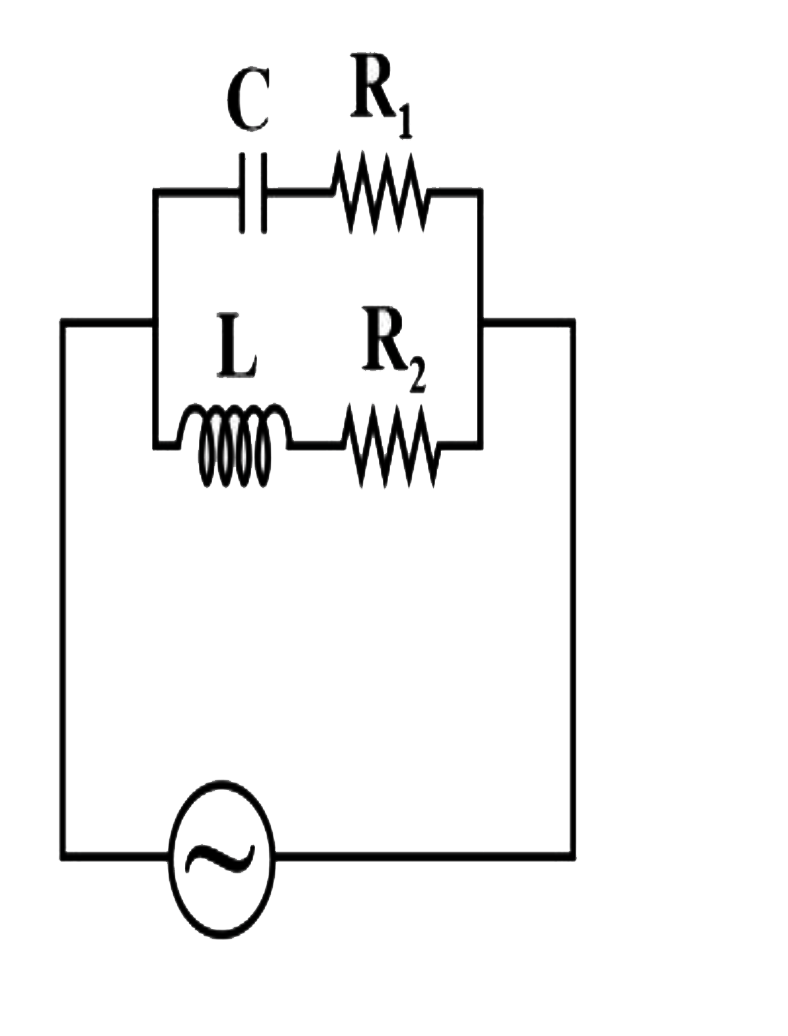
View SolutionIn the given circuit the R.M.S values of voltages across the capacitor C, inductor L and resistor R1 are 12V, 10V and 5 V respectively. Then the peak voltage across R2 is
View SolutionIn an LCR series ac circuit the voltage across L,C and R are V1,V2 and V3 respectively The voltage of the source is .
View SolutionIn an L-C-R circuit the AC voltage across R, L and C comes out as 10 V , 10 V and 20 V respectively . The voltage across the enter combination will be
View Solution