A capillary tube (A) is dipped in water. Another identical tube (B) is dipped in a soap-water solution. Which of the following shows the relative nature of the liquid columns in the two tubes?


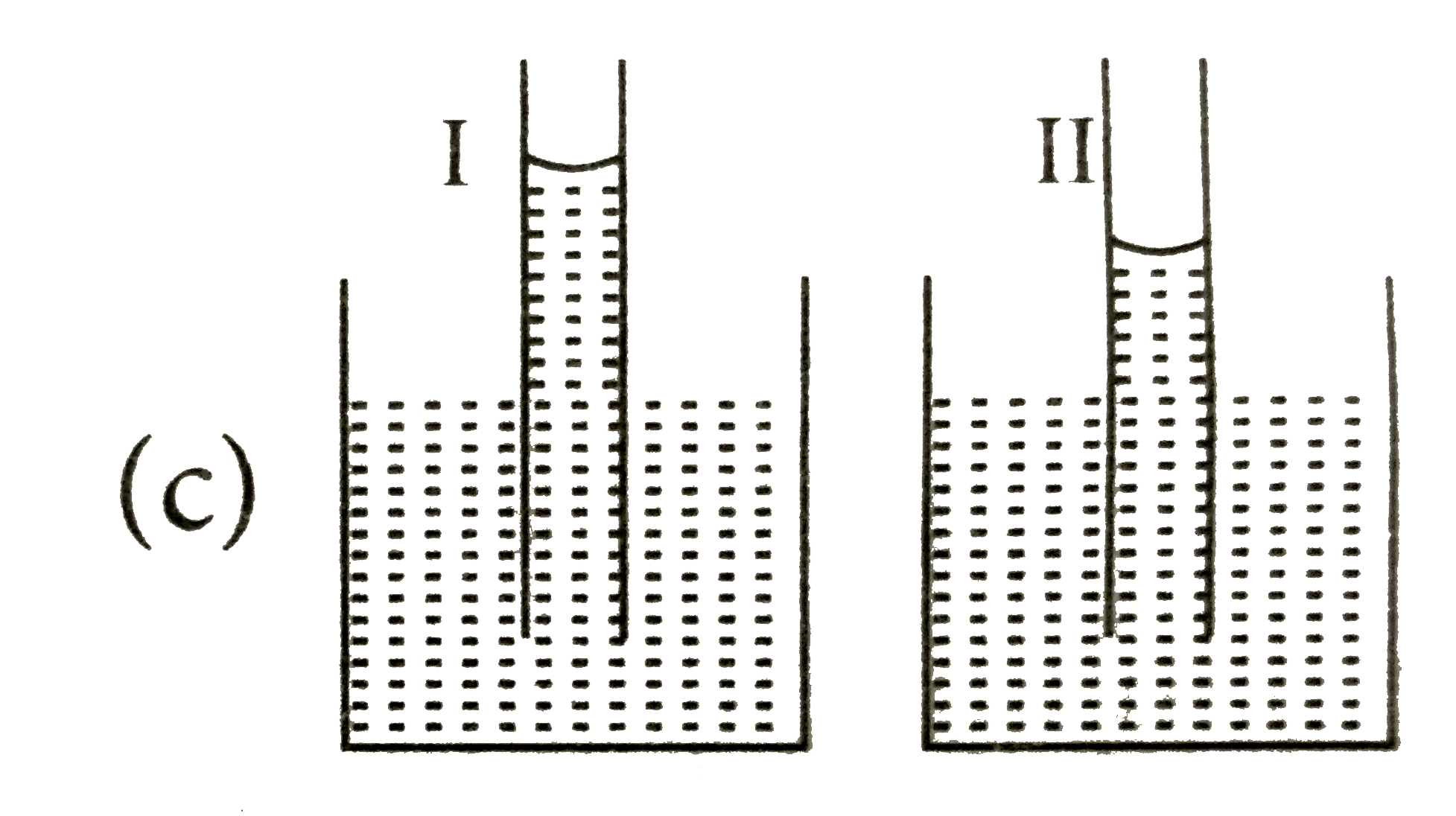

The correct Answer is:C
To solve the problem of comparing the liquid columns in two identical capillary tubes, one dipped in water and the other in a soap-water solution, we can follow these steps:
Step 1: Understand Capillary Action
Capillary action occurs due to the interplay of cohesive and adhesive forces in a liquid. When a liquid is in contact with a solid surface (like the walls of a capillary tube), the adhesive forces between the liquid and the solid can cause the liquid to rise in the tube.
Step 2: Identify the Liquids
- Tube A: Dipped in water.
- Tube B: Dipped in a soap-water solution.
Step 3: Analyze Surface Tension
Surface tension plays a crucial role in capillary action. Water has a relatively high surface tension, while soap-water solutions have a lower surface tension due to the presence of surfactants.
Step 4: Apply the Capillary Rise Formula
The height of the liquid column (h) in a capillary tube can be described by the formula:
h=2TcosθRρg
Where:
- T = surface tension of the liquid
- θ = contact angle
- R = radius of the tube
- ρ = density of the liquid
- g = acceleration due to gravity
Step 5: Compare the Two Tubes
- For Tube A (water): Water has a higher surface tension, leading to a certain height of rise.
- For Tube B (soap-water solution): The surface tension is lower, which means the height of the liquid column will be less compared to that in tube A.
Step 6: Conclusion
Since the surface tension of the soap-water solution is lower than that of water, the liquid column in Tube A (water) will rise higher than in Tube B (soap-water solution). Therefore, the relative heights of the liquid columns will show that Tube A has a higher liquid column than Tube B.
Final Answer
The correct option is that the liquid column in Tube A (water) will be higher than the liquid column in Tube B (soap-water solution).
---
Capillary action occurs due to the interplay of cohesive and adhesive forces in a liquid. When a liquid is in contact with a solid surface (like the walls of a capillary tube), the adhesive forces between the liquid and the solid can cause the liquid to rise in the tube.
- Tube A: Dipped in water.
- Tube B: Dipped in a soap-water solution.
Surface tension plays a crucial role in capillary action. Water has a relatively high surface tension, while soap-water solutions have a lower surface tension due to the presence of surfactants.
The height of the liquid column (h) in a capillary tube can be described by the formula:
h=2TcosθRρg
Where:
- T = surface tension of the liquid
- θ = contact angle
- R = radius of the tube
- ρ = density of the liquid
- g = acceleration due to gravity
- For Tube A (water): Water has a higher surface tension, leading to a certain height of rise.
- For Tube B (soap-water solution): The surface tension is lower, which means the height of the liquid column will be less compared to that in tube A.
Since the surface tension of the soap-water solution is lower than that of water, the liquid column in Tube A (water) will rise higher than in Tube B (soap-water solution). Therefore, the relative heights of the liquid columns will show that Tube A has a higher liquid column than Tube B.
The correct option is that the liquid column in Tube A (water) will be higher than the liquid column in Tube B (soap-water solution).
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Higher Order Thinking Skills
8 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Exemplar Problems
5 Videos
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
15 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
If the capillary tube is dipped in water is of insufficient length (l<h),will water overflow?
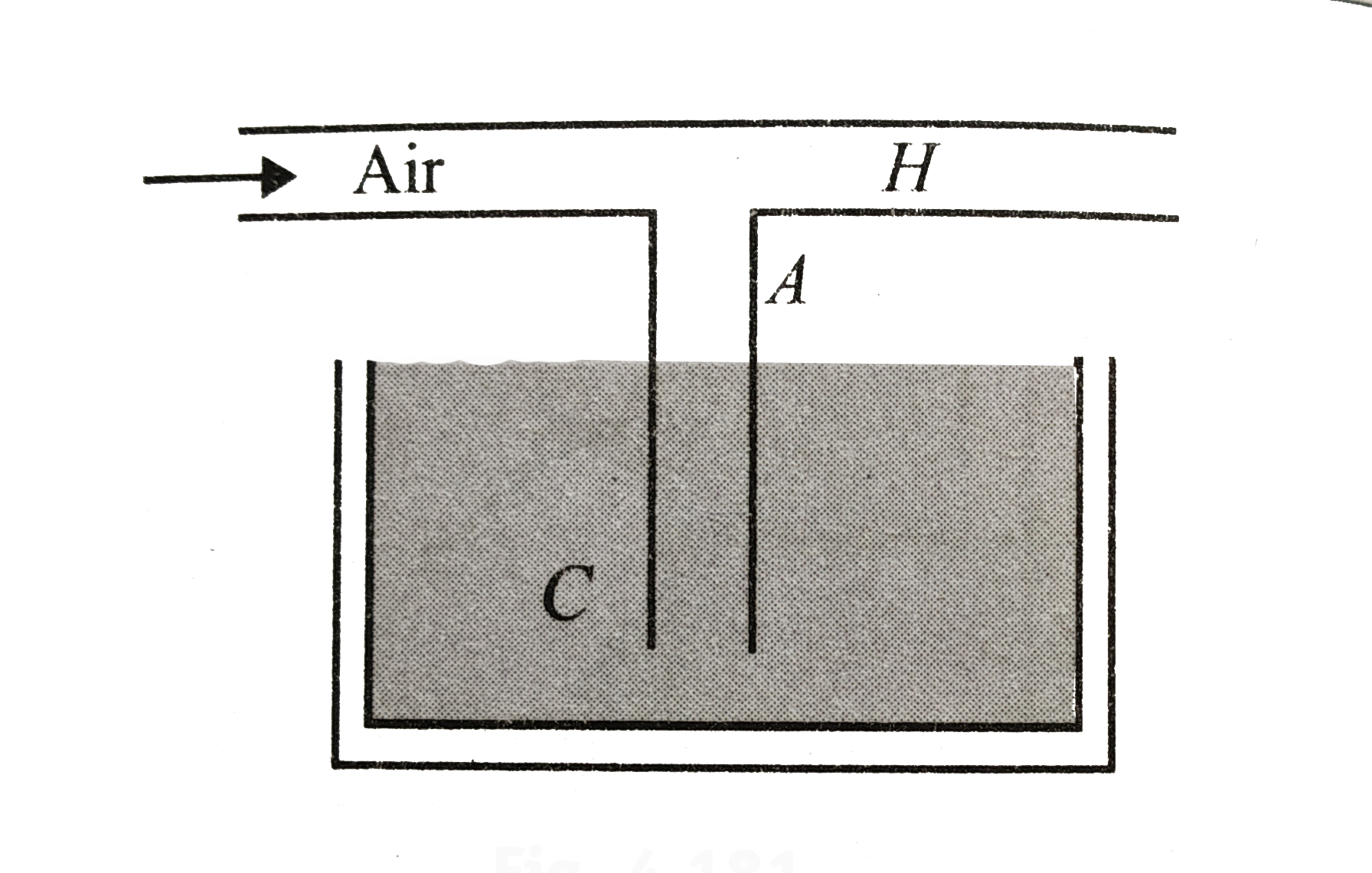
View SolutionFigure shows a capillary tube C dipped in a liquid that wets it. The liquid rises to a point A. If we blow air through the horizontal tube H, what will happen to the liquid column in the capillary tube?
View SolutionA 20 cm long capillary tube is dipped in water. The water rises up to 8 cm. If the entire arrangement is put in a freely falling elevator, the length of water column in the capillary tube will be
View SolutionIf a wax coated capillary tube is dipped in water, then water in it will-
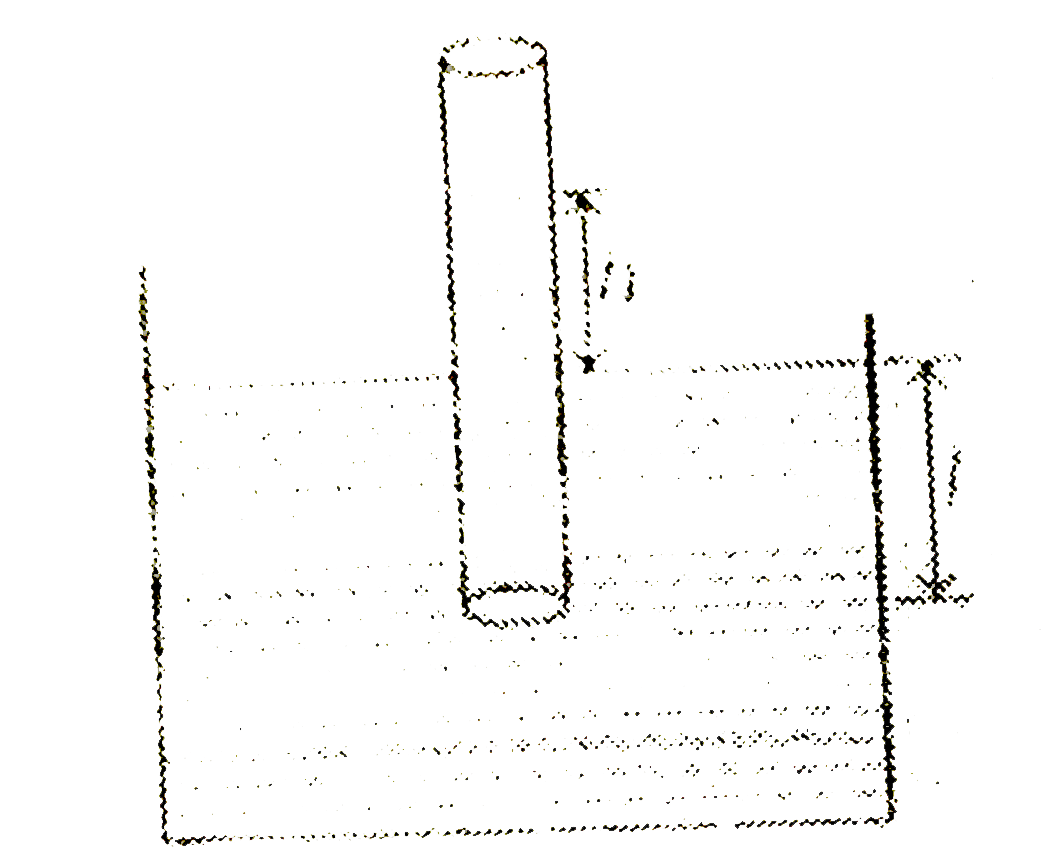
View SolutionA capillary tube is dipped in water to a depth and the water rises to a height h(<l) in the capillary tube. The lower end of the tube is closed in water by putting a lower over it. The tube is now taken out and the thumb is removed from the lower end and it kept open. The length of liquid column in the tube will be
View SolutionThe lower end of a glass capillary tube is dipped in water. Water rises to a height of 9 cm. The tube is then broken at a height of 5 cm. The height of the water column and angle of contact will be
View SolutionA vertical glass capillary tube, open at both ends, contains some water. Which of the following shapes may not be taken by the water -in the tube?
View SolutionWhen a capillary tube is dipped into a liquid, the liquid neither rises nor falls in the capillary.
View SolutionA cylindrical tube open at both ends, has a fundamental frequency f in air. The tube is dipped vertically in air. The tube is dipped vertically in water so that half of it is in water. The fundamental frequency of the air column is now
View SolutionA capillary tube is dipped in water vertically. It is long enough for the water to rise to the maximum height h in the tube. The length of portion immersed in water is I<h. The lower end of the tube is closed and then the tube is taken out and opened again. Will all the water flow out of tube? Explain.
View Solution
