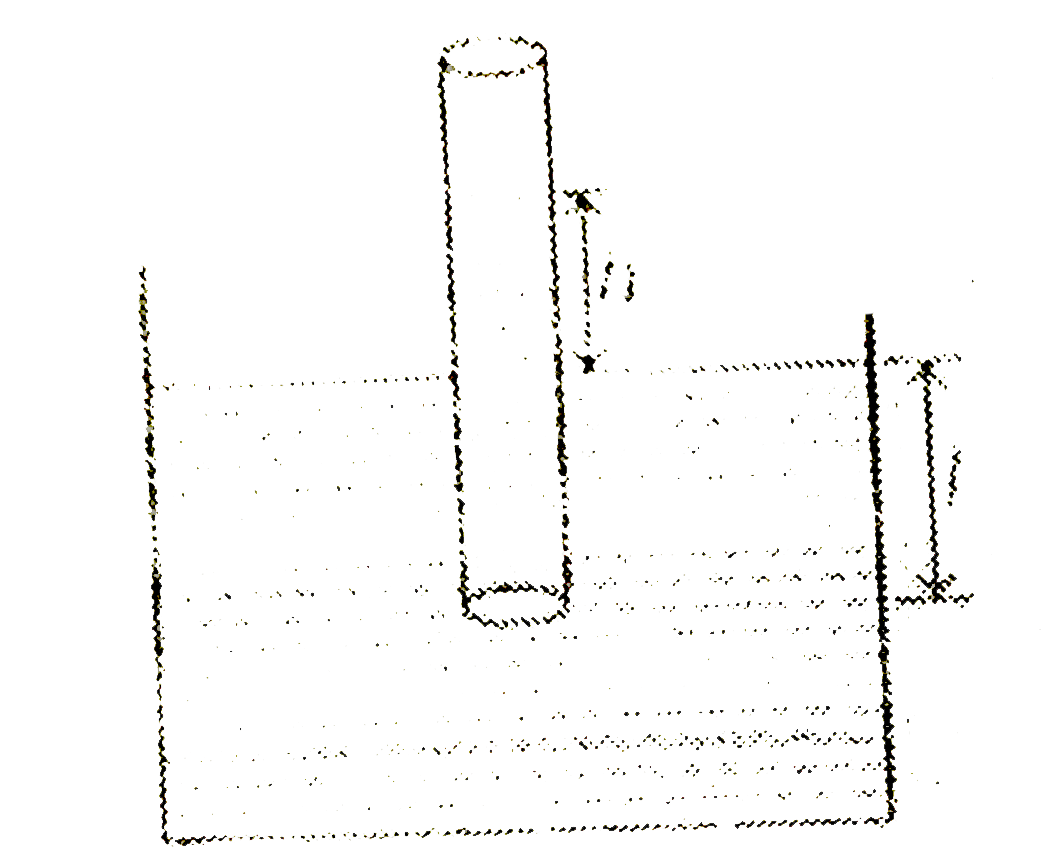
When a capillary tube is dipped in a liquid, the liquid rises to a height H in the tube. The free liquid surface inside the tube is hemispherical in shape. The tube is now pushed down sothat the height of the tube outside the liquid is less than H. Then the
liquid will come out of the tube like in a small fountain
liquid will ooze out of the tube slowly
free liquid surface inside the tube is hemispherical
the liquid will fill the tube but not come out its upper end.
The correct Answer is:D
To solve the problem regarding the behavior of a liquid in a capillary tube when the tube is pushed down, we need to analyze the situation step by step.
1. Understand the Initial Condition:
When the capillary tube is dipped into the liquid, the liquid rises to a height H due to capillary action. The free surface of the liquid inside the tube forms a hemispherical shape.
2. Identify the Forces at Play:
The rise of the liquid in the capillary tube is due to the balance of cohesive and adhesive forces. The adhesive forces between the liquid and the walls of the tube cause the liquid to rise, while the cohesive forces within the liquid resist this rise.
3. Apply the Capillary Rise Formula:
The height H to which the liquid rises in a capillary tube can be calculated using the formula:
H=2Scosθρgr
where S is the surface tension of the liquid, θ is the contact angle, ρ is the density of the liquid, g is the acceleration due to gravity, and r is the radius of the tube.
4. Consider the New Condition:
When the tube is pushed down so that the height of the tube outside the liquid is less than H, the liquid will still remain in the tube up to the height H unless the pressure conditions change.
5. Analyze the Pressure Changes:
As the tube is pushed down, the pressure at the bottom of the liquid column inside the tube will change. The pressure at a depth h in a liquid is given by:
P=P0+ρgh
where P0 is the atmospheric pressure.
6. Determine the New Equilibrium:
If the height of the liquid column inside the tube is still H, the pressure at the bottom of the liquid column inside the tube must equal the pressure exerted by the liquid column plus the atmospheric pressure. If the tube is pushed down too far, the liquid may no longer be able to maintain the height H due to the external pressure being greater than the internal pressure.
7. Conclusion:
If the tube is pushed down such that the height of the liquid column is less than H, the liquid will drop until a new equilibrium is reached where the pressure balance is maintained.
When the capillary tube is dipped into the liquid, the liquid rises to a height H due to capillary action. The free surface of the liquid inside the tube forms a hemispherical shape.
The rise of the liquid in the capillary tube is due to the balance of cohesive and adhesive forces. The adhesive forces between the liquid and the walls of the tube cause the liquid to rise, while the cohesive forces within the liquid resist this rise.
The height H to which the liquid rises in a capillary tube can be calculated using the formula:
H=2Scosθρgr
where S is the surface tension of the liquid, θ is the contact angle, ρ is the density of the liquid, g is the acceleration due to gravity, and r is the radius of the tube.
When the tube is pushed down so that the height of the tube outside the liquid is less than H, the liquid will still remain in the tube up to the height H unless the pressure conditions change.
As the tube is pushed down, the pressure at the bottom of the liquid column inside the tube will change. The pressure at a depth h in a liquid is given by:
P=P0+ρgh
where P0 is the atmospheric pressure.
If the height of the liquid column inside the tube is still H, the pressure at the bottom of the liquid column inside the tube must equal the pressure exerted by the liquid column plus the atmospheric pressure. If the tube is pushed down too far, the liquid may no longer be able to maintain the height H due to the external pressure being greater than the internal pressure.
If the tube is pushed down such that the height of the liquid column is less than H, the liquid will drop until a new equilibrium is reached where the pressure balance is maintained.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Higher Order Thinking Skills
8 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Exemplar Problems
5 Videos
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
15 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assertion And Reason
15 Videos
Similar Questions
Knowledge Check
Two capillary tubes of radii 0.2 cm and 0.4 cm are dipped in the same liquid.The ratio of heights through which liquid will rise in the tube is
A1:2B2:1C1:4D4:1
When a capillary tube is dipped in a liquid, it rises in the tube to a height h above the outside level . What would happen it the tube is dipped such that its length outside the liquid is less then h? Explain your answer briefly.
View SolutionThe end of a capillary tube is immersed into a liquid. Liquid slowly rises in the tube up to some height. The capillary-fluid system
View SolutionA capillary tube is dipped in water to a depth and the water rises to a height h(<l) in the capillary tube. The lower end of the tube is closed in water by putting a lower over it. The tube is now taken out and the thumb is removed from the lower end and it kept open. The length of liquid column in the tube will be
View SolutionThe liquid in the capillary tube will rise if the angle of contact is
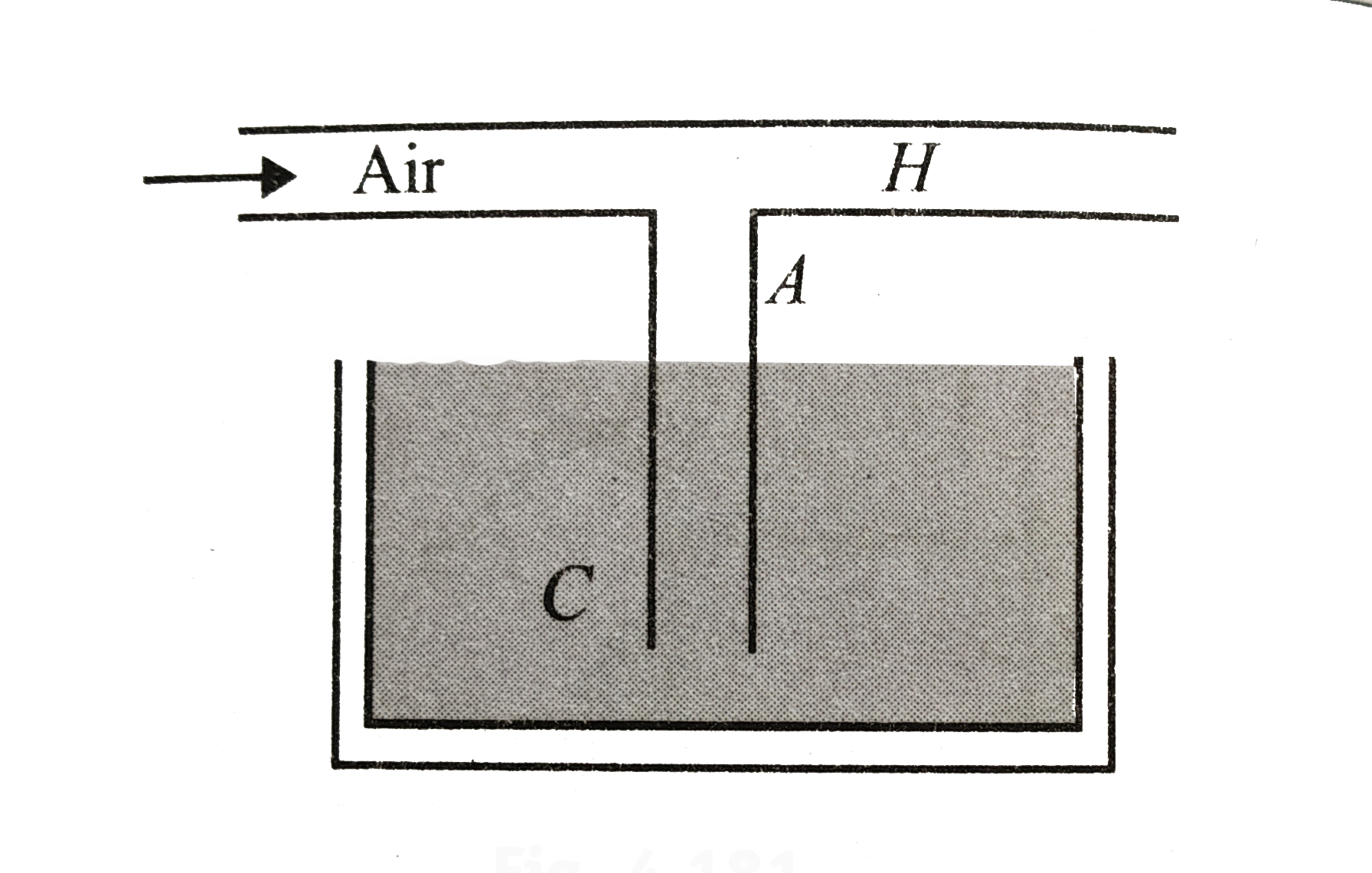
View SolutionFigure shows a capillary tube C dipped in a liquid that wets it. The liquid rises to a point A. If we blow air through the horizontal tube H, what will happen to the liquid column in the capillary tube?
View SolutionThe rise of a liquid in a capillary tube is due to :
View SolutionA capillary tube is dipped in water vertically. It is long enough for the water to rise to the maximum height h in the tube. The length of portion immersed in water is I<h. The lower end of the tube is closed and then the tube is taken out and opened again. Will all the water flow out of tube? Explain.
View Solution
