Assertion: The stress-strain behaviour varies from material to material.
Reason: A rubber can be pulled to several time its original length and still returns to its origin
shape.
Both assertion and reason are true and reason is the correct explanation of assertion.
Both assertion and reason are true but reason is not the correct explanation of assertion.
Assertion is true but reason is false.
Both assertion and reason are false.
The correct Answer is:B
To analyze the given question, we will break it down into two parts: the assertion and the reason.
Step 1: Understanding the Assertion
The assertion states that "The stress-strain behaviour varies from material to material."
- Explanation: This means that different materials respond differently when subjected to the same amount of stress. For example, metals, plastics, and rubber will all have unique stress-strain curves due to their distinct molecular structures and properties.
Step 2: Understanding the Reason
The reason provided is "A rubber can be pulled to several times its original length and still returns to its original shape."
- Explanation: This statement is true; rubber is an elastic material that can be stretched significantly and will return to its original shape once the stress is removed. However, this property of rubber does not explain why the stress-strain behavior varies across different materials.
Step 3: Conclusion
Now, we can conclude the relationship between the assertion and the reason:
- The assertion is true because different materials exhibit different stress-strain behaviors.
- The reason is also true, but it does not serve as a correct explanation for the assertion. The behavior of rubber is just one example of how materials can differ, but it does not encompass the full range of material behaviors.
Final Answer
Both the assertion and the reason are true, but the reason is not the correct explanation for the assertion.
The assertion states that "The stress-strain behaviour varies from material to material."
The reason provided is "A rubber can be pulled to several times its original length and still returns to its original shape."
Now, we can conclude the relationship between the assertion and the reason:
- The reason is also true, but it does not serve as a correct explanation for the assertion. The behavior of rubber is just one example of how materials can differ, but it does not encompass the full range of material behaviors.
Both the assertion and the reason are true, but the reason is not the correct explanation for the assertion.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Hooke'S Law
3 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Higher Order Thinking Skills
8 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - NCERT
8 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar
5 Videos
- MOTION IN A PLANE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A PLANE EXERCISE - Assertion And Reason
15 Videos
Similar Questions
Knowledge Check
The maximum load a wire can withstand without breaking, when its length is reduced to half of its original length, will
Abe doubleBbe halfCbe four timesDremain same.
Assertion: Above Curie temperature, a frerromagnetic material becomes paramagnetic.
Reason: When a magnetic material is heated to very high temperature, it loses its magnetic properties.
View SolutionAssertion Upto the elastic limit, strain ∝ stress.
Reason Upto elastic limit, material returns to its original shape and size, when external force is removed.
View SolutionFigure shows the relationship between tensile stress and strain for a typical material. Below proportional point A, stress is directly proportional to strain which means Young's moudulus (Y) is a constant. In this region the material obeys Hooke's law.

Provided the strain is below the yield point 'B' the material returns to its original shape and size when the force is removed. Beyond the yield point, the material retains a permancnt deformation after the stress is removed. For stresses beyond the yeld point, the material exhibit plastic flow, which means that it continues to elongate for little increases in the stress. Beyond C a local constriction occurs. The material fractures at D (i.e. breaking point). The graph below shows the stress-strain curve for 4 different materials.

If you bough a new shoe which bites in the beginning and later on fits perfectly, then the material used to making the shoe is
View SolutionFigure shows the relationship between tensile stress and strain for a typical material. Below proportional point A, stress is directly proportional to strain which means Young's moudulus (Y) is a constant. In this region the material obeys Hooke's law.

Provided the strain is below the yield point 'B' the material returns to its original shape and size when the force is removed. Beyond the yield point, the material retains a permancnt deformation after the stress is removed. For stresses beyond the yeld point, the material exhibit plastic flow, which means that it continues to elongate for little increases in the stress. Beyond C a local constriction occurs. The material fractures at D (i.e. breaking point). The graph below shows the stress-strain curve for 4 different materials.

If you bough a new shoe which bites in the beginning and later on fits perfectly, then the material used to making the shoe is
View SolutionFigure shows the relationship between tensile stress and strain for a typical material. Below proportional point A, stress is directly proportional to strain which means Young's moudulus (Y) is a constant. In this region the material obeys Hooke's law.

Provided the strain is below the yield point 'B' the material returns to its original shape and size when the force is removed. Beyond the yield point, the material retains a permancnt deformation after the stress is removed. For stresses beyond the yeld point, the material exhibit plastic flow, which means that it continues to elongate for little increases in the stress. Beyond C a local constriction occurs. The material fractures at D (i.e. breaking point). The graph below shows the stress-strain curve for 4 different materials.
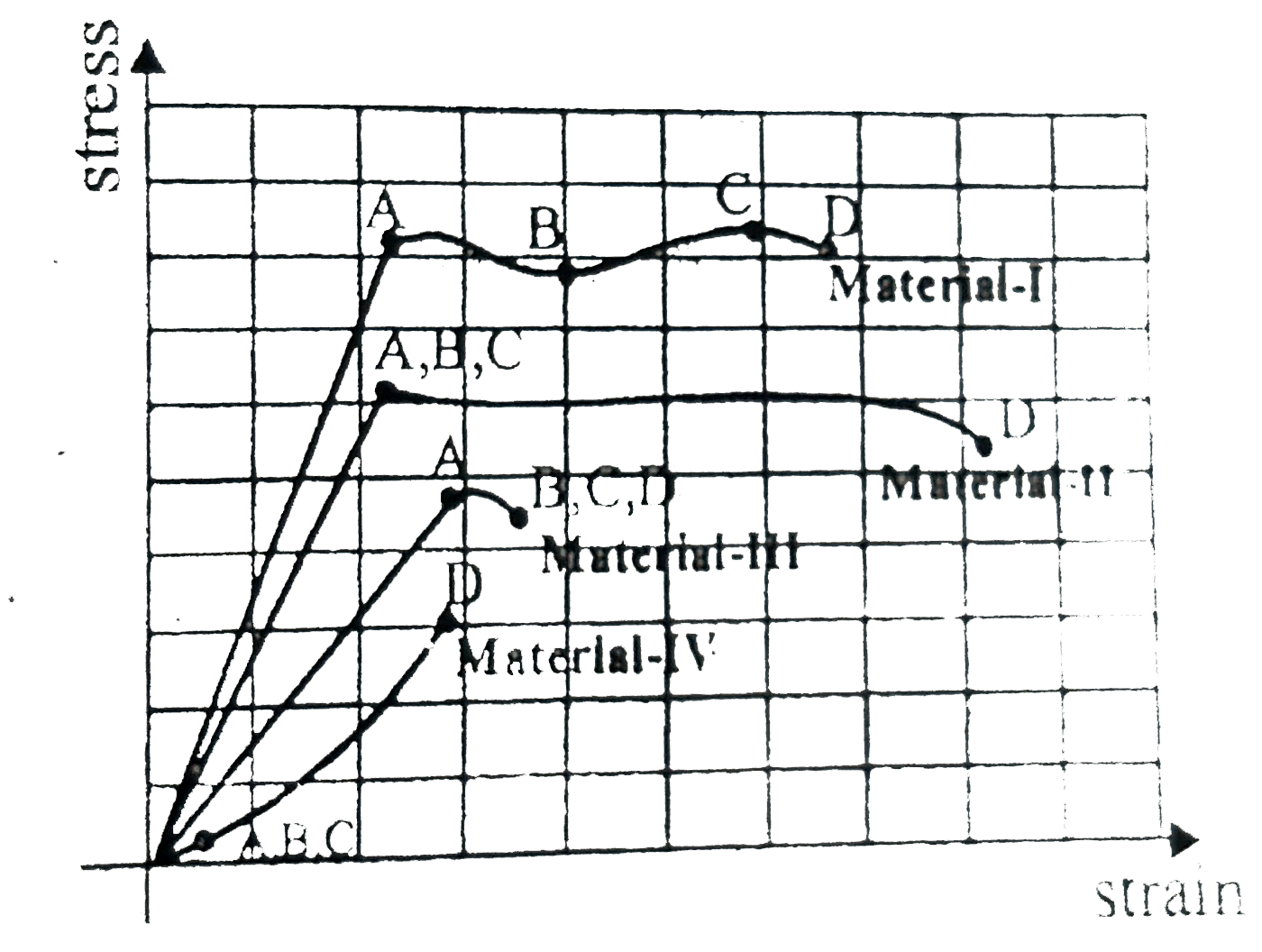
Material which is good for making wires by stretching is
View SolutionFigure shows the relationship between tensile stress and strain for a typical material. Below proportional point A, stress is directly proportional to strain which means Young's moudulus (Y) is a constant. In this region the material obeys Hooke's law.

Provided the strain is below the yield point 'B' the material returns to its original shape and size when the force is removed. Beyond the yield point, the material retains a permancnt deformation after the stress is removed. For stresses beyond the yeld point, the material exhibit plastic flow, which means that it continues to elongate for little increases in the stress. Beyond C a local constriction occurs. The material fractures at D (i.e. breaking point). The graph below shows the stress-strain curve for 4 different materials.

If you bough a new shoe which bites in the beginning and later on fits perfectly, then the material used to making the shoe is
View SolutionThe maximum load a wire can withstand without breaking, when its length is reduced to half of its original length, will
View Solution
