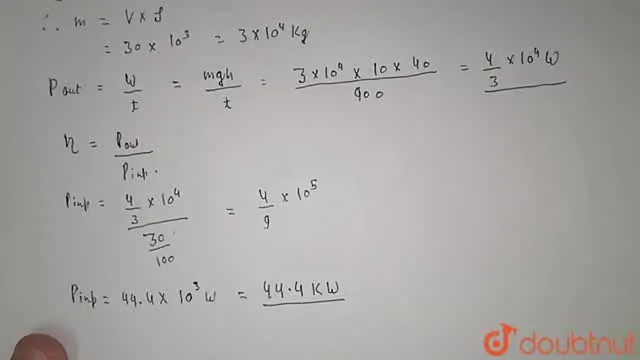A pump on the ground floor of a building can pump of water to fill a tank of volume 30ms3 in 15min. If the tank is 40m above the ground and the efficiency of the pump is 30% , how much electric power is consumed by the pump? (Takeg=10ms2)
36.5 kW
50 kW
52.5 kW
60.5 kW
The correct Answer is:B
Here, Volume of water = 30m3
t=15 min = 15 x 60 s = 900 s, Height, h = 60 m
Efficiency, η=40 %
Density of water = 103kgm−3
∴ Mass of water pumped = Volume x Density
=(30m3)(103kgm−3)=3×104kg
Poutput=Wt=mght=(3×104kg)(10ms−2)(60m)900s
=2×104W
Efficiency, η=PoutputPinput
Pinput=Poutputη=2×10440100=5×104
=50×103W=50kW
Related Playlists
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - WORK , ENERGY AND POWEREXERCISE - NCERT18videos
WORK , ENERGY AND POWER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - WORK , ENERGY AND POWEREXERCISE - ASSERTION & REASON15videos
WORK , ENERGY AND POWER
- BOOK - NCERT FINGERTPS ENGLISHCHAPTER - WAVESEXERCISE - Assertion And Reason15videos
WAVES
Similar Questions
A pump on the ground floor of a building can pump up water to fill a tank of volume 30m3 in 15 min. If the tank is 60 m above the ground, and the efficiency of the pump is 40%, how much electric power is consumed by the pump?
(Take g=10ms−2)View SolutionAn electric pump on the ground floor of a building taken 10 minute to fill a tank of 45m3 with water. The tank is 20m above the water level . If electric power consumed by the pump is 30kW then efficiency of the pump will be ( density of water is 1000kgm3)
View SolutionAn engine pumps up 100kg water through a height of 10m in 5s. If efficiency of the engine is 60%. What is the power of the engine? Takeg=10ms2.
View SolutionThe power of a motor pump is 2kW. How much water per minute the pump can raise to a heiht of 10 m ? (Given g=10m/s2)
View SolutionA ball is thrown vertically upwards from the ground and a student gazing out of the window sees it moving upward past him at 10ms−1. The window is at 15 m above the ground level. The velocity of ball 3 s after it was projected from the ground is [Take g=10ms−2]
View SolutionAn engine pumps 400 kg of water through height of 10 m in 40 s. Find the power of the engine if its efficiency is 80% (Take ,g =10 ms−2).
View SolutionAn engine pumps 400 kg of water through height of 10 m in 40 s. Find the power of the engine if its efficiency is 80% (Take ,g =10 ms−2).
View SolutionAn engine pumps 400 kg of water through height of 10 m in 40 s. Find the power of the engine if its efficiency is 80% (Take ,g =10 ms−2).
View SolutionAn engine pumps 800 kg of water through height of 10 m in 80 s. Find the power of the engine if its efficiency is 75%
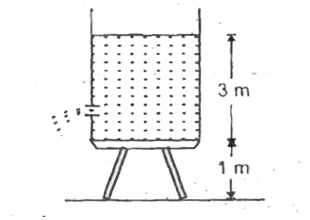
View SolutionWater is filled in a tank upto 3 m height. The base of the tank is at height 1 m above the ground. What should be the height of a hole made in it, so that water can be sprayed upto maximum horizontal distance on ground?
 View Solution
View Solution