माना और तब
किसी B का अस्तित्व इस प्रकार नहीं हो सकता है कि AB=BA
B's की एक से अधिक किन्तु परिमित संख्या का अस्तित्व इस प्रकार है कि AB=BA
ठीक एक B का अस्तित्व इस प्रकार है कि AB=BA
अनंत B's का अस्तित्व इस प्रकार है कि AB=BA
The correct Answer is:D
Step by step video, text & image solution for माना A=((1,2),(3,4)) और B=((a,0),(0,b)),a,binN तब by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- सारणिक तथा आव्यूह Book:ERRORLESS HINDIChapter:सारणिक तथा आव्यूह Exercise:EXERCISE
Explore 262 Videos
- सारणिक तथा आव्यूह Book:ERRORLESS HINDIChapter:सारणिक तथा आव्यूह Exercise:JEE ADVANCED MORE THAN ONE CORRECT ANSWERS
Explore 66 Videos
- सारणिक तथा आव्यूह Book:ERRORLESS HINDIChapter:सारणिक तथा आव्यूह Exercise:Solved Multiple Choice Questions ( रैखिक समीकरणों का निकाय कुछ विशेष सारणिक, सारणिक का अवकलन तथा समाकलन)
Explore 50 Videos
- सरल रेखा Book:ERRORLESS HINDIChapter:सरल रेखा Exercise:JEE ADVANCE (Matrix Match type questions)
Explore 4 Videos
Similar Questions
∣∣ ∣ ∣∣111cos(nx)cos(n+1)xcos(n+2)xsin(nx)sin(n+1)xsin(n+2)x∣∣ ∣ ∣∣ निर्भर नहीं करता है
View Solutionसारणिक ∣∣ ∣∣abaα+bbcbα+caα+bbα+c0∣∣ ∣∣=0 यदि
View Solutiona का वह मान जिसके लिये समीकरण निकाय a3x+(a+1)3y+(a+2)3z=0,ax(a+1)y+(a+2)z=0,x+y+z=0 का एक अशून्य हल है
View Solution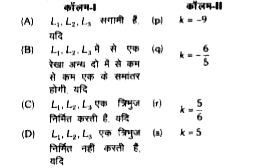
View SolutionIf A'=⎡⎢⎣34−1201⎤⎥⎦and B=[−121123], then verify that (A+B)′=A′+B′
View SolutionLet A=(1234) and B=(a00b), where a,b∈N, then
View SolutionLet A=[1234]andB=[a00b],a,b∈N Then,
View SolutionLet A=[1234]andB=[a00b] where a, b are natural numbers, then which one of the following is correct ?
View Solutionमाना A=[1234] तथा B=[a00b],a,b∈N हो तो-
View Solutionयदि A=[1234] तब [IfA=[1234] then]
View SolutionLet (1234) and B=(a00b),a,bεN. Then
View SolutionFind a matrix X such that 2A + B + X = 0 where A=[−1234],B=[3−215]
View Solutionयदि 2A+B=0, जहाँ 0 शून्य आव्यूह है तथा A=[−1234] तो आव्यूह B ज्ञात कीजिए।
View Solutionযদি A=[−1234] ও B=[3−215] এবং 2A+B+X=0 হয়, তবে X ম্যাট্রিক্স নির্ণয় করো |
View Solutionमाना 0<P(A)<1,0<P(B)<1 तथा P(A∪B)=P(A)+P(B)−P(A)P(B), तब
View Solution