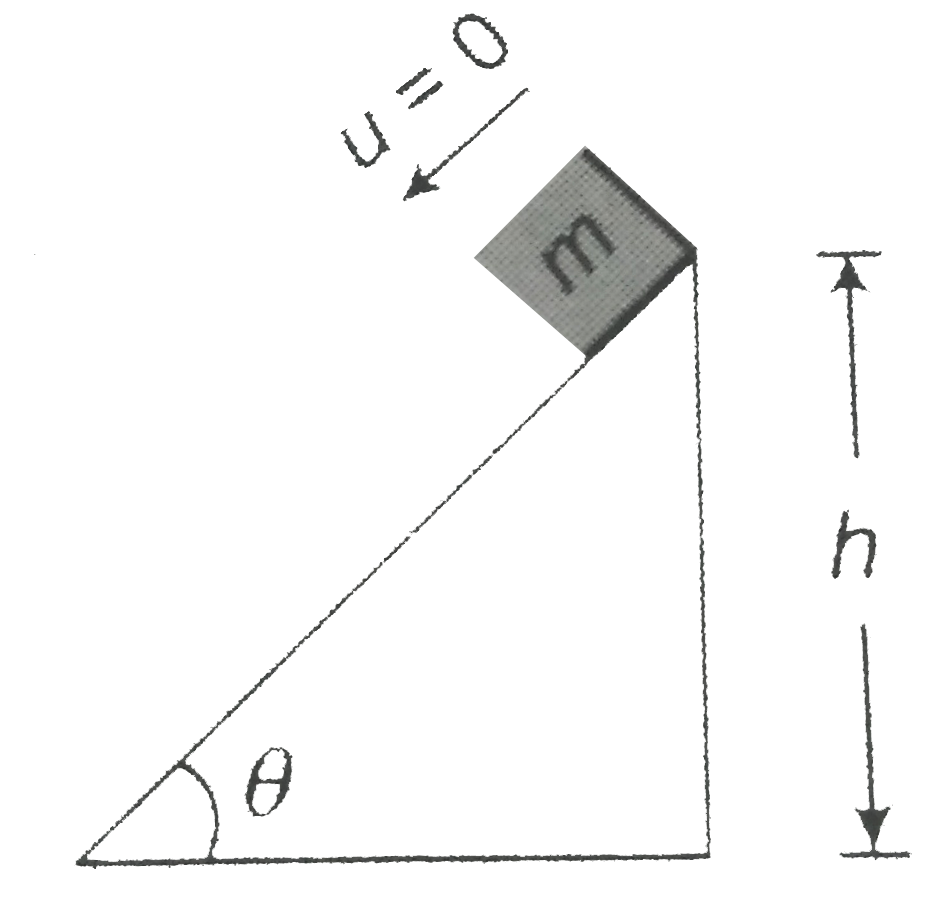
A block released from rest from the top of a smooth inclined plane of angle $θ_{1}$ reaches the bottom in time $t_{1}$ . The same block released from rest from the top of another smooth inclined plane of angle $θ_{2}$ reaches the bottom in time $t_{2}$ If the two inclined planes have the same height, the relation between $t_{1} and t_{2}$ is

$\frac{t_{2}}{t_{1}} = {(\frac{{sin θ}_{1}}{{sin θ}_{2}})}^{1 / 2}$

$\frac{t_{2}}{t_{1}} = 1$

$\frac{t_{2}}{t_{1}} = \frac{{sin θ}_{1}}{{sin θ}_{2}}$

$\frac{t_{2}}{t_{1}} = \frac{{sin}^{2} θ_{1}}{{sin}^{2} θ_{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

Lengths of the two inclined planes are
$l_{1} = \frac{h}{{sin θ}_{1}} and l_{2} = \frac{h}{{sin θ}_{2}}$
acceleration of the block down the two planes are
$a_{1} = g {sin θ}_{1} and a_{2} = g {sin θ}_{2}$
As $l_{1} = \frac{1}{2} a_{1} t_{1}^{2} and l_{2} = \frac{1}{2} a_{2} t_{2}^{2}$
$∴ \frac{l_{1}}{l_{2}} = \frac{a_{1} t_{1}^{2}}{a_{2} t_{2}^{2}} or \frac{t_{2}^{2}}{t_{1}^{2}} = \frac{a_{1} l_{2}}{a_{2} l_{1}} = \frac{g {sin θ}_{1}}{g {sin θ}_{2}} \times \frac{{sin θ}_{1}}{{sin θ}_{2}} = \frac{{sin}^{2} θ_{1}}{{sin}^{2} θ_{2}}$
p or $\frac{t_{2}}{t_{1}} = \frac{{sin θ}_{1}}{{sin θ}_{2}}$

Updated on:21/07/2023

Class 11 PHYSICS NEWTONS LAW OF MOTION

Topper's Solved these Questions

Knowledge Check

A block released from rest from the top of a smooth inclined plane of angle $θ_{1}$ reaches the bottom in $t_{1}$ .
The same block relased from rest from the top of another smooth inclined plane of angle $θ_{2}$ , reaches the bottom in time $t_{2}$ . If the two inclined planes have the same height, the relation between $t_{1} and t_{2}$ is
A $\frac{t_{2}}{t_{1}} = \frac{{sin θ}_{1}}{{({sin θ}_{2})}_{2}^{\frac{1}{2}}}$
B $\frac{t_{2}}{t_{1}} = 1$
C $\frac{t_{2}}{t_{1}} = \frac{{sin θ}_{1}}{{sin θ}_{2}}$
D $\frac{t_{2}}{t_{1}} = \frac{{sin}^{2} θ_{1}}{{sin}^{2} θ_{2}}$
A block released from rest from the top of a smooth inclined plane of angle $30^{\circ}$ reaches the bottom in time $t_{1}$ . The same block released from rest from the top of another smooth inclined plane of angle $60^{\circ}$ , reaches the bottom in time $t_{2}$ . If the two inclined planes have the same height, the relation between $t_{1}$ and $t_{2}$ is
A $\frac{t_{2}}{t_{1}} = \frac{1}{2}$
B $\frac{t_{2}}{t_{1}} = 1$
C $\frac{t_{2}}{t_{1}} = \frac{1}{\sqrt{3}}$
D $\frac{t_{2}}{t_{1}} = 2$
A block released from rest from the tope of a smooth inclined plane of angle of inclination $θ_{1} = 30^{\circ}$ reaches the bottom in time $t_{1}$ . The same block, released from rest from the top of another smooth inclined plane of angle of inclination $θ_{2} = 45^{\circ}$ reaches the bottom in time $t_{2}$ . Time $t_{1}$ and $t_{2}$ are related as ( Assume that the initial heights of blocks in the two cases are equal ).
A $\frac{t_{2}}{t_{1}} = \frac{1}{\sqrt{2}}$
B $\frac{t_{2}}{t_{1}} = \frac{1}{2}$
C $\frac{t_{2}}{t_{1}} = 1$
D $\frac{t_{1}}{t_{2}} = \frac{1}{2}$

MTG GUIDE-LAWS OF MOTION-NEET CAFÉ (TOPICWISE PRACTIVE QUESTIONS) ( NEWTON.S LAWS OF MOTION)

Home
Ask Doubt
Profile

The correct Answer is:C

Lengths of the two inclined planes are l1=hsinθ1andl2=hsinθ2 acceleration of the block down the two planes are a1=gsinθ1anda2=gsinθ2 As l1=12a1t21andl2=12a2t22 ∴l1l2=a1t21a2t22ort22t21=a1l2a2l1=gsinθ1gsinθ2×sinθ1sinθ2=sin2θ1sin2θ2 p or t2t1=sinθ1sinθ2

Topper's Solved these Questions

Similar Questions

Knowledge Check

MTG GUIDE-LAWS OF MOTION-NEET CAFÉ (TOPICWISE PRACTIVE QUESTIONS) ( NEWTON.S LAWS OF MOTION)