A horizontal force of 500 N pulls two masses 10 kg and 20 kg (lying on a frictionless table) connected by a light string. What is the tension in the string ? Does the answer depend on which mass end the pull is applied?

Here, m1=10kg,m2=20kgandF=500N Case (a) : When the force F is applied on mass m1: Let T1 be the tension in the connecting string. The free body diagram with all the forces in the horizontal directions of the masses m1andm2 are shown in figure (a). Now, applying Newton.s second law of motion to masses m, and m, we get
F−T1=m1a
andT1=m2a
Eliminating a from eqns (i) and (ii) we get,
F−T1m1=T1m2orT1=m2Fm1+m2=20×50010+20=333.3N
Case (b) : When the force is applied on mass my. With reference to figure (b), we can write,
T2=m1a
and F−T2=m2a
Again eliminating a from eqns (iii) and (iv) we get,
T2=m1Fm1+m2=10×50010+20=166.7N.
So, the tensions in both the cases are different, i.e., it depends on which mass the force is applied. It may be noted that the acceleration is same in both the cases.
Topper's Solved these Questions
- BOOK - MTG GUIDECHAPTER - LAWS OF MOTIONEXERCISE - NEET CAFÉ (TOPICWISE PRACTIVE QUESTIONS) ( NEWTON.S LAWS OF MOTION)81videos
LAWS OF MOTION
- BOOK - MTG GUIDECHAPTER - LAWS OF MOTIONEXERCISE - NEET CAFÉ (TOPICWISE PRACTIVE QUESTIONS) (LAW OF CONSERVATION OF LINEAR MOMENTUM AND ITS APPLICATIONS)6videos
LAWS OF MOTION
- BOOK - MTG GUIDECHAPTER - KINEMATICS EXERCISE - AIPMT/ NEET MCQs31videos
KINEMATICS
- BOOK - MTG GUIDECHAPTER - MOTION OF SYSTEM OF PARTICLES AND RIGID BODY EXERCISE - AIPMT / NEET (MCQs)37videos
MOTION OF SYSTEM OF PARTICLES AND RIGID BODY
Similar Questions
Two block of masses m1andm2 are lying on a frictionless horizontal table connected by a light string m2 is pulled by horizontal force F. Calculate the tension in the string .
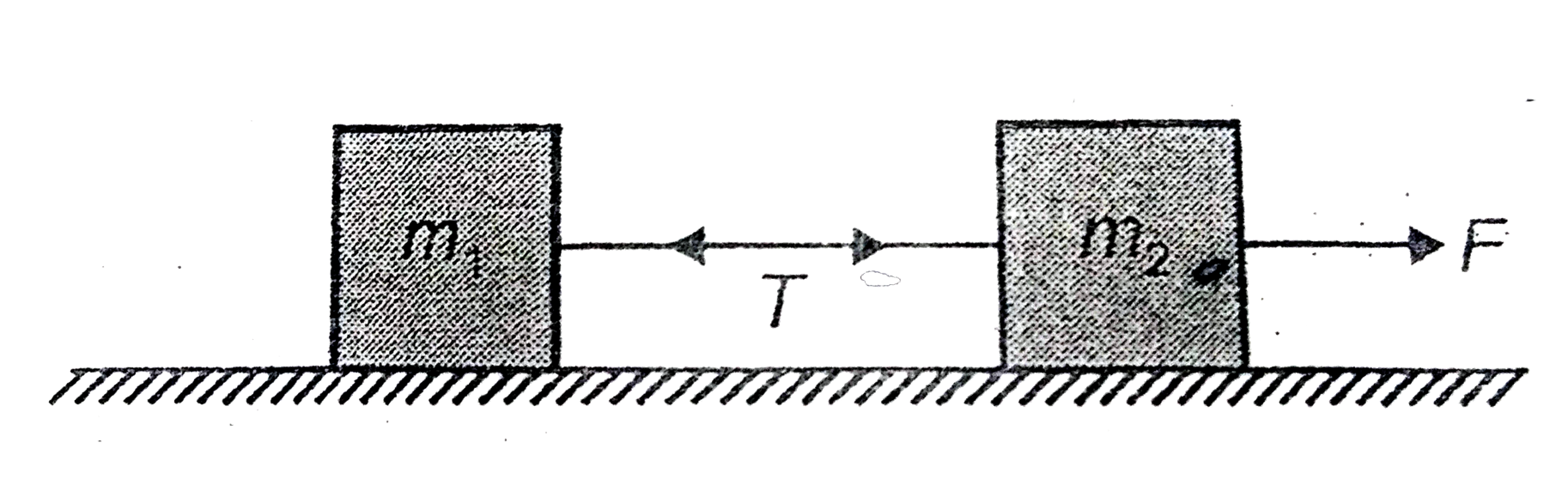 View Solution
View SolutionTwo blocks each of mass m are connected over two light and frictionless pulleys and a fixed wedge by a light string as shown in figure. The tension in the string is :
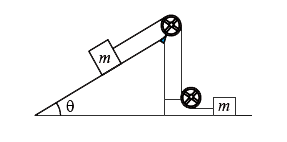 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A string of length L and mass M is lying on a horizontal table. A force F is applied at one of its ends. Tension in the string at a distance x from the end at which force is applied is
AZeroBF should be equal to the weight of A and BCF(L-x)/LDF(L-x)M - Question 2 - Select One
A string of length L and mass M is lying on a horizontal table. A force F is applied at one of its ends. Tension in the string at a distance x from the end at which force is applied is
AZeroBFCF(L−y)LDF(l−y)M - Question 3 - Select One
Two blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 A188 NB2=368 NC288 ND168 N
A188 NB2=368 NC288 ND168 N
Two masses of 10 kg and 20 kg are connected with an inextensible string passing over two smooth pulleys as shown in the figure. The acceleration of 20 kg mass will be :
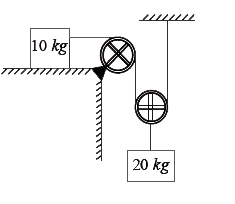 View Solution
View SolutionThree metal blocks A , B and C of masses 10 kg , 20 kg and 30 kg rest on a frictionless table in a line . A is converted to B and B to C by two pieces of string . With the help of a third string attached to C , the system is pulled to the right with a force of 60 N . Find the tensions of the first and second strings .
View SolutionTwo blocks of masses of 40 kg and 30 kg are connected by a weightless string passing over a frictionless pulley as shown in the figure.
 View Solution
View SolutionTwo blocks of masses m and M are placed on a horizontal frictionless table connected by light spring as shown in the figure. Mass M is pulled to the right with a force F. If the acceleration of mass m is a, then the acceleration of mass M will be
 View Solution
View SolutionTwo blocks A and B each of 20 kg lying on a frictionless table are connected by a light string. The system is pulled horizontally with an acceleration of 2ms−2 by a force F on B. The tension in the string will be
View Solution
