If pressure P, velocity of light C and acceleration due to gravity g are chosen as fundamental units, then dimensional formula of mass is Solution in Telugu
Pc3g−4
Pc−4g3
Pc4g−3
Pc4g3
The correct Answer is:C
Step by step video, text & image solution for If pressure P, velocity of light C and acceleration due to gravity g are chosen as fundamental units, then dimensional formula of mass is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- RIVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - UNITS AND MEASUREMNET (PRINCIPLE OF HOMOGENEITY)
32 Videos
- RIVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - UNITS AND MEASUREMNET (MEASUREMENT OF ERRORS)
66 Videos
- RIVISION EXERCISEBOOK - AAKASH SERIESCHAPTER - RIVISION EXERCISEEXERCISE - MOTION IN A PLANE (LAMNI.S THEOREM)
6 Videos
- RAY OPTICS BOOK - AAKASH SERIESCHAPTER - RAY OPTICS EXERCISE - EXERCISE III
64 Videos
- ROTATIONAL DYNAMICS BOOK - AAKASH SERIESCHAPTER - ROTATIONAL DYNAMICS EXERCISE - PRACTICE SHEET (ADVANCED) Integer Type Questions
7 Videos
Similar Questions
A force F is given by F at + bt2 where t is time. What are dimensions of 'a' and 'b'?
View SolutionMeasurement of errors 35. The length of a rectangular plate is measured as 10 cm by a vernier scale of least count 0.01 cm and its breadth as 5 cm by the same scale. The percentage error in area is
View SolutionIf a vector →A makes angles 45∘and60∘ with x and y axis respectively then the angle made by it with z-axis is
View SolutionTwo plane mirrors are arranged at right angles to each other as shown in figure. A ray of light is incident on the horizontal mirror at an angle θ For what value of θ the ray emerges parallel to the incoming ray after reflection from the vertical mirror?
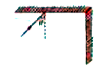
View SolutionFour uniform solid spheres each of diameter √5 cm and mass 0.5 kg are placed with their centres at the corners of a square of side 4 cm. The moment of inertia of the system about the diagonal of the square is N×10−4kg m2, then the value of N is .
View SolutionIf the speed of light c, acceleration due to gravity (g) and pressure (p) are taken as the fundamental quantities then the dimension of gravitational constant is
View SolutionIf velocity of light (c ), gravitational constant (G) and Planck's constant (h) are chosen as fundamental units. What would be the dimensions of acceleration due to gravity ?
View SolutionIf the velocity of light denoted by 'c', acceleration due to gravity 'g' and atmospheric pressure 'p' are taken as fundamental units, then the dimensional formula of length will be :
View SolutionIf C, the velocity of light, g the acceleration due to gravity and P the atmospheric pressure in M.K.S units are the fundamental units then the dimension of length will be :
View SolutionThe speed of light c , acceleration due to gravity g and pressure p are taken as fundamental units, the dimensions of gravitational constant G are :
View SolutionIf speed of light (c), acceleration due to gravity (g) and pressure (P) are taken as fundamental units, the possible relation to gravitational constant (G) is..............
View SolutionIf pressure P, velocity of light C and acceleration due to gravity g are chosen as fundamental units, then dimensional formula of mass is
View SolutionIf speed of light (c), acceleration due to gravity (g) and pressure (P) are taken as fundamental units, the dimensions of gravitational constant (G) are
View SolutionIf the velocity of light, acceleration due to gravity and normal pressure are chosen as fundamental units, find the unit of mass, length and time. Given that velocity of light c=3×108ms−1g=10ms−2 and the normal atmospheric pressure P=105Nm−2
View SolutionIf pressure P, velocity of light C and acceleration due to gravity g are chosen as fundamental units, then dimensional formula of mass is
View SolutionIf pressure P, velocity of light C and acceleration due to gravity g are chosen as fundamental units, then dimensional formula of mass is
View Solution
