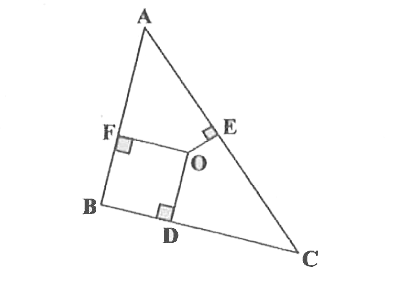
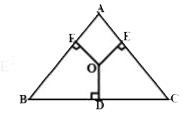
In the given figure, O is a point in the interior of a DeltaABC, OD⊥BC,OE⊥AC and OF⊥AB. Show that :
AF2+BD2+CE2=AE2+CD2+BF2.
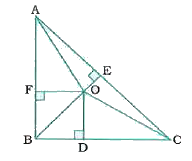 Solution in Gujrati
Solution in Gujrati
The correct Answer is:AF2+BD2+CE2=AE2+CD2+BF2
Step by step video, text & image solution for In the given figure, O is a point in the interior of a DeltaABC, OD bot BC, OE bot AC" and "OF bot AB. Show that : AF^(2)+BD^(2)+CE^(2)=AE^(2)+CD^(2)+BF^(2). by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.6
15 Videos
- TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - TEST YOUR SKILLS
29 Videos
- TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.4
9 Videos
- SURFACE AREAS AND VOLUMESBOOK - KUMAR PRAKASHANCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - TEST YOUR SKILLS
5 Videos
Similar Questions
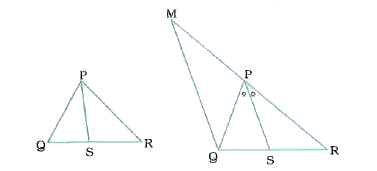
In the given figure, PS in the bisector of ∠QPR of ΔPQR. Prove that QSSR=PQPR.
View SolutionIn the given figure, DE||BC and PE||DC. If AP= 4 cm and PB= 12 cm, find AD.
View SolutionLet ΔABC~ΔDEF and their areas be, respectively, 64cm2 and 121cm2. If EF=15.4cm, find BC.
View SolutionThe slant height of a frustum of a cone is 4 m and the perimeter of circular ends are 18 m and 16 m. Find the cost of painting its curved surface at 10 per sq. m.
View Solutionआकृति 6 . 54 में ΔABC के अभ्यन्तर में स्थित कोई बिंदु O है तथा OD⊥BC,OE⊥AC और OF⊥AB है दर्शाइए कि
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AE2+CD2+BF2

View SolutionO is a point in the interior of ΔABC,OD⊥BC,OE⊥ACandOF⊥AB, as shown in the figure,
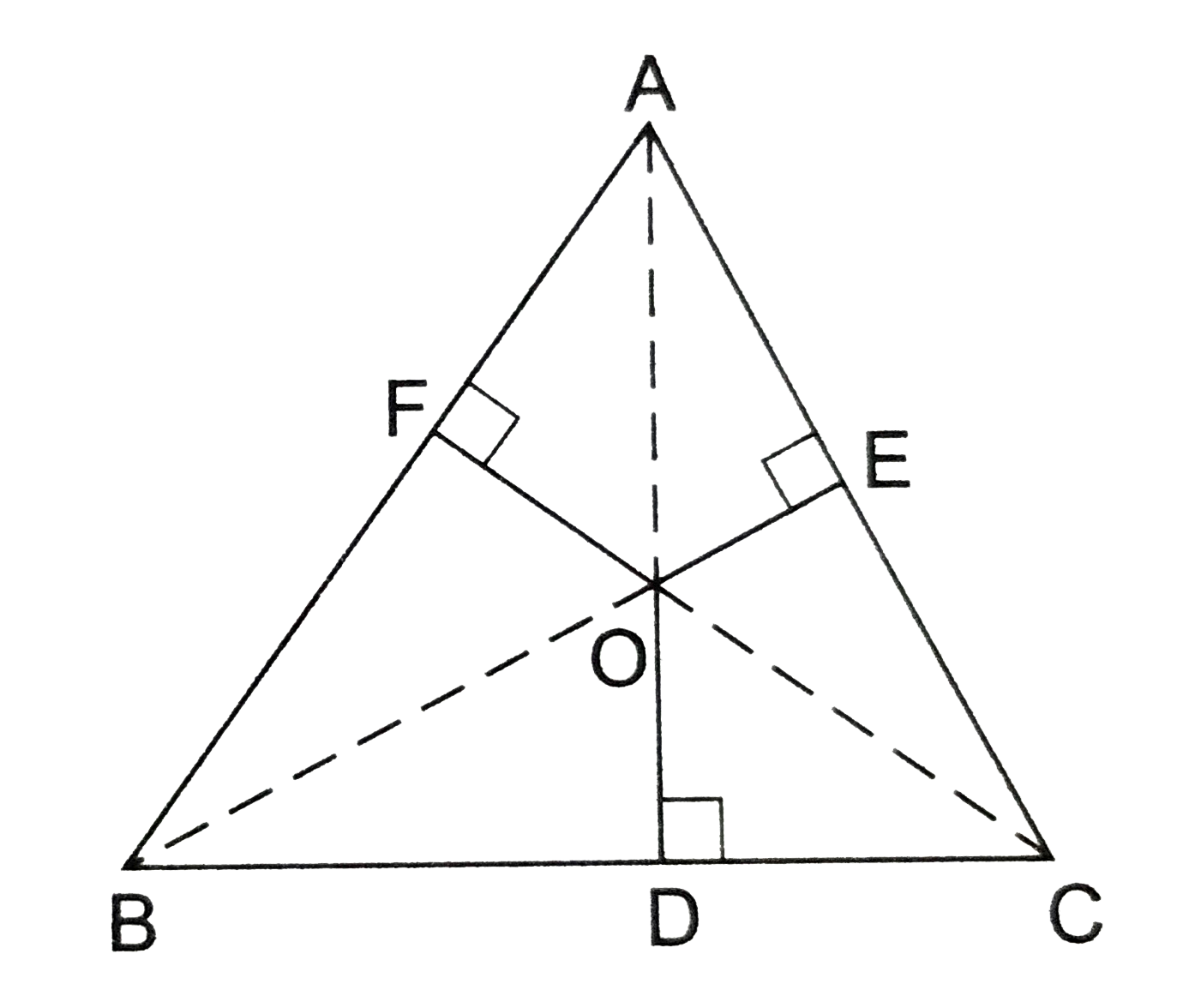
Prove that :
(i)OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AD2+BF2+CD2
View SolutionΔABC के अंदर बिंदु O से भुजाओ BC,CA और AB पर क्रमशः लंब OD,OE और OF डाले गए है । सिद्ध कीजिए कि AF2+BD2+CE2=AE2+CD2+BF2.
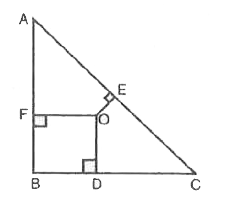
View SolutionIn fig . O is a point in the interior of a triangle ABC , OD ⊥BC,OE⊥ACandOF⊥AB . Show that
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2+AE2+CD2+BF2 .
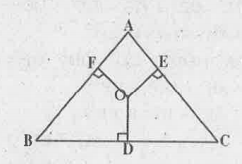
View Solutionआकृति में , ΔABC के अभ्यंतर में स्थित कोई बिंदु O है तथा OD⊥BC , OE⊥AC और OF⊥AB है । दर्शाइए कि
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AE2+CD2+BF2 .
View Solutionత్రిభుజము ABC అంతరంలో ఏదైనా బిందువు 'O' OD⊥BC,OE⊥AC మరియు OF⊥AB అయిన AE2+BF2+CD2=AF2+BD2+CE2 అని చూపండి.
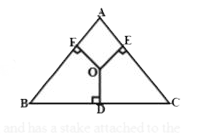
View Solution‘O’ is any point in the interior of a triangle ABC. If OD⊥BC,OE⊥AC and OF ⊥ AB, show that
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AE2+CD2+BE2.
View Solution‘O’ is any point in the interior of a triangle ABC. If OD⊥BC,OE⊥AC and OF ⊥ AB, show that
(i) OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AE2+CD2+BE2.
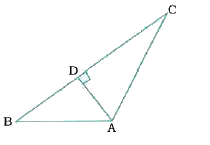
View SolutionIn the given figure, if AD⊥BC, prove that AB2+CD2=BD2+AC2
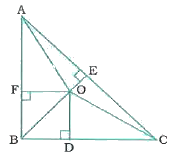
View SolutionIn the given figure, O is a point in the interior of a DeltaABC, OD⊥BC,OE⊥AC and OF⊥AB. Show that :
OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2.
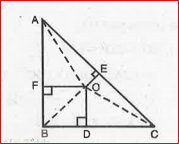
View SolutionIn fig., O is a point in the interior of a triangle ABC, OD ⊥ BC, OE ⊥ AC and OF ⊥ AB. Show that:- OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2 .
View Solution
