Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.Solution in Gujrati
The correct Answer is:ar(ABC)ar(PQR)=(ADPM)2
Step by step video, text & image solution for Prove that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.5
23 Videos
- TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.6
15 Videos
- TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.3
27 Videos
- SURFACE AREAS AND VOLUMESBOOK - KUMAR PRAKASHANCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - TEST YOUR SKILLS
5 Videos
Similar Questions
Sides of triangles are given below, Determine which of them are right triangles. In case of a right triangle, write the length of its hypotenuse :
7 cm, 24 cm, 25 cm
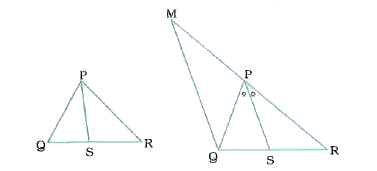
View SolutionIn the given figure, PS in the bisector of ∠QPR of ΔPQR. Prove that QSSR=PQPR.
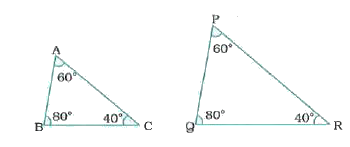
View SolutionState which pairs of triangles in the given figure are similar. Write the similarity criterion used by you for answering the question and also write the pairs of similar triangles in the symbolic form :
View SolutionThe slant height of a frustum of a cone is 4 m and the perimeter of circular ends are 18 m and 16 m. Find the cost of painting its curved surface at 10 per sq. m.
View SolutionThe areas of the two similar triangles are in the ratio of the square of the corresponding medians.
View Solutionसिद्ध कीजिए कि दो समरूप त्रिभुजों के क्षेत्रफलों का अनुपात इनकी संगत माध्यिकाओ के अनुपात का वर्ग होता है
View Solutionसिद्ध कीजिए कि दो समरूप त्रिभुजों के क्षेत्रफलों का अनुपात इनकी संगत माध्यिकाओं के अनुपात का वर्ग होता है ।
View SolutionProve that the ratio of areas of two similar triangle is equal to the square of the ratio of their corresponding medians.
View SolutionThe ratio of areas of two similar triangles is equal to the ratio of the squares of corresponding…….
View SolutionProve that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View SolutionProve that the ratio of areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View Solutionprove that The areas of the two similar triangles are in the ratio of the square of the corresponding medians.
View SolutionProve that the ratio at the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View SolutionProve that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View SolutionProve that the ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding medians.
View Solution
