From a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2.Solution in Gujrati
The correct Answer is:The total expenses of polishes and painting = Rs. 6275
Step by step video, text & image solution for From a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm^(2). by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SURFACE AREAS AND VOLUMESBOOK - KUMAR PRAKASHANCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - SKILL TESTING EXERCISE
49 Videos
- SURFACE AREAS AND VOLUMESBOOK - KUMAR PRAKASHANCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - MULTIPLE CHOICE QUESTIONS
18 Videos
- SURFACE AREAS AND VOLUMESBOOK - KUMAR PRAKASHANCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - EXERCISE 13.8
10 Videos
- STATISTICSBOOK - KUMAR PRAKASHANCHAPTER - STATISTICSEXERCISE - MULTIPLE CHOICE QUESTIONS
12 Videos
- TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - SUMS TO ENRICH REMEMBER
9 Videos
Similar Questions
The length, breadth and height of a cuboidal box are 15 cm, 12 cm and 8 cm respectively. Find its total surface area.
View SolutionThe total surface area of a cuboid with length 20 cm, breadth 15 cm and height 10 cm is ……… cm2.
View SolutionFind the volume of a sphere whose radius is (i) 7 cm (ii) 0.63 cm.
View SolutionThe marks scored by Kavya in 10 tests of Mathematics are 35. 18, 41, 24, 45, 10, 28, 32, 40, 15. Then, the range of the data is.......
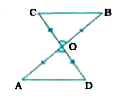
View SolutionIn the given figures, OA = OB and OD = OC. Show that
(i) △AOD=△BOC and (ii) AD||BC
View SolutionFrom a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2
View SolutionFrom a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2 .
View SolutionFrom a solid cylinder of height 2.8 cm and diameter 4.2 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid. (Take π=22/7 )
View SolutionFrom a solid cylinder whose height is 15 cm and diameter 16 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid. [Useπ=3.14.]
View Solutionऊँचाई 2⋅4 सेमी और व्यास 1⋅4 सेमी वाले एक ठोस बेलन में से इसी ऊँचाई और इसी व्यास वाला एक शंक्वाकार खोल काट लिया जाता है | शेष बचे ठोस का निकटतम वर्ग सेन्टीमीटर तक पृष्ठीय क्षेत्रफल ज्ञात कीजिए |
View Solutionएक 24 सेमी. ऊँचाई तथा 1.4 सेमी, व्यास वाले ठोस बेलन में से समान ऊँचाई और समान व्यास वाला एक शंकु के कार का खोल (cavity) काट लिया जाता है। शेष बचे हुए ठोस का पृष्टीय क्षेत्रफल निकटतम वर्ग सेमी, तक ज्ञात करें।
View SolutionFrom a solid cylinder whose height is 2.4 cm and the diameter 1.4 cm, a cone of the same height and same diameter is carved out. Find the volume of the remaining solid to the nearest cm3.
View SolutionFrom a solid cylinder whose height is 2.4cm and diameter 1.4cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2.
View SolutionFrom a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2
View SolutionFrom a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2 .
View SolutionFrom a solid cylinder of height 2.8 cm and diameter 4.2 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid. (Take π=22/7 )
View Solution
