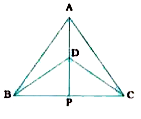
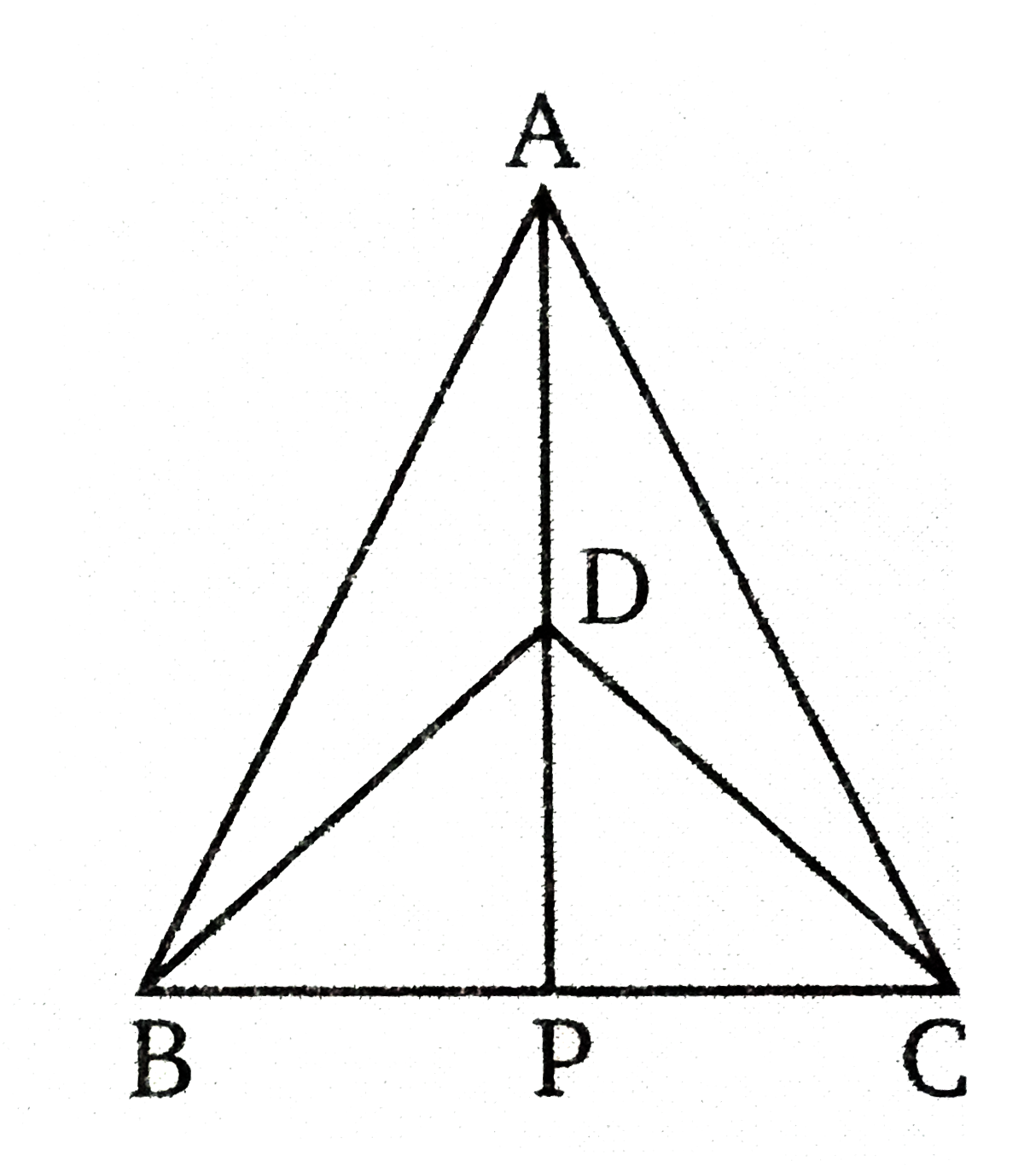
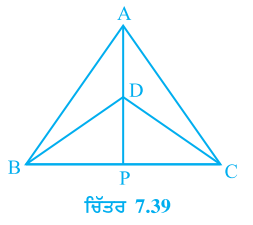
△ ABC and △ DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see the given figure). If AD is extended to intersect BC at P, show that, ( i ) △ABD=△ACD(ii)△ABP=△ACP (iii) AP bisects ∠ A as well as ∠ D. (iv) AP is the perpendicular bisector of BC
 Solution in Gujrati
Solution in Gujrati
The correct Answer is:CP
Step by step video, text & image solution for triangle ABC and triangle DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see the given figure). If AD is extended to intersect BC at P, show that, ( i ) triangle ABD = triangle ACD (ii) triangle ABP = triangle ACP (iii) AP bisects angle A as well as angle D. (iv) AP is the perpendicular bisector of BC by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - EXERCISE 7.4
6 Videos
- TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - EXERCISE 7.5
4 Videos
- TRIANGLESBOOK - KUMAR PRAKASHANCHAPTER - TRIANGLESEXERCISE - EXERCISE 7.2
8 Videos
- SURFACE AREAS AND VOLUMESBOOK - KUMAR PRAKASHANCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - MULTIPLE CHOICE QUESTIONS
18 Videos
Similar Questions
Show that in a right angled triangle, the hypotenuse is the longest side.
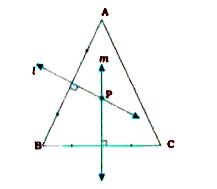
View SolutionABC is a triangle. Locate a point in the interior of △ ABC which is equidistant from all the vertices of △ ABC
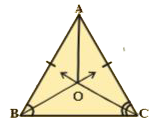
View SolutionIn an isosceles triangle ABC, with AB = AC, the bisectors of ∠Band∠C intersect each other at O. Join A to O. Show that :
(i) OB = OC (ii) AO bisects ∠A
View SolutionThe total surface area of a cuboid with length 20 cm, breadth 15 cm and height 10 cm is ……… cm2.
View SolutionDelta ABC and Delta DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at E show that
View SolutionΔABCandΔDBC are on same base BC and their vertices A and D are on opposite sides of BC. It is given that:
area of ΔABC= area of ΔDBC
Prove that BC bisects the line segment AD.
View SolutionΔABC और ΔDBC एक ही आधार BC पर बने दो समद्विबाहु त्रिभुज इस प्रकार हैं कि A और D भुजा BC के एक ही ओर स्थित हैं। यदि AD बढ़ाने पर BC को P पर प्रतिच्छेद करे, तो दर्शाइए कि
(i) ΔABD≅ΔACD
(ii) ΔABP≅ΔACP
(iii) AP कोण A और कोण D दोनों को समद्विभाजित करता है।
(iv) AP रेखाखंड BC का लम्ब समद्विभाजक है।

View Solutionसमान आधार BC पर दो समद्विबाहु त्रिभुज ABC तथा DBC है तथा BC की समान भुजा पर शीर्ष A और Dहै यदि AD को बढ़ाने पर ,BC को P पर प्रतिच्छेद करती है ।
(i) ΔABD≅ΔACD
(ii) ΔABP≅ΔACP
(iii) AP,∠A व ∠Dको समद्विभाजित करते है ।
(iv) AP, BC का लम्बर्द्धक है ।
View Solution△ ABC and △ DBC are isosceles triangles on the same base BC. Prove that line AD bisects BC at right angles
View SolutionABC and DBC are two isosceles triangles on the same base BC (see the given figure). Show that ∠ABD=∠ACD.
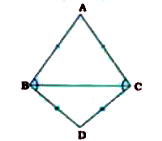
View Solution△ ABC and △ DBC are isosceles triangles on the same base BC. Prove that line AD bisects BC at right angles
View SolutionΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (See Fig.
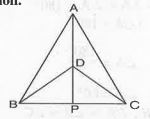 ).If AD is extended to intersect BC at P, show that AP bisects ∠A as well as ∠D.
).If AD is extended to intersect BC at P, show that AP bisects ∠A as well as ∠D.
View Solution△ABC ਅਤੇ △DBC ਇੱਕ ਹੀ ਆਧਾਰ BC ਉਤੇ ਦੋ ਸਮਦੋਭੁਜੀ ਤ੍ਰਿਭੁਜਾਂ ਹਨ ਅਤੇ ਸਿਖਰ A ਅਤੇ D ਭੁਜਾ BC ਦੇ ਇਕੋ ਪਾਸੇ ਹਨ (ਵੇਖੋ ਚਿੱਤਰ 7.39)। ਜੇ ਭੁਜਾ AD ਨੂੰ ਇਸ ਤਰ੍ਹਾਂ ਵਧਾਇਆ ਜਾਵੇ ਕਿ ਭੁਜਾ BC ਨੂੰ P 'ਤੇ ਕੱਟੇ ਤਾਂ ਸਿੱਧ ਕਰੋ ਕਿ △ABD≅△ACD
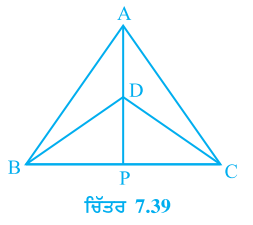
View Solution△ABC ਅਤੇ △DBC ਇੱਕ ਹੀ ਆਧਾਰ BC ਉਤੇ ਦੋ ਸਮਦੋਭੁਜੀ ਤ੍ਰਿਭੁਜਾਂ ਹਨ ਅਤੇ ਸਿਖਰ A ਅਤੇ D ਭੁਜਾ BC ਦੇ ਇਕੋ ਪਾਸੇ ਹਨ (ਵੇਖੋ ਚਿੱਤਰ 7.39)। ਜੇ ਭੁਜਾ AD ਨੂੰ ਇਸ ਤਰ੍ਹਾਂ ਵਧਾਇਆ ਜਾਵੇ ਕਿ ਭੁਜਾ BC ਨੂੰ P 'ਤੇ ਕੱਟੇ ਤਾਂ ਸਿੱਧ ਕਰੋ ਕਿ△ABP≅△ACP
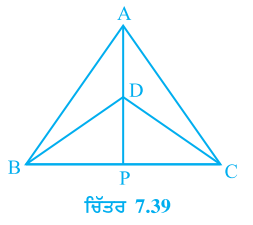
View Solution△ABC ਅਤੇ △DBC ਇੱਕ ਹੀ ਆਧਾਰ BC ਉਤੇ ਦੋ ਸਮਦੋਭੁਜੀ ਤ੍ਰਿਭੁਜਾਂ ਹਨ ਅਤੇ ਸਿਖਰ A ਅਤੇ D ਭੁਜਾ BC ਦੇ ਇਕੋ ਪਾਸੇ ਹਨ (ਵੇਖੋ ਚਿੱਤਰ 7.39)। ਜੇ ਭੁਜਾ AD ਨੂੰ ਇਸ ਤਰ੍ਹਾਂ ਵਧਾਇਆ ਜਾਵੇ ਕਿ ਭੁਜਾ BC ਨੂੰ P 'ਤੇ ਕੱਟੇ ਤਾਂ ਸਿੱਧ ਕਰੋ ਕਿ AP, ∠A ਅਤੇ ∠D ਨੂੰ ਸਮਦੁਭਾਜਿਤ ਕਰਦੀ ਹੈ।
View Solution
