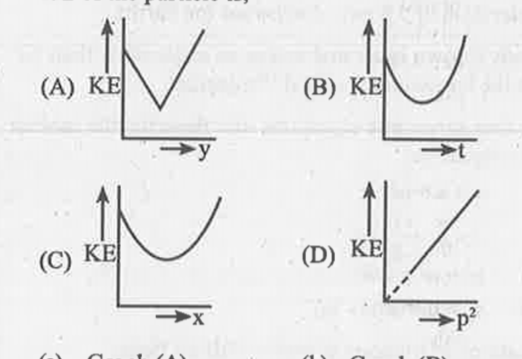
A partical is projected up from a point at an angle theta whith the horizontal displacement, the graph among the following which does not represent the variation of kinrtic energy KE of the particle is,
 Solution in Telugu
Solution in Telugu
Graph (A)
Graph (B)
Graph (C )
Graph (D)
The correct Answer is:A
Step by step video, text & image solution for A partical is projected up from a point at an angle theta whith the horizontal displacement, the graph among the following which does not represent the variation of kinrtic energy KE of the particle is, by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WORK, POWER & ENERGY BOOK - AAKASH SERIESCHAPTER - WORK, POWER & ENERGY EXERCISE - EXERCISE - II (POWER)
17 Videos
- WORK, POWER & ENERGY BOOK - AAKASH SERIESCHAPTER - WORK, POWER & ENERGY EXERCISE - EXERCISE - II (VERTICAL CIRCLE)
16 Videos
- WORK, POWER & ENERGY BOOK - AAKASH SERIESCHAPTER - WORK, POWER & ENERGY EXERCISE - EXERCISE - II (WORK)
20 Videos
- WORK POWER ENERGYBOOK - AAKASH SERIESCHAPTER - WORK POWER ENERGYEXERCISE - EXERCISE - 3
61 Videos
Similar Questions
A bullet loses 25% of its initial kinetic energy after penetrating through a distance of 2cm in a target. The further distance it travels before coming to rest is
View SolutionA mass of 0.1 kg is rotated in a vertical circle using a string of legth 20 cm. When the string makes an angle 30∘ with the vertical, the speed of the mass is 1.5ms−1. The tangential acceleration of the mass at that instant is
View SolutionThe length of a smooth inclined plane of incilination 30∘ is 5 m. The work done is moving a 10kg mass from the bottom of the inclined plane to top is
View SolutionIf →A+→B=→Rand2→A+→B is perpendicular to →B then
View SolutionA particle is projected from a point at an angle with the horizontal at t=0 . At an instant t, if p is linear momentum, x is horizontal displacement, y is vertical displacement, and E is kinetic energy of the particle, then which of the following graphs are correct?
View SolutionA particle is projected with velocity v at an angle of θ with horizontal. The average angular velocity of the particle from the point of projection to impact equals:
View SolutionA ball is thrown up with a certain velocity at an angle theta to the horizontal. The graph between kinetic energy and horizontal displacement is given by:
View SolutionA particle is projected from a point of an angle with the horizontal. At any instant t, if p is the linear momentum and E the kinetic energy, then which of the following graph is/are correct?
View SolutionA particle is projected up from a point at an angle of 30∘ with the horizontal. At any time tif p= linear momentum y= vertical displacement x= horizontal displacement then the kinetic energy (K) of the particle plotted against these parametes can be
View SolutionThe velocity -displacement graph of a particle is as shown in the figure. Which of the following graphs correctly represents the variation of acceleration with displacement?
View SolutionA partical is projected up from a point at an angle theta whith the horizontal displacement, the graph among the following which does not represent the variation of kinrtic energy KE of the particle is,
View SolutionA particle falls from rest under the action of gravity . Variation of kinetic energy (KE) and potential energy (PE) of the particle , with time t is represented by the graph
View SolutionA particle is projected at an angle θ with the horizontal . If angle of elevation of highest point of trajectory is ϕ when seen from point of projection, then
View Solutionक्षैतिज दिशा से θ कोण पर एक कण को ऊपर की दिशा में प्रक्षेपित किया जाता है। किसी समय पर यदि रेखीय संवेग p, ऊर्ध्वाधर विस्थापन y, क्षैतिज विस्थापन x हो, तब उपरोक्त के मध्य कौनसा ग्राफ कण की गतिज ऊर्जा KE में परिवर्तन को प्रदर्शित नहीं करता है
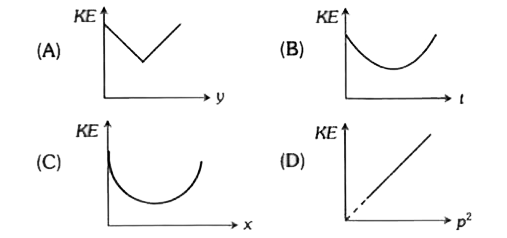
View SolutionA ball moves horizontally at a certain velocity theta It is thrown vertically, making an angle. The kinetic energy of the particle will increase with KE horizontal displacement x in this way
View Solution
