Cv and Cp denote the molar specific heat capacities of a gas at constant volume and constant pressure, respectively. Then Solution in Telugu
Cp−Cv is larger for a diatomic ideal gas than for a monoatomic ideal gas
Cp+Cv is larger for a diatomic ideal gas than for a monoatomic ideal gas
Cp/Cv is larger for a diatomic ideal gas than for a monoatomic ideal gas
Cp.Cv is larger for a diatomic ideal gas than for a monoatomic ideal gas
The correct Answer is:B, D
Step by step video, text & image solution for C_v and C_p denote the molar specific heat capacities of a gas at constant volume and constant pressure, respectively. Then by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- THERMODYNAMICSBOOK - AAKASH SERIESCHAPTER - THERMODYNAMICSEXERCISE - LECTURE SHEET (EXERCISE - II (LINKED COMPREHENSION TYPE QUESTIONS))
6 Videos
- THERMODYNAMICSBOOK - AAKASH SERIESCHAPTER - THERMODYNAMICSEXERCISE - LECTURE SHEET (EXERCISE - II (INTEGER TYPE QUESTIONS))
5 Videos
- THERMODYNAMICSBOOK - AAKASH SERIESCHAPTER - THERMODYNAMICSEXERCISE - LECTURE SHEET (EXERCISE - II (STRAIGHT OBJECTIVE TYPE QUESTIONS))
10 Videos
- THERMAL PROPERTIES OF MATTERBOOK - AAKASH SERIESCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - ADDITIONAL PRACTICE EXERCISE (LEVEL - II) PRACTICE SHEET (ADVANCED) Integer/Subjective Type Questions
2 Videos
- THERMOMETRY BOOK - AAKASH SERIESCHAPTER - THERMOMETRY EXERCISE - Numerical Exercise (LEVEL- 1)
11 Videos
Similar Questions
A gaseous mixture enclosed in a vessel of volume V consists of one gram mole if a gas ‘A’ with γ=(CpCv)=53 and another gas .B. with γ=75 at a certain of gases A and B are 4 and 32 respectively. The gases do not react with each other and are assumed to be ideal. The gaseous mixture follows the relation PV19/13 = constant in an adiabatic process [519/13=10.5]
Find the number of moles of gas B in the gaseous mixture
View SolutionCp/Cv ratio for a gas mixture consisting of 4g of helium and 32g of oxygen is N times 0.5. Then N is equal to
View SolutionThe figure shows two paths for the change of state of a gas from A to B. The ratio of molar heat capacities in path 1 and path 2 is:
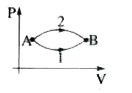
View SolutionA constant pressure gas thermometer has a volume of 200cc, 273.2cc and 525 .1cc at 0°C, 100°C and boiling point of sulphur respectively. The resistance possessed by a platinum wire at the same temperatures as above are 2.000Ω,2.778Ωand5.280Ω respectively. Estimate the boiling point of sulphur on the basis of the two sets of observations.
View SolutionIf a temperature scale defines the freezing point of water as 100∘C and the boiling as 0∘ what temperature on the scale corresponds to 25∘ C ?
View SolutionLet (C_v) and (C_p) denote the molar heat capacities of an ideal gas at constant volume and constant pressure respectively . Which of the following is a universal constant?
View SolutionC_v and C_p denote the molar specific heat capacities of a gas at costant volume and constant pressure, respectively. Then
View SolutionGiven the , Cp−Cv=R and γ=CpVv where Cp=molar specific heat at constant pressure Cv=molar specific heat at constant volume. Then Cv=
View Solutionसत्य/असत्य कथन : गैस की स्थिर दाब पर विशिष्ट ऊष्मा (Cp ), स्थिर आयतन पर विशिष्ट ऊष्मा (Cv ) से अधिक होती है।
View SolutionFor an ideal gas , the specific heat at constant pressure Cp is greater than the specific heat at constant volume Cv This is because
View SolutionLet (Cv)and(Cp) denote the molar heat capacities of an ideal gas at constant volume and constant pressure respectively . Which of the following is a universal constant?
View SolutionIf Cp and Cv are molar heats at constant pressure and constant volume respectively and R is gas constant for 1 mole, then the correct relation is
View SolutionThe molar specific heats of an ideal gas at constant pressure and volume arc denoted by CP and CV respectively. If γ=CPCVandR is the universal gas constant, then CV is equal to
View SolutionGiven the , C_p-C_v=R and gamma=(C_p)/(V_v) where C_p =molar specific heat at constant pressure C_v =molar specific heat at constant volume. Then C_v =
View SolutionShow that for an ideal gas C_p - C_v = R where C_p and C_v are the heat capacity at constant pressure and constant volume respectively.
View Solution
