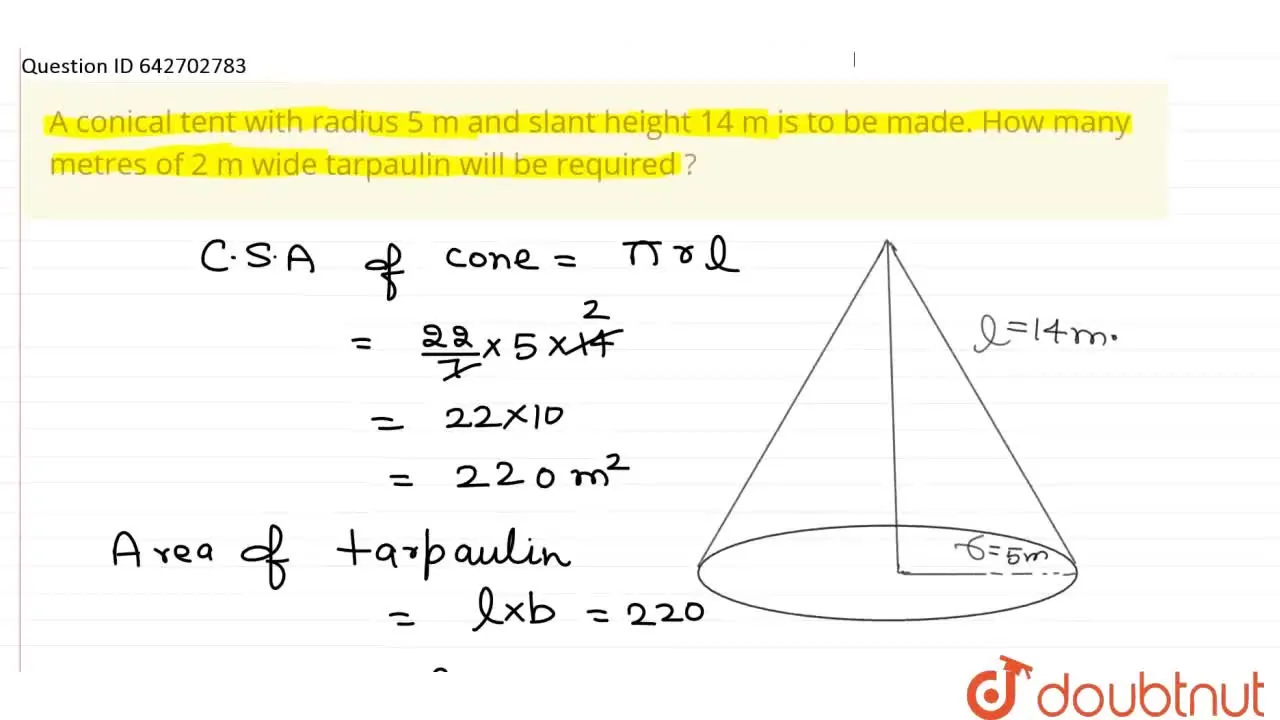
A conical tent with radius 5 m and slant height 14 m is to be made. How many metres of 2 m wide tarpaulin will be required ?
The correct Answer is:110 m
Step by step video, text & image solution for A conical tent with radius 5 m and slant height 14 m is to be made. How many metres of 2 m wide tarpaulin will be required ? by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Related Playlists
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - MULTIPLE CHOICE QUESTIONS18videos
SURFACE AREAS AND VOLUMES
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - EXERCISE 13.9 (OPTIONAL)3videos
SURFACE AREAS AND VOLUMES
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - PROBABILITY EXERCISE - Multiple Choice Questions (MCQs)10videos
PROBABILITY
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - TRIANGLESEXERCISE - SUMS TO ENRICH REMEMBER9videos
TRIANGLES
Similar Questions
5 conical tents with diameter 8 m and height 3 m are to be made. How many metres of 2 m wide tarpaulin will be required to make these 5 tents ? (π=3.14)
View SolutionThe radius of the base of a conical tent is 5 m and its height is 12 m. how many metres of canvas of width 5 metres will be required to make it? (use π=227)
View SolutionA closed cylindrical tank of diameter 14 m and height 5 m is made form a sheet of metal. How much sheet of metal will be required?
View SolutionThe height of a conical tent is 14 m and its floor area is 346.5 m2 . How much canvas , 1.1 m wide , will be required for it
View SolutionInto a conical tent of radius 8.4 m and vertical height 3.5 m, how many full bags of wheat can be emptied, if space required for the wheat in each bag is 1.96m3 ?
View SolutionA conical tent of a diameter 24 m at the base and its height 16 m.The canvas required to make it is
View SolutionA conical tent of a diameter 24 m at the base and its height 16 m. The canvas required to make it is
View SolutionA conical tent of a diameter 24 m at the base and its height 16 m. The canvas required to make it is
View SolutionA conical tent of a diameter 24 m at the base and its height 16 m. The canvas required to make it is
View SolutionA circus tent is cylindrical to a height of 4 m and conical above it. If its diameter is 105 m and its slant height is 40 m, the total area of the canvas required in m2 is (a) 1760 (b) 2640 (c) 3960 (d) 7920
View Solution