Explain the minimum deviation angle for prism by graph of deviation angle versus incidence angle. Solution in Gujrati
The graph of experimentally measured values of the angle of deviation against the values of angle of incidence i is shown in the figure.
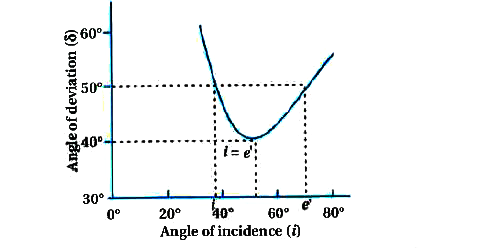
From the graph it is clear that the value of the angle of deviation becomes minimum for only one particular value of the angle of incidence i.
Also we can see that for two values of angle of incidence angle of deviation is same.
It has been experimentally established that for any given prism the ray for which the angle of incidence and angle of emergence are equal the angle of deviation is minimum for that ray.
This angle is called the angle of minium deviation of the given prism for the incident monochromatic light.
Note that,
when i=e⇒δ=δm
For prism
i+e=A+δ ...(1)
the condition for minimum deviation angle.
i=e then δ=δm
∴i+i=A+δm
∴2i=A=δm
∴i=A+δm2 ...(2)
According to Snell.s Law for AB surface,
n1sini=n2sinr1 ... (3)
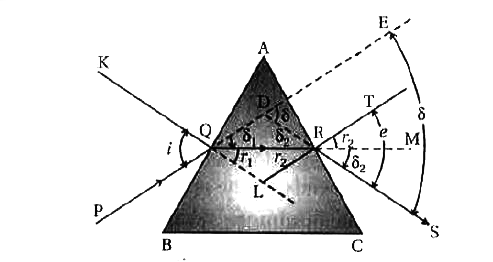
From equation (3) & (4)
n1sine=n2sinr2 ... (4) (i=e)
∴ sin i =sin e
∴n2sinr1=n2sinr2
r1=r2=r (Suppose)
r1+r2=A
r+r=A
r=A2 ... (5)
Using this result in equations (3) and (4)
n1sini=n2sinr
n1sini=n2sinA2 ... (6)
[∵ From eqn.(5)]
Using this result in equation (6)
n1sin(A+δm2)=n2sinA2
sin(A+δm2)sin(A2)=n2n1
If prism is kept in air then, n1, = 1 and n2 = 1 So, above equation is,
n=sin(A+δm2)sin(A2)
This equation shows that for a given prism value of δm depends on the angle of prism and th refractive index of the material of prism and th medium in which the prism is kept.
Remember : When δ is minium ray QR passin through the prism travels parallel to the base B of the prism.
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - KUMAR PRAKASHANCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - SECTION - A (TRY YOURSELF)
74 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - KUMAR PRAKASHANCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - SECTION-B (NUMERICALS)(NUMERICAL FROM TEXTUAL ILLUSTRATIONS)
17 Videos
- MOVING CHARGES AND MAGNETISMBOOK - KUMAR PRAKASHANCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - SECTION D (MCQs ASKED IN COMPETITIVE EXAMS)
34 Videos
- SAMPLE QUESTION PAPER BOOK - KUMAR PRAKASHANCHAPTER - SAMPLE QUESTION PAPER EXERCISE - PART-B SECTION-C
5 Videos
Similar Questions
Give range of wavelength of visible light.
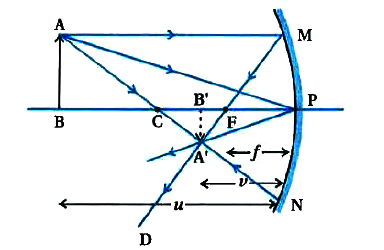
View SolutionSuppose that the lower half of the concave mirror.s reflecting surface as shown in figure is covered with an opaque (non-reflective) material. What effect will this have on the image of an object placed in front of the mirror ?
View SolutionElectric charge Q having mass M moves on a circular path of radius R with velocity →v, perpendicular to uniform magnetic field B. When a particle completes one revolution, work done by the field is _______
View SolutionTwo charges –q and +q are located at points (0, 0, –a) and (0, 0, a), respectively.
(a) What is the electrostatic potential at the points (0, 0, z) and (x, y, 0) ?
(b) Obtain the dependence of potential on the distance r of a point from the origin when r/a>>1 .
(c) How much work is done in moving a small test charge from the point (5,0,0) to (–7,0,0) along the x-axis? Does the answer change if the path of the test charge between the same points is not along the x-axis?
View SolutionAngle of minimum deviation is equal to the angle of prism A of an equilateral glass prism. The angle of incidence at which minimum deviation will be obtained is
View SolutionFigure shows the graph of angle of deviation del versus angle of incidence i for a light ray striking a prism. The prism angle is
View SolutionAngle of minimum deviation is equal to the angle prism A of an equilateral glass prism. The angle incidence at which minimum deviation will be obtained is
View SolutionFigure shows graph of deviation δ versus angle of incidence for a light ray striking a prism. Angle of prism is
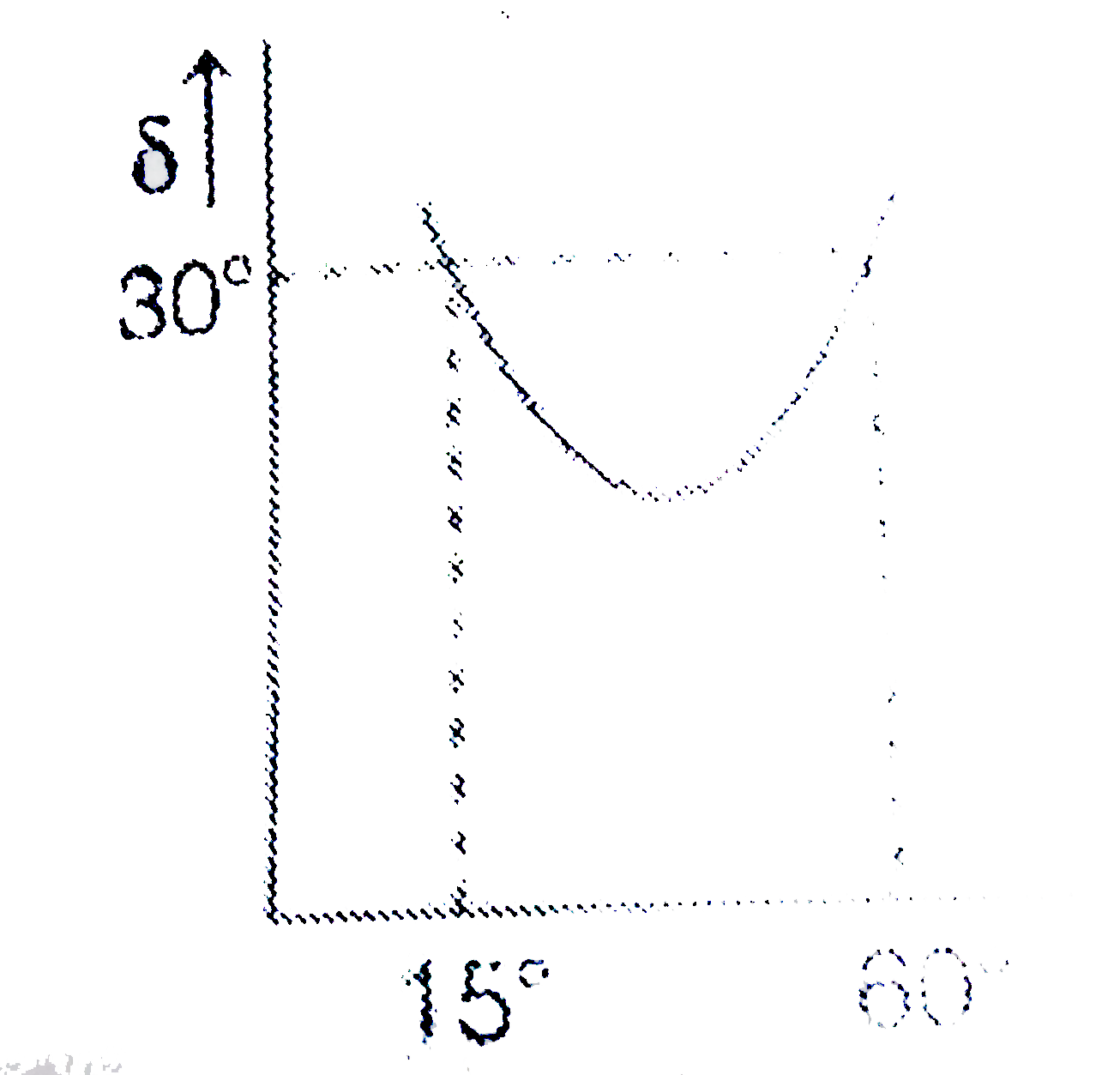
View Solutionएक समबाहु प्रिज़्म न्यूनतम विचलन की स्तिथि में है! यदि आपतन कोण प्रिज़्म का 4/5 गुना हो तो न्यूनतम विचलन का कोण होगा-
View Solutionन्यूनतम विचलन अवस्था में एक प्रकाश किरण एक समकोणिक प्रिज़्म पर एक प्रकार आपतित होती है की आपतन कोण, प्रिज़्म कोण का 34 है! न्यूनतम विचलन कोण ज्ञात कीजिए!
View Solutionन्यूनतम विचलन कोण, प्रिज्म कोण तथा आपतन कोण में संबन्ध लिखिये।
View Solutionप्रिज्म से क्या तातपर्य है? किसी प्रिज्म के लिए आपतन कोण तथा विचलन कोण का वक्र खींचकर इसे समझाइये। अल्पतम विचलन कोण से क्या तातपर्य है?
View SolutionThe refracting angle of a prism is 40^(@) . A ray of light is incident at angle 38^(@) and passes in the position of minimum deviation. The angle of minimum deviation is
View Solutionएक प्रकाश किरण किसी प्रिज्म पर 45∘ के कोण पर आपतित हो रही है| यदि यह न्यूनतम कोण से विचलित होती है और प्रिज्म कोण 60∘ है, तो न्यूनतम विचलन कोण और अपवर्तनांक ज्ञात कीजिए|
View SolutionDraw a graph of angle of deviation versus the angle of incidence in a refracting prism.
View Solution
