Let α,β be two real roots of the equation cot2x−2λcotx+3=0,λ∈R . If cot(α+β)=12 , then value of λ is :
A
1
B
2
C
12
D
32
Video Solution
Text Solution
The correct Answer is:B
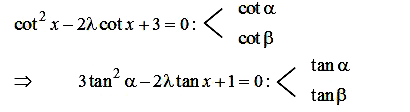
tanα+tanβ=2λ3,tanαtanβ=13
∴cot(α+β)=12⇒tanα+tanβ1−tanαtanβ=2
⇒2λ31−13=2⇒λ=2
|
Similar Questions
Knowledge Check
Let αandβ be two real roots of the equation 5cot2x−3cotx−1=0, then cot2(α+β)=
A2B3C4D5Let α and β be two real roots of the equation 5cot2x−3cotx−1=0 , then cot2(α+β) =
A2B3C4D5If α,β be the roots of the the equation x2−2x+2=0 and cotθ=x+1 then (x+α)n−(x+β)nα−β=
AcosnθsinnθBsinnθcosnθCsinnθsinnθDcosnθcosnθ
If cot(α+β)=0, then sin(α+2β)=
View SolutionLet α,β are the two real roots of equation x2+px+q=0,p,q∈R,q≠0. If the quadratic equation g(x)=0 has two roots α+1α,β+1β such that sum of roots is equal to product of roots, then the complete range of q is:
View SolutionLet αandβ be the roots of x2+x+1=0, then the equation whose roots are α2020andβ2020 is
View SolutionLet α and β be the roots of the equation x2+x+1=0.
Then, for y≠0 in R.
∣∣ ∣ ∣∣y+1αβαy+β1β1y+α∣∣ ∣ ∣∣ is
View SolutionIf cotαandcotβ are the roots of the equation x2+bx+c=0 with b≠0 then the value of cot(α+β) is :
View Solution