A very long straight conductor and an isosceles triangular conductor lie in a plane and are separated from each other as shown in the figure. If a = 10 cm, b = 20 cm, and h = 10 cm find the coefficient of mutual induction.
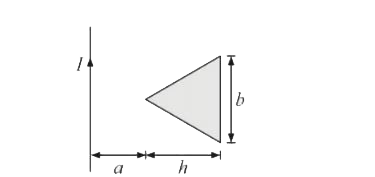
2×10−7[1+ln2]
4×10−8[1+ln2]
4×10−9[1+ln2]
2×10−10[1+ln2]
The correct Answer is:C
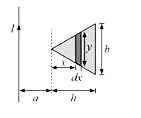
Consider an elementary section of coil as shown in figure. Area of this section
dA=ydx
but yb=xh⇒y=bhx
dA=bhxdx
Magnetic flux linked with this section,
dϕ=BdA=μ0hI2π(a+x)⋅bhxdx⇒dϕ=μ0hIb2πh⋅xa+xdx
∴ Total magnetic flux linked with the coil
ϕ=μ0hIb2πh∫h0xa+xdx
=μ0hIb2πh[x−aln|a+x|]h0=2×10−7×20×10−210×10−2 [10×10−2+10×10−2ln∣∣∣21∣∣∣]
=4×10−9[1+ln2]H
Similar Questions
A very long staright conductor and an isoceles triangular conductor lie in a plane and separated from each other as shown in the figure.
Given a=10cm, b=20cm and h=10cm
(a) Find their coefficeint of mutual induction.
(b) If current in the staright wire is increasing at a rate of 2A/s , find the direction and magnitude of current in the triangular wire. [Diameter of the wire crosss-section d=1mm, resistivity of the wire, ρ=1.8×10−8Ω−m]
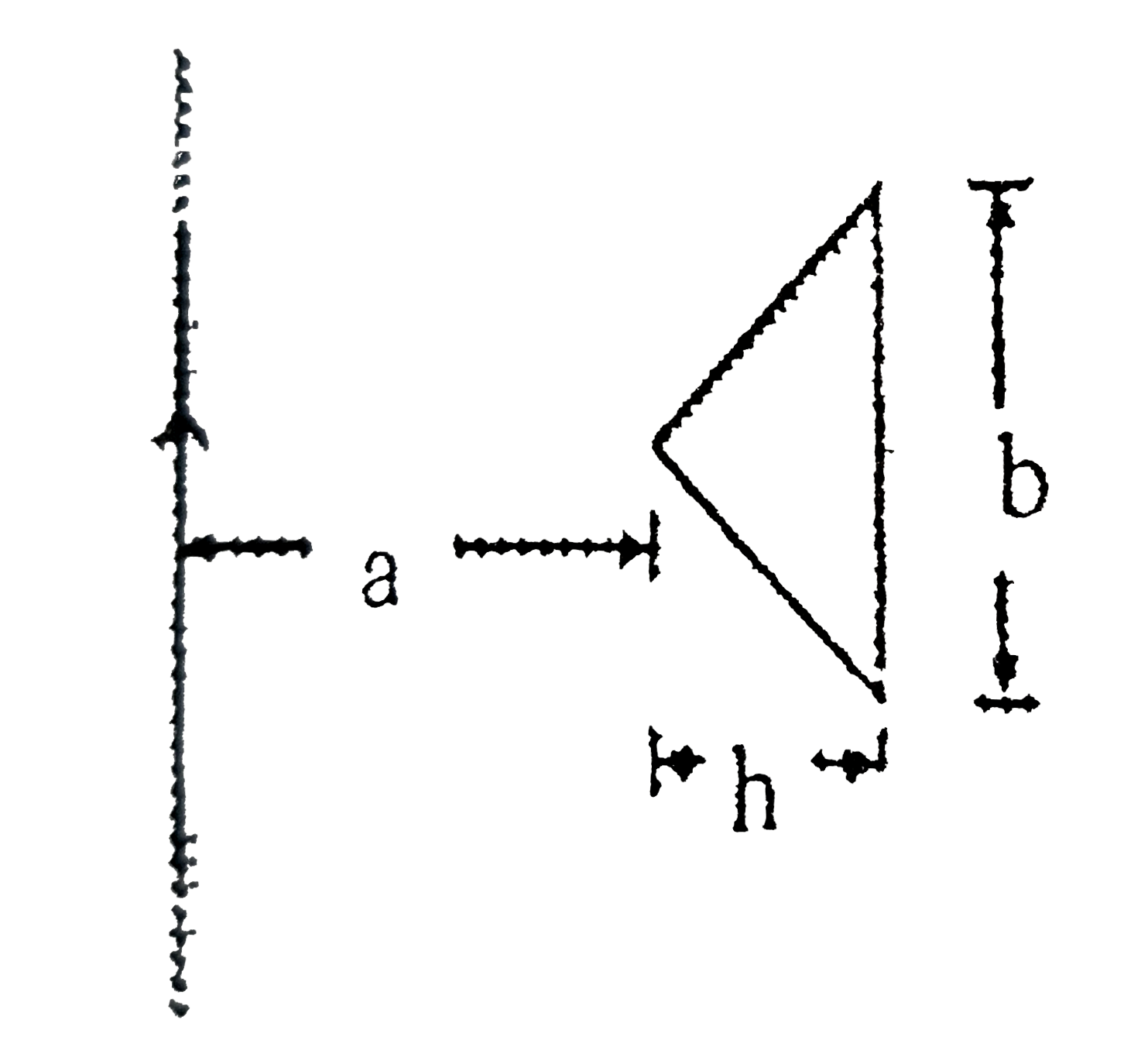
View SolutionA very long conductor and an isosceles triangular conductor lie in a plane and are separated from each other as shown in the figure. Find the coefficient of mutual induction. (a = 10 cm, b = 20 cm, h = 10 cm)
View Solution
Knowledge Check
In the figure AD=12cm,AB=20cmandAE=10cm. Find EC :
 A14 cmB10 cmC8 cmD15 cm
A14 cmB10 cmC8 cmD15 cmTwo long wires are placed parallel to each other 10cm apart as shown in figure. The magnetic field at point P is
 A5/6×10−3T directed perpendicular into the paperB1/3×10−3T directed perpendicular out of the paperC5/6×10−3T directed perpendicular out of the paperD1/6×10−3T directed perpendicular into the paper
A5/6×10−3T directed perpendicular into the paperB1/3×10−3T directed perpendicular out of the paperC5/6×10−3T directed perpendicular out of the paperD1/6×10−3T directed perpendicular into the paperThree very long straight current carrying conductors are placed parallel to each other as shown in. The conductors A and C are fixed whereas conductors B is free to move. If the conductor B is pulled towards right through a very small distance r, then what is the net force acting on it and the angular frequency of the resulting oscillation.
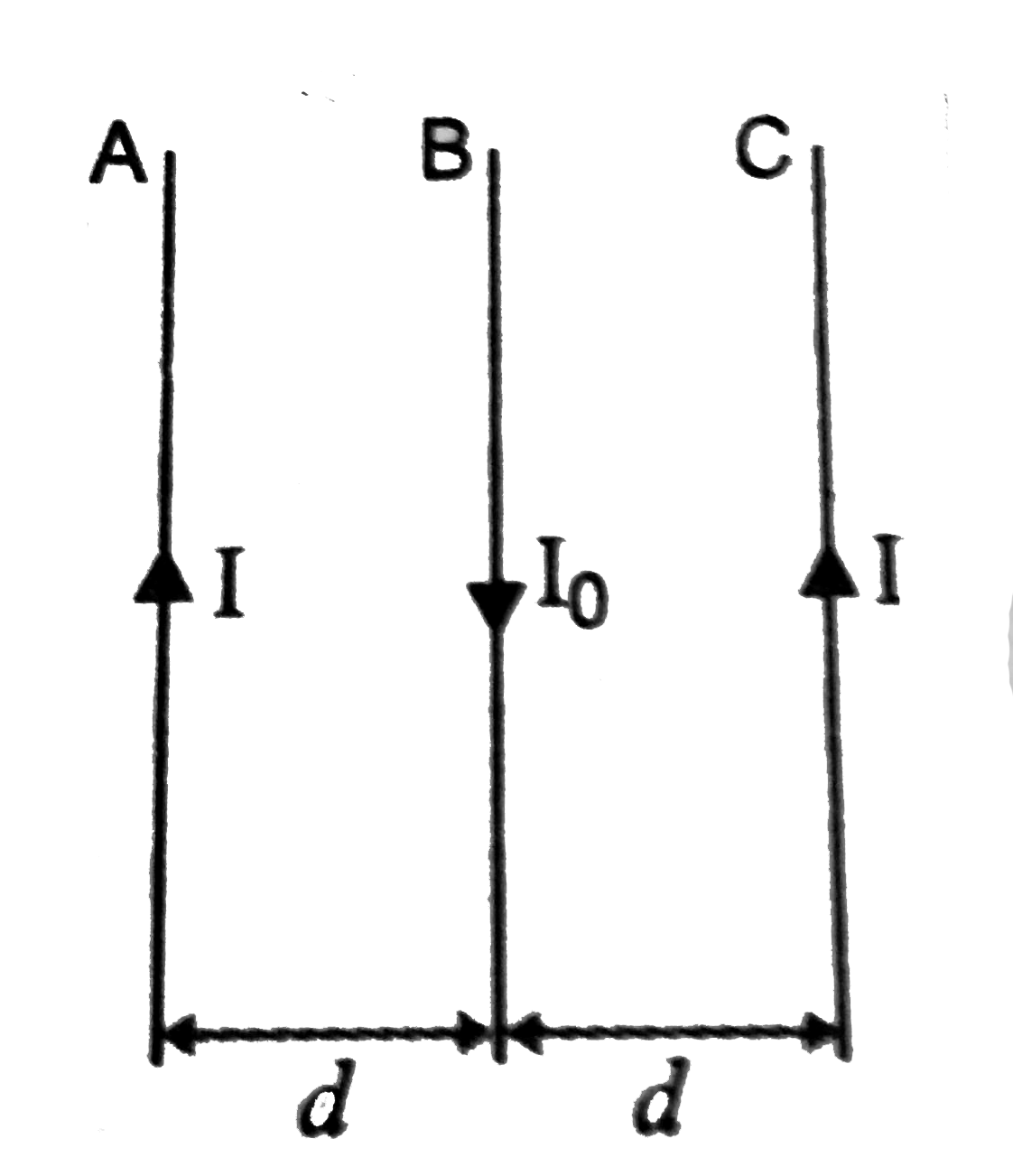 Aμ02II0πd2, √μ0II0πmd2Bμ02II04πr2, √μ0II02πmd2Cμ0II02πr2, √μ0II04πmdDμ0II02π[(d+r)−(d−r)d2−r2], √μ0II0πmd2
Aμ02II0πd2, √μ0II0πmd2Bμ02II04πr2, √μ0II02πmd2Cμ0II02πr2, √μ0II04πmdDμ0II02π[(d+r)−(d−r)d2−r2], √μ0II0πmd2
In an isosceles triangle, the equal sides are each 10 cm and the base is 12 cm. Find the altitude of the triangle.
View SolutionIn an isosceles triangle, altitude is 12 cm and the base is 10 cm each. Find the measure of equal sides.
View SolutionIn figure bcdf is a circular coil of non-insulated thin uniform conductors, ab and de are very long straight parallel conductors, ab and de are very long straight parallel conductors, tangential to the coil at the points b and d. If the current 5A enters the coil from a to b, find the magnetic field induction at O, the centre of the coil. The diameter of the coil is 10cm.

View SolutionFind the magnetic moment of the current carrying loop OABCO shown in figure.

Given that i=4.0A,OA=20cm and AB=10cm.
View SolutionFind the area of an isosceles triangle whose equal sides are 8 cm each and the third side is 10 cm ?
View Solution
