कथन-1 यदि f(x)=[cosx+sinx],0<x<2π जहां [x], x के पूर्णांक भाग को प्रदर्शित करता है तब f(x),5 बिंदुओं पर असतत होगा।
कथन-2 x=π2,3π4,π,5π4,3π2 के लिए बाएं सीमा तथा दाएं सीमा बराबर नहीं है।
कथन -1 सही है कथन -2 सही है कथन -1 के लिए, कथन -2 का स्पष्टीकरण सही है
कथन-1 सही है कथन -2 सही है: कथन-1 के लिए , कथन -2 का स्पष्टीकरण सही नहीं है
कथन-1 सही है, कथन -2 गलत है
कथन-1 गलत है : कथन-2 सही है
The correct Answer is:A
Step by step video, text & image solution for कथन-1 यदि f(x)=[cosx+sinx],0ltxlt2pi जहां [x], x के पूर्णांक भाग को प्रदर्शित करता है तब f(x),5 बिंदुओं पर असतत होगा। कथन-2 x=(pi)/2,(3pi)/4,pi,(5pi)/4,(3pi)/2 के लिए बाएं सीमा तथा दाएं सीमा बराबर नहीं है। by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- फलन, सीमा, सांतत्य तथा अवकलनीयताBOOK - ERRORLESS HINDICHAPTER - फलन, सीमा, सांतत्य तथा अवकलनीयताEXERCISE - JEE ADVANCED( INTEGER TYPE)
40 Videos
- फलन, सीमा, सांतत्य तथा अवकलनीयताBOOK - ERRORLESS HINDICHAPTER - फलन, सीमा, सांतत्य तथा अवकलनीयताEXERCISE - JEE ADVANCED( MATCH THE COLUMN TYPE)
13 Videos
- फलन, सीमा, सांतत्य तथा अवकलनीयताBOOK - ERRORLESS HINDICHAPTER - फलन, सीमा, सांतत्य तथा अवकलनीयताEXERCISE - JEE ADVANCED( MORE THAN ONE CORRECT ANSWERS)
60 Videos
- प्रायिकताBOOK - ERRORLESS HINDICHAPTER - प्रायिकताEXERCISE - EXERCISE
99 Videos
- लघुगणक और आंशिक भिन्नें BOOK - ERRORLESS HINDICHAPTER - लघुगणक और आंशिक भिन्नें EXERCISE - JEE Advanced (integer type questions)
8 Videos
Similar Questions
माना एक फलन जो संबंध
f(2tanx1+tan2x)=(1+cos2x)(sec2x+2tanx)2 को संतुष्ट करता है।
कथन-1 y=f(x) का परिसर R है।
कथन-2: रेखीय फलन का परिसर R होगा यदि प्रांत R है।
View Solutionकॉलम I में दिए गए वक्तव्यों/व्यंजकों का कॉलम II में दिये गये मानों से सुमेल करे।
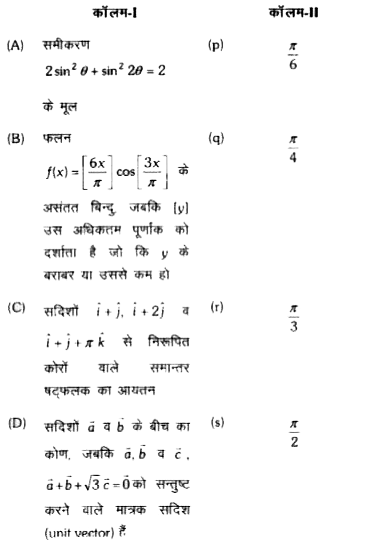
View Solutionमान लीजिए f:R→R एक फलन है जो समीकरण f(x+y)=f(x)+f(y),∀x,yεR को संतुष्ट करता हैक् यदि x=0 पर फलन f(x) अवकलनीय है तो
View SolutionA, B, C तीन परस्पर स्वतंत्र घटनायें हैं। S1 तथा S2 दो कथनों को देखने पर
S1 : A तथा B∪C स्वतन्त्र हैं
S2 : A तथा B∩C स्वतन्त्र हैं तब
View Solutionपद 6+log32⎛⎜ ⎜⎝13√2 ⎷4−13√2 ⎷4−13√2√4−13√2…⎞⎟ ⎟⎠ का मान है
View SolutionDifferentiate sin−1{sinx+cosx√2}, −3π4<x<π4 with respect to x
View Solutionwhich of the following statement is/are true ?
(i) f(x) =sin x is increasing in interval [−π2,π2]
(ii) f(x) = sin x is increasing at all point of the interval [−π2,π2]
(3) f(x) = sin x is increasing in interval (−π2,π2)UU(3π2,5π2)
(4) f(x)=sin x is increasing at all point of the interval (−π2,π2)UU(3π2,5π2)
(5) f(x) = sin x is increasing in intervals [−π2,π2]&[3π2,5π2]
View SolutionLet f(x)=sinx+2cos2x,π4≤x≤3π4. Then f attains its-
View Solutionनिम्न कथन सत्य या असत्य है
यदि f(x)=√x−2 तो : fof=f2
View SolutionSometimes functions are defined like f(x)=max{sinx,cosx} , then f(x) is splitted like f(x)={{:(cosx, x in (0,(pi)/(4)]),(sinx, x in ((pi)/(4),(pi)/(2)]):} etc. If f(x)=max{(1)/(2),sinx} , then f(x)=(1)/(2) is defined when x in
View Solutionयदि 3x=4x−1 तब x बराबर है
View Solutionयदि f(x)=Asin(πx2)+B,.f'(12)=√2 तथा ∫10f(x)dx=2Aπ तब A तथा B के मान है
View SolutionRange of f(x)=sin−1x+tan−1x+sec−1x is (a)(π4,3π4) (b) [π4,3π4] (c){π4,3π4} (d) none of these
View SolutionIf f(x)=√1−sin2x , then f′(x) is equal to
(a)−(cosx+sinx),for x∈(π4,π2)
(b)cosx+sinx ,for x∈(0,π4)
(c)−(cosx+sinx),for x∈(0,π4)
(d)cosx−sinx ,for x∈(π4,π2)
View SolutionDifferentiate sin−1{sinx+cosx√2}, −3π4<x<π4 with respect to x
View Solution
