Find the potential function V (x,y) of an electrostatic field →E=2axyˆi+a(x2−y2)ˆj where a is a constant. Solution in Telugu
v0+ax2y−ay33
V0−axy2−ay23
V0+axy2+ay33
V0−ax2y+ay33
The correct Answer is:D
Step by step video, text & image solution for Find the potential function V (x,y) of an electrostatic field vec(E)=2axy hat(i)+a(x^(2)-y^(2))hat(j) where a is a constant. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ELECTROSTATICSBook:AAKASH SERIESChapter:ELECTROSTATICSExercise:LECTURE SHEET (EXERCISE - IV) (LEVEL - II (ADVANCED) STRAIGHT OBJECTIVE TYPE QUESTIONS)
Explore 6 Videos
- ELECTROSTATICSBook:AAKASH SERIESChapter:ELECTROSTATICSExercise:LECTURE SHEET (EXERCISE - IV) (LEVEL - II (ADVANCED) MORE THAN ONE CORRECT ANSWER TYPE QUESTIONS)
Explore 4 Videos
- ELECTROSTATICSBook:AAKASH SERIESChapter:ELECTROSTATICSExercise:LECTURE SHEET (EXERCISE - III) (LEVEL - II (ADVANCED) INTEGER TYPE QUESTIONS)
Explore 2 Videos
- ELECTROMAGNETICSBook:AAKASH SERIESChapter:ELECTROMAGNETICSExercise:ADDITIONAL EXERCISE
Explore 6 Videos
- ELEMENTS OF VECTORSBook:AAKASH SERIESChapter:ELEMENTS OF VECTORSExercise:QUESTIONS FOR DESCRIPTIVE ANSWERS
Explore 9 Videos
Similar Questions
Two metallic solid spheres of radii R and 2R are changed such that both of them have same charge density σ. If the spheres are located for away from each other and connected by a conducting wire, the new charge density on bigger sphere is :
View SolutionFour charges of 1 mC, 2mC, 3mC and- 6 mC are placed one at each corner of the square of side 1m. The square lies in the x-y plane with its centre at the origin.
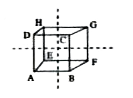
View SolutionTwo infinite line charges, each having a uniform density λ. Pass through the midpoints of two pairs of opposite faces of a cube of edge L as shown in figure. The modulus of the total electric flux due to both the line charges through the face is ABCD is λL/(kε0) find the value of k ?
View SolutionWhat is the radius of the path of an electron (mass 9 × 10-31 kg and charge 1.6×x10–19 C) moving at a speed of 3×x107 m/s in a magnetic field of 6x×10−4 T perpendicular to it? What is its frequency? Calculate its energy in keV. (1eV=1.6×10−19J)
View SolutionFind the components of vector ¯¯¯A=2ˆi+3ˆj along the directions of ˆi+ˆjandˆi−ˆj
View SolutionFind the potential V of an electrostatic field →E=a(yˆi+xˆj), where a is a constant.
View SolutionIf electric field is (a) vec E = 2 a x y hat(i) + a(x^(2) - y^(2)) hat(j) (b) vec(E ) = ay hat(i) + (ax + bz) hat(j) + by hat(k)) Find potential.
View SolutionThe potential field of an electric field vec(E)=(y hat(i)+x hat(j)) is
View SolutionFor given vec(E)=2x hat(i)+3yhat(j) , find the potential at (x, y) if V at origin is 5 volts.
View SolutionIf →E=2yˆi+2xˆj, then find V(x,y,z)
View SolutionIf →E=2x2ˆi−3y2ˆj, then V (x, y, z)
View SolutionThe potential phi(x,y) of an electrostatic field E=a(y hat(i)+c hat(j)) is [ a is a constant and hat(i) and hat(j) are unit vectors along X and Y axes ]
View SolutionFind the values of x, y and z so that the vectors →a=xˆi+2ˆj+zˆkand →b=2ˆi+yˆj+ˆkare equal.
View Solutionfind the value of x,y and z so that the vectors →a=xˆi+2ˆj+zˆkand→b=2ˆi+yˆj+ˆk are equal
View SolutionThe potential field of an electric field →E=(yˆi+xˆj) is
View SolutionThe potential field of an electric field vec(E)=(y hat(i)+x hat(j)) is
View Solution
