A steel wire, 2mm in diameter, is just stretched between two fixed points at a temperature of 30∘C . Determine its tension when the temperature falls to 20∘C . (Coefficient of linear expansion of steel = 0.000011/∘C, Young's modulus for steel =2.0×1011Nm−2) Solution in Telugu
Thermal stress =FA=YαΔt
Tension in the wire =YαA(t2−t1)
Here Y=2.0×1011Nm−2,α=11×10−6/∘C,t2=30∘C,t1=20∘C, radius = 1mm =1×10−3m,
A=πr2=π(1×10−3)2=π×10−6m2
The tension in the wire
=2.0×1011×11×10−6×π×10−6×(30−20)=69.14N
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH SERIESCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - EXERCISE -IA
60 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH SERIESCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - EXERCISE -IA (MATCHING)
4 Videos
- MECHANICAL PROPERTIES OF FLUIDS BOOK - AAKASH SERIESCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - EXERCISE-3
43 Videos
- MOTION IN A PLANE BOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANE EXERCISE - Practice Exercise
84 Videos
Similar Questions
A spring is stretched by applying a load to its free end. The strain produced in the spring is
View SolutionA spring of young's modulus 2×1011 pa is suspended vertically and subjected to a load of 5 kg and elongation is 2 mm. when the load is doubled - match the following (g=9.8m/s2)
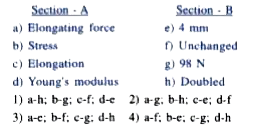
View SolutionA U-tube contains water and methylated spirit separated by mercury. The mercury columns in the two arms are in level with 10.0cm of water in one arm and 12.5cm of spirit in the other. What is the specific gravity of spirit.
View SolutionA person runs along a circular path of radius 5m. Find the magnitude of the displacement if he runs all the way around the circle ?
View SolutionA steel wire 2mm in diameter is stretched between two clamps, when its temperature is 40^@C Calculate the tension in the wire, when its temperature falls to 30^@C Given, coefficient Y for steel = 21 xx 10^(11) dyn e//cm^2
View SolutionA steel wire of length 20 cm and uniform cross-section 1mm^(2) is tied rigidly at both the ends. If the temperature of the wire is altered from 40^(@)C to 20^(@)C , the change in tension. [Given coefficient of linear expansion of steel is 1.1xx10^(5) .^(@)C^(-1) and Young's modulus for steel is 2.0xx10^(11) Nm^(-2) ]
View SolutionA tungsten wire, 0.5mm in diameter, is just strechted between two fixed points at a temperture of 40^(@)C . Determine the tension in the wire when the temperature falls to 20^(@)C . (coefficant of linear expansion of tungsten = 4.5xx10^(-6)//.^(0)C, Young's modulus of tungsten = 3.4xx10^(10) Nm^(-2))
View SolutionA steel rod of length 5m is fixed between two support. The coefficient of linear expansion of steel is 12.5×10−6/∘C. Calculate the stress (in 108N/m2) in the rod for an increase in temperature of 40∘C. Young's modulus for steel is 2×1011Nm−2
View SolutionA steel wire 2mm in diameteris ust streched between two dixed point at a temperature of 20^(@)C . Determine its tension when its temperature falls to 10^(@)C . Linear expansivity of steel =11 xx 10^(-6)//K, Young modulus =2 xx 10^(11)//m^(-2)
View SolutionA steel wire 2 mm is diameter is just stretched between two fixed points at a temperature of 20^(@)C . If the temperature falls to 10^(@)C , then the tension in the wire is (Coefficient of linear expansion of steel (alpha) is 11xx10^(-6)//""^(@)C and Y_(s)=2.1xx10^(11)N//m^(2) )
View SolutionA steel wire 2 mm in diameter is just stretched between two fixed points at a temperature of 20^(@)C. If the temperature falls to 10^(@)C, then the tension in the wire is (The coefficient of linear expansion of steel = 11xx10^(-6)//^(@)C and Y for steel 2.1 xx10^(11)N//m^(2))
View SolutionA steel wire of cross-sectional area 0.5mm2 is held between two fixed supports. If the wire is just taut at 20∘C, determine the tension when the temperature falls to 0∘C. Coefficient of linear expansion of steel is 1.2×10−5C−1 and its Young's modulus is 2.0×1011Nm−2.
View SolutionCalculate the force required to prevent a steel wire of 1mm2 cross-section from contracting when it cools from 60∘C to 15∘C, if Young's modulus for steel is 2×1011Nm−2 and its coefficient of linear expansion is 0.000011^@C^-1.
View SolutionA tungsten wire, 0.5 mm in diameter, is just stretched between two fixed points at a temperature of 40^@C . Determine the tension in the wire when the temperature falls to 20^@C . (coefficient of linear expansion of tungsten = 4.5 xx 10^(-6)//""^@C , Young's modulus of tungsten = 3.45 xx 10^11Nm^(-2) )
View SolutionA steel wire 2mm in diameter is just stretched between two rigid walls at 20°C. If the temperature falls to 10°C, find the tension in the wire. Linear coefficient of expansion of steel = 11×10−6/kandY=2×1011N/m2
View Solution
