A block of mass 1 kg is fastended to one end of a wire of cross-sectional area 2 mm2 and is rotated in a vertical circle of radius 20 cm. The speed of the block at the bottom of the circle is 3.5 ms−1. Find the elongation of the wire when the block is at the top of the circle. Solution in Telugu
i) Tension at the bottom of the circle,
T=mv2r+mg=1×(3.5)20.2+1×9.8
=61.25+9.8=71.05N
This tension in the string is equal to the force, F i.e., F=71.05N, L =r=0.2m.
The increase in length,
e=FLAY=71.05×0.22×10−6×2×1011
=3.553×10−5m
ii) Tension at the top of the circle, T = Tension at the bottom - 6 mg
=71.05−6×1×9.8=71.05−58.8=12.25N.
F= 12.25 N, L = 0.2 m.
The increase in length =FLAY
=12.25×0.22×10−6×2×1011
=0.6125×10−5m
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH SERIESCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - EXERCISE -IA
60 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH SERIESCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - EXERCISE -IA (MATCHING)
4 Videos
- MECHANICAL PROPERTIES OF FLUIDS BOOK - AAKASH SERIESCHAPTER - MECHANICAL PROPERTIES OF FLUIDS EXERCISE - EXERCISE-3
43 Videos
- MOTION IN A PLANE BOOK - AAKASH SERIESCHAPTER - MOTION IN A PLANE EXERCISE - Practice Exercise
84 Videos
Similar Questions
A spring is stretched by applying a load to its free end. The strain produced in the spring is
View SolutionA spring of young's modulus 2×1011 pa is suspended vertically and subjected to a load of 5 kg and elongation is 2 mm. when the load is doubled - match the following (g=9.8m/s2)
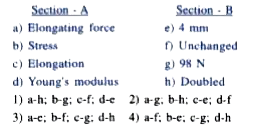
View SolutionA U-tube contains water and methylated spirit separated by mercury. The mercury columns in the two arms are in level with 10.0cm of water in one arm and 12.5cm of spirit in the other. What is the specific gravity of spirit.
View SolutionA person runs along a circular path of radius 5m. Find the magnitude of the displacement if he runs all the way around the circle ?
View SolutionA block of weight 10 N is fastened to one end of a wire of cross sectional area 3mm2 and is rotated in a vertical circle of radius 20 cmk. The speed of the block at the bottom of the circle is 2ms−1. Find the elongation of the wire when the block is at the bottom of the circle. Young modulus of the material of the wire =2×1011Nm−2.
View SolutionA block of mass 1 Kg is fastened to one each of a wire of cross-sectional area of 2mm^(2) and is rotated in verticle circle of radius 20cm . The speed of the block at the bottom of the circle is 3.5ms^(-1) . The elongation of the wire when the block is at top of the circle
View SolutionA body of mass 1 kg is fastened to one end of a steel wire of cross- section area 3 xx 10^(-6)m^(2) and is rotated in horizantal circle of radius 20 cm with a constant speed 2 m//s . Find the elongation of the wire (Y= 2 xx 10^(11) N//m^(2))
View SolutionA block of weight 10 N is fastened to one end of wire of cross-sectional area 4mm2 and is rotated ina vertical circle of radius 30 cm. The speed of the block at the bottom of the circle is 3ms−1. Find the elongation of the wire when the block is at the bottom of the circle . Young's modulus of the material of the wire =2×1011Nm−2.(g=10ms−2)
View SolutionA 14.5 kg mass fastened to the end of a steel wire of unstretched length 1.0 m is whirled in a vertical circle with an angular velocity of 2rev.s−1 at the bottom of the circle. The cross sectional area of the wire is 0.065cm2. Calculate the elongation of the wire when the mass is at the lowest point of its path.
View SolutionA 14.5 kg mass, fastened to the end of a steel wire of unstretched 1.0 m, is whirled in a vertical circle with an angular velocity of 2 rev/s at the bottom of the circle. The cross- sectional area of the wire is 0.065 cm2. Calculate the elongation of the wire when the mass is at the lowest point of its path.
View SolutionA 14.5 kg mass, fastened to the end of a steel wire of unstretched 1.0 m, is whirled in a vertical circle with an angular velocity of 2 rev/s at the bottom of the circle. The cross- sectional area of the wire is 0.065 cm2. Calculate the elongation of the wire when the mass is at the lowest point of its path.
View SolutionA 14.5 kg mass, fastened to the end of a steel wire of unstretched 1.0 m, is whirled in a vertical circle with an angular velocity of 2 rev/s at the bottom of the circle. The cross- sectional area of the wire is 0.065 cm2. Calculate the elongation of the wire when the mass is at the lowest point of its path.
View SolutionA block of weight 10 N is fastened to one end of a wire of cross sectional area 3mm2 and is rotated in a vertical circle of radius 20 cm. The speed of the block at the bottom of the circle is 2ms−1. Find the elongation of the wire when the block is at the bottom of the circle. Young modulus of the material of the wire =2×1011Nm−2.
View SolutionA block of mass 1 kg is fastended to one end of a copper wire of cross- sectional area 1mm2 and is rotated in a horizontal circle of radius 20 cm. If the breaking stress of copper is 5×108Nm−2, find the maximum number of revolutions the block make in the minute without the string breaking.
View SolutionA block of mass 1 kg is fastended to one end of a copper wire of cross- sectional area 1mm2 and is rotated in a horizontal circle of radius 20 cm. If the breaking stress of copper is 5×108Nm−2, find the maximum number of revolutions the block make in the minute without the string breaking.
View SolutionA block of mass 1 kg is fastended to one end of a wire of cross-sectional area 2 mm^(2) and is rotated in a vertical circle of radius 20 cm. The speed of the block at the bottom of the circle is 3.5 ms^(-1) . Find the elongation of the wire when the block is at the top of the circle.
View Solution
