Force acting on a particle is (2ˆi+3ˆj)N. Work done by the force is zero, when a particle is moved along the line 3y+kx=5. Here the value of k is Solution in Telugu
2
4
6
8
The correct Answer is:A
Step by step video, text & image solution for Force acting on a particle is (2hati+3hatj)N. Work done by the force is zero, when a particle is moved along the line 3y+kx=5. Here the value of k is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - WORK -POWER-ENERGYEXERCISE - LECTURE SHEET (EXERCISE-I WORKDONE BY FORCE MORE THAN ONE CORRECT ANSWER TYPE QUESTIONS)2videos
WORK -POWER-ENERGY
- BOOK - AAKASH SERIESCHAPTER - WORK -POWER-ENERGYEXERCISE - LECTURE SHEET (EXERCISE-I WORKDONE BY FORCE LINKED COMPREHENSION TYPE QUESTIONS)2videos
WORK -POWER-ENERGY
- BOOK - AAKASH SERIESCHAPTER - VISCOSITY EXERCISE - Questions for Descriptive Answers3videos
VISCOSITY
- BOOK - AAKASH SERIESCHAPTER - WORK POWER ENERGYEXERCISE - EXERCISE - 361videos
WORK POWER ENERGY
Similar Questions
A constant force F acts on a moving body along a smooth quarter circular arc of radius R fixed on horizontal surface. Calculate the work done by force F from A to B
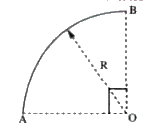 View Solution
View SolutionA block of mass 2.5 kg is pushed 2.2. m along a frictionless horizontal table by a constant 16 N force directed 45∘ above the horizontal. Then
Workdone by the applied force is03:07View SolutionAn iron sphere of mass 20×10−3 kg falls through a viscous liquid with terminal velocity 0.5 m/sec. Find the terminal velocity of another iron sphere of mass 54×10−2 kg.
02:41View SolutionIf →A+→B=→Rand2→A+→B is perpendicular to →B then
01:11View SolutionForce acting on a particle is )jˆ3 iˆ (2 N. Work done by this force is zero. when a particle is moved on the line 3y + kx = 5. Here value of k is:
 View Solution
View SolutionForce acting on a particale is (2hat(i)+3hat(j))N . Work done by this force is zero, when the particle is moved on the line 3y+kx=5 . Here value of k is ( Work done W=vec(F).vec(d))
03:43View SolutionA particle of mass m initially moving with speed v.A force acts on the particle f=kx where x is the distance travelled by the particle and k is constant. Find the speed of the particle when the work done by the force equals W.
03:43View SolutionA force →F=2ˆi−3ˆj+7ˆk(N) acts on a particle which undergoes a displacement →r=7ˆi+3ˆj−2ˆk
(M). Calculate the work done by the forceView SolutionThe work done by the force →F=2ˆi−3ˆj+2ˆk in moving a particle from (3, 4, 5) to (1, 2, 3) is :
03:22View SolutionA uniform force of (2ˆi+ˆj) N acts on a particle of mass 1 kg. The particle displaces from position (3ˆj+ˆk) m to (5ˆi+3ˆj) m. The work done by the force on the particle is
03:43View SolutionA uniform force of (2ˆi+ˆj) N acts on a particle of mass 1 kg. The particle displaces from position (3ˆj+ˆk) m to (5ˆi+3ˆj) m. The work done by the force on the particle is
03:21View SolutionOn a particle (2hati+ 3hatj) N After the ball acts, the action is zero. If the particle 3y+kx = 5 Is moving along the line, however k What is its value?
03:21View SolutionForce acting on a particle is (2hati+3hatj) N. Work done by this force is zero ,when a particle is moved on the line 3y+kx=5 . Here value of k is
View SolutionA uniform force of (2ˆi+ˆj) N acts on a particle of mass 1 kg. The particle displaces from position (3ˆj+ˆk) m to (5ˆi+3ˆj) m. The work done by the force on the particle is
03:46View SolutionForce acting on a particle is (2ˆi+3ˆj)N. Work done by the force is zero, when a particle is moved along the line 3y+kx=5. Here the value of k is
02:42View Solution
