समीकरणों x=2at1+t2,y=a(1−t2)1+t2(−1≤t≤1) से दत्त बिन्दु का बिन्दुपथ होगा
एक सरल रेखा
एक वृत्त
एक दीर्घवृत्त
एक अतिपरवलय
The correct Answer is:B
Step by step video, text & image solution for समीकरणों x=(2at)/(1+t^(2)), y=(a(1-t^(2)))/(1+t^(2))(-1 le t le 1) से दत्त बिन्दु का बिन्दुपथ होगा by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- वृत्त तथा वृत्तों का निकाय BOOK - ERRORLESS HINDICHAPTER - वृत्त तथा वृत्तों का निकाय EXERCISE - JEE Advanced (More than one correct answers)
28 Videos
- वृत्त तथा वृत्तों का निकाय BOOK - ERRORLESS HINDICHAPTER - वृत्त तथा वृत्तों का निकाय EXERCISE - JEE Advanced (Reasoning type questions )
8 Videos
- वृत्त तथा वृत्तों का निकाय BOOK - ERRORLESS HINDICHAPTER - वृत्त तथा वृत्तों का निकाय EXERCISE - Solved Multiple Choice Questions (वृत्त की स्पर्श रेखा तथा अभिलम्ब)
72 Videos
- वर्ग समीकरण एवं असमिकायें BOOK - ERRORLESS HINDICHAPTER - वर्ग समीकरण एवं असमिकायें EXERCISE - JEE Advanced (Matrix Match type questions)
2 Videos
- शांकव परिच्छेद BOOK - ERRORLESS HINDICHAPTER - शांकव परिच्छेद EXERCISE - JEE Advanced (Matrix Match type questions)
7 Videos
Similar Questions
माना कि L1 मूलबिन्दु से गुजरने वाली एक सरल रेखा है तथा सरल रेखा L2 का समीकरण x+y=1 है। यदि वृत्त x2+y2−x+3y=0 द्वारा L1 और L2 पर काटे गये अन्तःखण्ड बराबर हैं, तो निम्नलिखित में से कौन-सा समीकरण रेखा L1 को निरूपित करता है
View Solutionमाना L1=2x+3y+p−3=0,L2:2x+3y+p+3=0
जहाँ p एक वास्तविक संख्या है तथा C=x2+y2+6x−10y+30=0
कथन-1 : यदि रेखा L1 वृत्त C की जीवा है, तब रेखा L2 सदैव वृत्त C का व्यास नही होगी तथा
कथन-2 : यदि रेखा L1 वृत्त C का व्यास है, तब रेखा L2 वृत्त C की जीवा नहीं होगी।
View Solutionरेखा lx+my+n=0 वृत्त x2+y2=a2 की एक स्पर्श रेखा होगी यदि
View Solution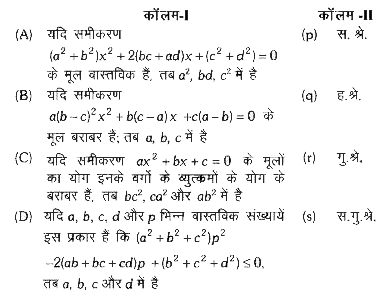
View Solution
View SolutionIf x=1−t21+t2 and y=2at1+t2, then dydx=
View SolutionLet g(x)=∫x0f(t)dy=t , where f is such that 12≤f(t)≤1 for t∈[0,1]and0≤f(t)≤12 for t∈[1,2]. then . g(2) satisfies the inequality
View Solutionयदि (If) cosx=1−t21+t2 तथा (and) siny=2t1+t2,0≤t≤1 सिद्ध करें कि (Prove that) d2ydx2, t से स्वतंत्र है |
View SolutionIf x=sin−1t,y=log(1−t2),0≤t<1, then the value of d2ydx2 at t=13 is -
View Solutionदर्शाइए की बिंदु x=2at1+t2 तथा y=a(1−t2)1+t2 जहाँ a स्थिर राशि है t प्राचल है तथा −1≤t≤1,t के प्रत्येक मान के लिए वृत्त पर स्थित है ।
View SolutionShow that the point (x,y) given y x=2at1+t2 and y=a(1−t2)1+t2 lies on a circle..
View SolutionIf x=2at1+t2,y=a(1−t2)1+t2 , where t is a parameter, then a is
View SolutionIf x=2at1+t2,y=a(1−t2)1+t2 , where t is a parameter, then a is
View SolutionLet g(x)=∫x0f(t)dt, where 12≤f(t)<1,t∈[0,1]and0<f(t)≤12fort∈[1,2]. Then :
View Solutionसमीकरणों x=2at1+t2,y=a(1−t2)1+t2(−1≤t≤1) से दत्त बिन्दु का बिन्दुपथ होगा
View Solution
