Completion type Question : A body is dropped from the top of a tower of height h and at the same time another body is projected from the foot of the tower, in the same vertical line, with a velocity which would be just sufficient to take it to the same height h. The two bodies will meet at a height ..................
To solve the problem, we need to analyze the motion of both bodies: one is dropped from the top of a tower and the other is projected upwards from the base of the tower.
1. Identify the Variables:
- Let the height of the tower be h.
- Let the height at which the two bodies meet be x (measured from the ground).
- The height from the top of the tower to the meeting point will then be h−x.
2. Determine the Initial Conditions:
- The body dropped from the top has an initial velocity u1=0 and falls under the influence of gravity.
- The body projected from the bottom has an initial velocity u2 which is sufficient to reach height h.
3. Calculate the Initial Velocity u2:
- To find u2, we can use the kinematic equation for the body projected upwards:
v2=u2−2gh
At the maximum height, the final velocity v=0:
0=u22−2gh⟹u22=2gh⟹u2=√2gh
4. Set Up the Equations of Motion:
- For the body dropped from the top:
h−x=12gt2(Equation 1)
- For the body projected from the bottom:
x=u2t−12gt2(Equation 2)
- Substitute u2=√2gh into Equation 2:
x=√2gh⋅t−12gt2
5. Combine the Equations:
- Add Equation 1 and Equation 2:
(h−x)+x=12gt2+(√2gh⋅t−12gt2)
Simplifying gives:
h=√2gh⋅t
- Rearranging gives:
t=h√2gh=h√2g⋅1√h=√h√2g
6. Substitute t Back to Find x:
- Substitute t into the equation for x:
x=√2gh⋅√h√2g−12g(√h√2g)2
- Simplifying gives:
x=√h2−12g⋅h2g=h−h4=3h4
Final Answer:
The two bodies will meet at a height of 3h4 from the ground.
---
- Let the height of the tower be h.
- Let the height at which the two bodies meet be x (measured from the ground).
- The height from the top of the tower to the meeting point will then be h−x.
- The body dropped from the top has an initial velocity u1=0 and falls under the influence of gravity.
- The body projected from the bottom has an initial velocity u2 which is sufficient to reach height h.
- To find u2, we can use the kinematic equation for the body projected upwards:
v2=u2−2gh
At the maximum height, the final velocity v=0:
0=u22−2gh⟹u22=2gh⟹u2=√2gh
- For the body dropped from the top:
h−x=12gt2(Equation 1)
- For the body projected from the bottom:
x=u2t−12gt2(Equation 2)
- Substitute u2=√2gh into Equation 2:
x=√2gh⋅t−12gt2
- Add Equation 1 and Equation 2:
(h−x)+x=12gt2+(√2gh⋅t−12gt2)
Simplifying gives:
h=√2gh⋅t
- Rearranging gives:
t=h√2gh=h√2g⋅1√h=√h√2g
- Substitute t into the equation for x:
x=√2gh⋅√h√2g−12g(√h√2g)2
- Simplifying gives:
x=√h2−12g⋅h2g=h−h4=3h4
The two bodies will meet at a height of 3h4 from the ground.
Topper's Solved these Questions
Similar Questions
A body is thrown horizontally from the top of a tower of height 5m. It touches the ground at a distance of 10m from the foot of the tower. Find the initial velocity of the body.
View SolutionA particle was dropped from the top of the tower and at the same time another body is thrown vertically upwards from the bottom of the tower with such a velocity that it can just reach the top of the tower. They will meet at a height .......... after .......... seconds.
View Solution
Knowledge Check
A body is released from the top of a tower of height h. It takes t sec to reach the ground. Where will be the ball after time t2 sec?
AAt h2 from the groundBAt h4 from the groundCdepends upon mass and volume of the bodyDat 3h4 from the groundA body dropped from the top of a tower covers 7/16 of the total height in the last second of its fall. The time of fall is
A2secB4secC1secD(507)secA: A body X is dropped from the top of a tower. At the same time, another body Y is thrown horizontally from the same position with a velocity u. Both bodies will reach the ground at the same time.
R: Horizontal velocity has no effect on motion in the vertical direction.AIf both A and B are true and R is the correct explanation of ABIf both A and R are true but R is not correct explantion of ACIf A is true but R is falseDIf A is false but R is true
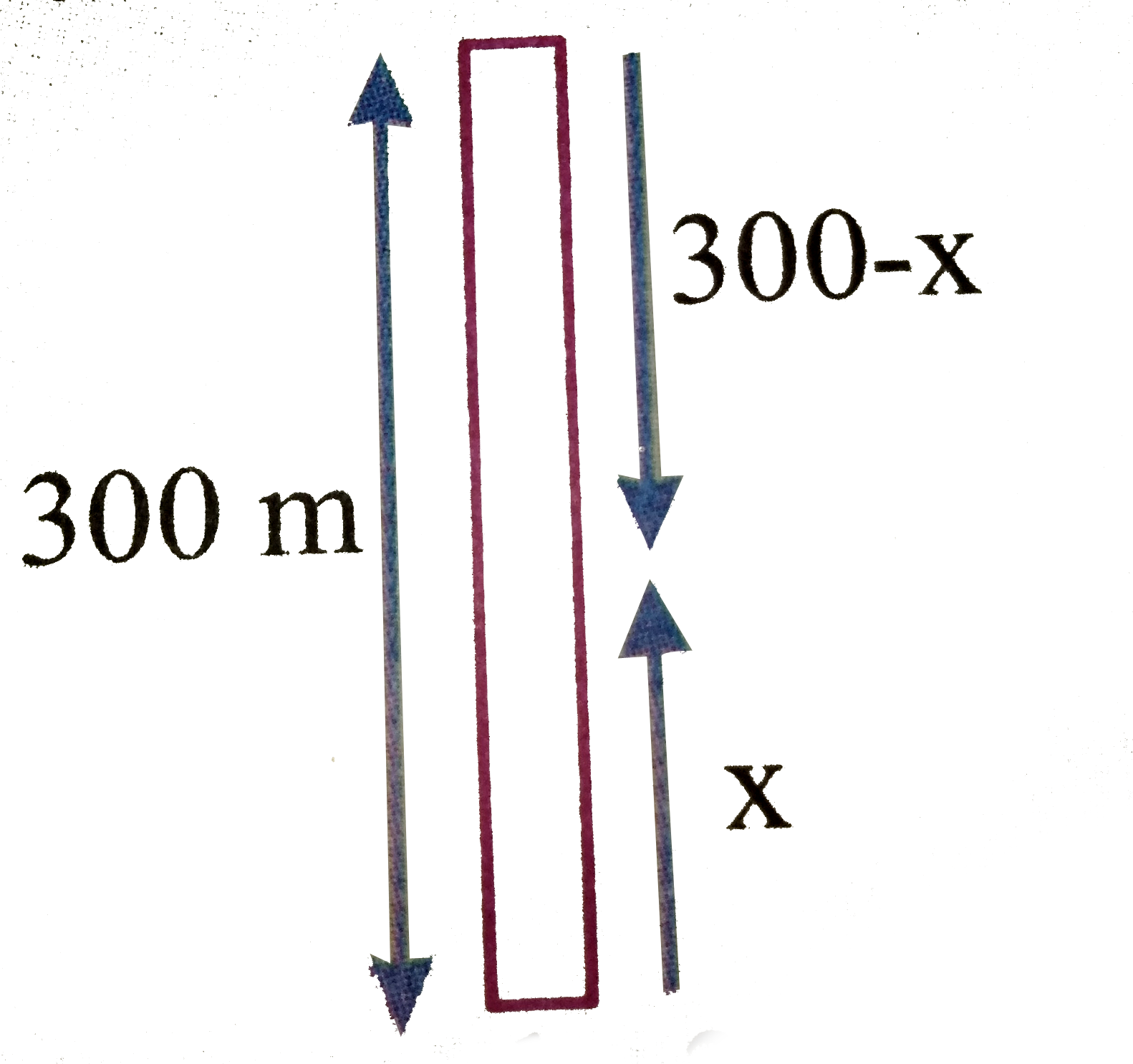
A stone is allowed to fall from the top of a tower 300 m height and at the same time another stone is projected vertically up from the ground with a velocity 100m/s. Find when and where the two stones meet?
View SolutionA body A is dropped vertically from the top of a tower. If another identical body B is thrown horizontally from the same point at the same instant, then
View SolutionA body is released from the top of a tower of height 50 m. Find the velocity with which the body hits the ground. (g=9.8m/s2)
View SolutionA ball is dropped from the top of a tower of height 100 m and another ball is thrown vertically upward at the same instant with a velocity of 10 m/s. Find the time after which the ball will be at same level.
View SolutionThe angle of elevation of the top of a tower from the point P and Q at distance of a and b respectively from the base of the tower and in the same straight line with it are complementary .The height of the tower is
View Solution
