State in the following cases, whether the motion is one, two or three dimensional motion (a) a kite flying on windy day (b) a speeding car on a long straight high way (c ) a carrom coin rebounding from the side if the board (d) a plane revolving around its star.
Step by step video solution for State in the following cases, whether the motion is one, two or three dimensional motion (a) a kite flying on windy day (b) a speeding car on a long straight high way (c ) a carrom coin rebounding from the side if the board (d) a plane revolving around its star. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
State in the following cases, whether the motion is one, two or three dimensional motion is one, two or three dimesional motion: (a) a dite fluing onwindy day (b) a speeding car on a long straight high way (c ) a carrom coin rebounding from the side if the board (d) a plane revolving around its star.
View SolutionGiven below are some examples of motion. State in each case. If the motion is one, two or three dimensions : (i) A flying bird in the sky(ii) A footballkicked by a playes (iii) Earth revolving around the sum (iv) The motion of the bob of a simple pendulum.
View Solution
Knowledge Check
The general motion of a rigid body can be considered to be a combination of (i) a motion of its centre of mass about an axis, and (ii) its motion about an instantaneous exis passing through the centre of mass. These axes need not be stationary. Consider, for example, a thin uniform disc welded (rigidly fixed) horizontally at its rim to a massless, stick as shown in the figure. When the disc-stick system is rotated about the origin on a horizontal frictionless plane with angular speed ω the motion at any instant can be taken as a combination of (i) a rotation of the disc through an instantaneous vertical axis passing through its centre of mass (as is seen from the changed orientation of points P and Q). Both these motions have the same angular speed ω in this case
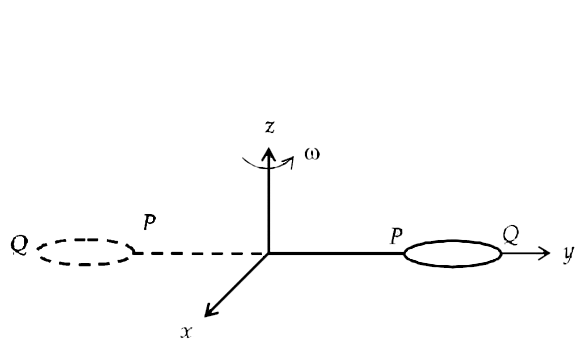
Now consider two similar system as shown in the figure: Case (a) the disc with its face vertical and parallel to x-z plane, Case (b) the disc with its face making an angle of 45∘ with x-y plane and its horizontal diameter parallel to x-axis. In both the cases, the disc is welded at point P, and the systems are rotated with constant angular speed ω about the z-axis.
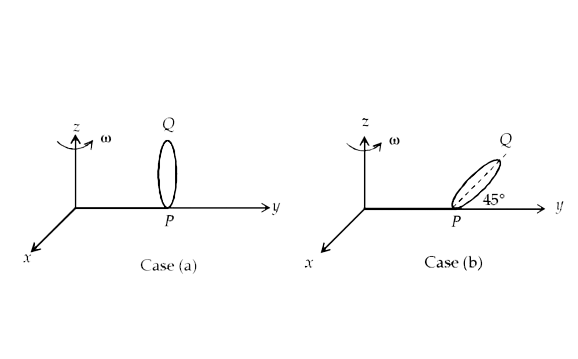
Which of the following statements regarding the angular speed about the instantaneous axis (passing through the centre of mass) is correct?Ait is √2ω for both the casesBit is ω for cases (a), and ω/√2 for case (b)Cit is ω for case (a), and √2ω for case (b)Dit is ω for both the cases.Arrange the following steps in sequential order to verify laws of reflection of light.
(A) Place a mirror on MM' and looking at the pins P and Q from the other side of the normal, observe their images.
(B) Fix a white paper to a drawing board and draw a straight line MM' and a line ON perpendicular to MM' on the paper.
(C) Fix two more pins P' and Q' in line with the images of the pins P and Q.
(D) Draw a line PO such that it makes suitable acute angle with the normal and fix two pins P and Q on the line, which is the incident ray.
(E) Measure ∠PON, the angle of incidence and ∠(P'ON), the angle of reflection.
(F) Remove the plane mirror and join P' and O. OP' is the reflected ray.
(G) Repeat the experiment for different angles of incidence and tabulate the results.AB A E C D F GBB D A C F E GCB A D C E F GDB D C E F A GQuestion Stimulus :-
DIRECTIONS: Read the following passage carefully and answer the questions given below it.
Long, long ago in a big forest, there were many trees, among the cluster of trees, there was a very tall pine tree. He was so tall that he could talk to the stars in the sky. He could easily gaze over the heads of the other trees. One day, late in the evening, the pine tree saw a little girl approaching him.
He could see her only because of his height. She was in tears. The pine tree bent as low as he could and asked the weeping girl. ‘What is the matter? Why are you crying?’ the little girl, still sobbing replied, ‘I was gathering flowers for the goddess Durga, Who I believe, would help my parents to overcome their poverty and I have lost my way’. The pine tree said to the little girl, ‘It is late in the evening. It will not be possible for you to return to your house, which is at the other end at the forest. Sleep for the night at this place.’ The pine tree pointed out to an open cavelike place under him. The little girl was frightened of wild animals.
The girl quickly crept into the cave-like place. The pine tree was happy and pleased with himself. He stood like a soldier guarding the place. The girl woke up in the morning and was amazed to see the pine tree standing like a guard outside the cave. Then her gaze travelled to the heap of flowers that she had gathered the previous night. The flowers lay withering on the ground. The pine tree understood what was going on in the girl’s mind. He wrapped his branches around the nearby flower trees and shook them gently. The little girl’s eyes brightened. But a great surprise awaited her. The pine tree brought out a bag of gold coins which had been lying for years in the hole in its trunk and gave it to the girl. With teary eyes she thanked her benefactor and went away.
Which word in the passage means ‘the state of being extremely poor’?AApproachBPovertyCOvercomeDBenefactor
Given below are some eamples of wave motion. State in each case, if the wave motino is transverse, longitudinal or a combination of both?
(i) Motion of a kink in a long coil spring produced by displacing one end of spring side ways.
(ii) Waves produed in a cylinder containing a liquid by moving its piston back and forth.
(iii) Waves produed by a motor boat sailing in water.
(iv) Light waves travelling from sun to earth.
(v) ultrasonic waves in air produced by a vibrating quartz crystal.
View SolutionA solid cylinder of mass 20kg and radius 0.12m rotating wit initial angula speed of 125rad/s is placed lightly (i.e. without any translational push) on a horizontal table with coefficient of kinetic friction μk=0.15 between the cylinder and the table.
(a) After how long does the cylinder start rolling ?
(b) What is the initial translational energy, rotational energy and total energy of the cylinder ?
(c) What is the final (i.e. after rolling begins) translational energy, rotational energy and total energy of the cylinder ? (d) Is the finl total enrgy equal to the initial total energy of motion of the cylinder ? If not, where does the difference of energy disappear ?
(e) Account for the loss of total energy of motion in the following way : find the work done by friction on the body its translational motion. Show that net work done by friction on the body is negative, equal in magnitude to the loss of total energy calculated in (d) above.
View SolutionState which of the following statements are true (T) and which false (F): Point has a size because we can see it as a thick dot on paper. By lines in geometry, we mean only straight lines. Two lines in a place always intersect in a point. Any plane through a vertical line is vertical. Any plane through a horizontal line is horizontal. There cannot be a horizontal line is a vertical plane. All lines in a horizontal plane are horizontal. Two lines in a plane always intersect in a point. If two lines intersect at a point P , then P is called the point of concurrence of the two lines. If two lines intersect at a point p , then p is called the point of intersection of the two lines. If A, B, C and D are collinear points D, P and Q are collinear, then points A, B, C, D, P and Q and always collinear. Two different lines can be drawn passing through two given points. Through a given point only one line can be drawn. Four points are collinear if any three of them lie on the same line. The maximum number of points of intersection of three lines in three. The minimum matching of the statements of column A and Column B .
View SolutionProjectile motion is a combination of two one-dimensional motion: one in horizontal and other in vertical direction. Motion in 2D means in a plane. Necessary condition for 2D motion is that the velocity vector is coplanar to the acceleration vector. In case of projectile motion, the angle between velocity and acceleration will be 0∘<θ<180∘. During the projectile motion, the horizontal component of velocity ramains unchanged but the vertical component of velocity is time dependent. Now answer the following questions:
A particle is projected from the origin in the x-y plane. The acceleration of particle in negative y-direction is α. If equation of path of the particle is y=ax−bx2, then initial velocity of the particle is
View SolutionProjectile motion is a combination of two one-dimensional motion: one in horizontal and other in vertical direction. Motion in 2D means in a plane. Necessary condition for 2D motion is that the velocity vector is coplanar to the acceleration vector. In case of projectile motion, the angle between velocity and acceleration will be 0∘ltθlt180∘. During the projectile motion, the horizontal component of velocity ramains unchanged but the vertical component of velocity is time dependent. Now answer the following questions:
An object is projected from origin in x-y plane in which velocity changes according to relation →v=aˆi+bxˆj. Path of particle is
View Solution
