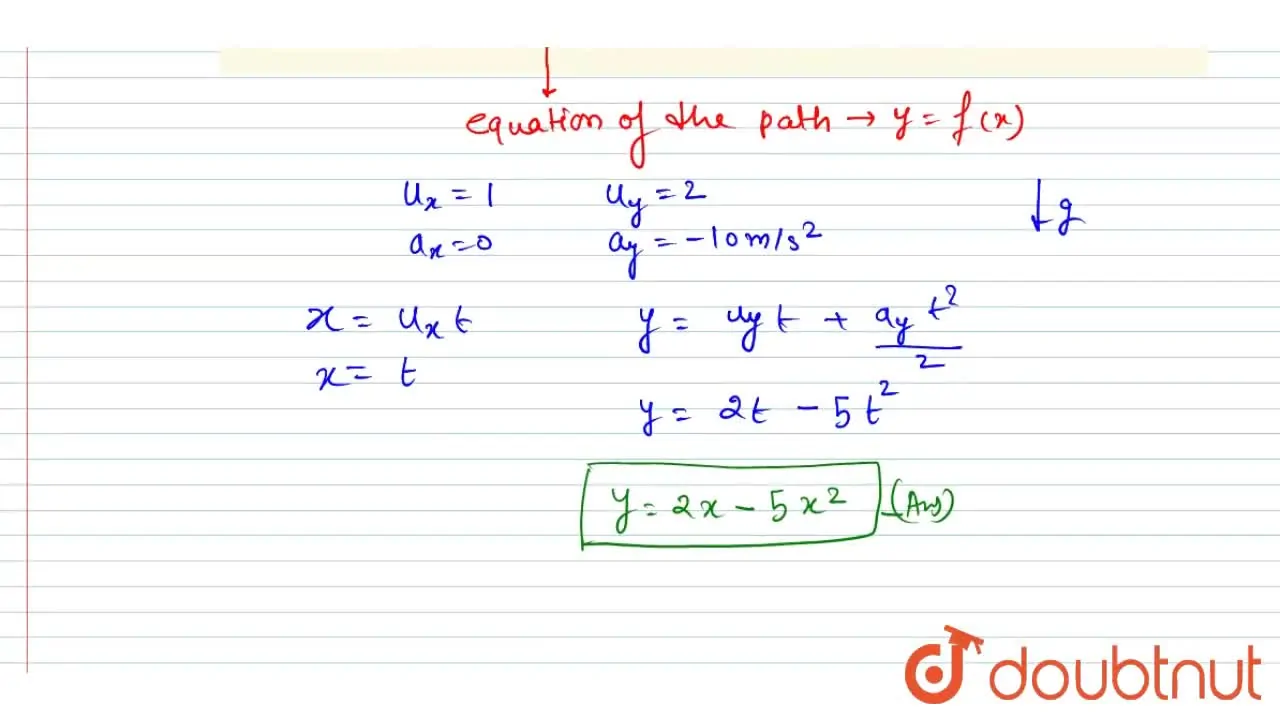
A projectile is given an initial velocity of (ˆi+2ˆj)m/s, where ˆi is along the ground and ˆj is along the vertical . If g=10m/s2, the equation of its trajectory is :
y=2x−5x2
4y=2x−5x2
4y=2x−25x2
y=x−5x2
The correct Answer is:A
Step by step video & image solution for A projectile is given an initial velocity of ( hat(i) + 2 hat (j) ) m//s, where hat(i) is along the ground and hat (j) is along the vertical . If g = 10 m//s^(2) , the equation of its trajectory is : by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
A projectile is given an initial velocity of (ˆi+2ˆj) The Cartesian equation of its path is (g=10ms−1) ( Here , ˆi is the unit vector along horizontal and ˆj is unit vector vertically upwards)
View SolutionA particle has an initial velocity of 3ˆi+4ˆj and an acceleration of 0.4ˆi+0.3ˆj. Its speed after 10s is :
View SolutionA particle has an initial velocity of 3ˆi+4ˆj and an acceleration of 0.4ˆi+0.3ˆj. Its speed after 10s is :
View SolutionA projectile is projected in air with initial velocity →v=(3ˆi+4ˆj) m/s from the origin. The equation of trajectory of the projectile is given as (g=−10ˆjms2) (neglect air resistance )
View SolutionA particle has initial velocity of (3ˆi+4ˆj)m/s and a acceleration of (4ˆi−3ˆj)m/s2. Its speed after one second will be equal to :-
View SolutionUnit vector along the vector ˆi+ˆj+ˆk is
View SolutionThe unit vector along →A=2ˆi+3ˆj is :
View SolutionThe component of vector A=2ˆi+3ˆj along the vector ˆi+ˆj is
View SolutionA particle has initial velocity (3ˆi+4ˆj) and has acceleration (0.4ˆi+0.3ˆj). Its speed after 10s is.
View SolutionA particle is moving in x-y plane. At an instant, it has velocity (4ˆi+4ˆj)m/s and acceleration (3ˆi+5ˆj)m/s2 At that instant, the radius of curvature of its path will be :
 View Solution
View Solution