AB is a vertical pole with B at the ground level and A at the top. A man finds that the angle of elevation of the point A from a certain point C on the ground is 60o . He moves away from the pole along the line BC to a point D such that CD=7m . From D the angle of elevation of the point A is 45o . Then the height of the pole is (1) 7√321√3−1m (2) 7√32√3+1m (3) 7√32√3−1m (4) 7√32√3+1m
To find the height of the vertical pole AB, we can use the information given about the angles of elevation from points C and D.
1. Define Variables:
- Let the height of the pole AB be H.
- Let the distance from point B (the base of the pole) to point C be BC=B.
- The distance from point C to point D is given as CD=7m.
2. Using the Angle of Elevation from Point C:
- From point C, the angle of elevation to point A is 60∘.
- Using the tangent function:
tan(60∘)=HB
- Since tan(60∘)=√3:
√3=HB⟹H=B√3(Equation 1)
3. Using the Angle of Elevation from Point D:
- From point D, the angle of elevation to point A is 45∘.
- The distance from point B to point D is BD=BC+CD=B+7.
- Using the tangent function:
tan(45∘)=HB+7
- Since tan(45∘)=1:
1=HB+7⟹H=B+7(Equation 2)
4. Equate the Two Expressions for H:
- From Equation 1 and Equation 2:
B√3=B+7
5. Rearranging the Equation:
- Rearranging gives:
B√3−B=7
- Factoring out B:
B(√3−1)=7
- Solving for B:
B=7√3−1
6. Substituting B back to find H:
- Substitute B back into Equation 1:
H=B√3=(7√3−1)√3
- Simplifying:
H=7√3√3−1
7. Rationalizing the Denominator:
- Multiply numerator and denominator by √3+1:
H=7√3(√3+1)(√3−1)(√3+1)=7√3(√3+1)3−1=7√3(√3+1)2
8. Final Result:
- Therefore, the height of the pole H is:
H=7√32(√3+1)m
Conclusion:
The height of the pole is 7√32(√3+1)m.
- Let the height of the pole AB be H.
- Let the distance from point B (the base of the pole) to point C be BC=B.
- The distance from point C to point D is given as CD=7m.
- From point C, the angle of elevation to point A is 60∘.
- Using the tangent function:
tan(60∘)=HB
- Since tan(60∘)=√3:
√3=HB⟹H=B√3(Equation 1)
- From point D, the angle of elevation to point A is 45∘.
- The distance from point B to point D is BD=BC+CD=B+7.
- Using the tangent function:
tan(45∘)=HB+7
- Since tan(45∘)=1:
1=HB+7⟹H=B+7(Equation 2)
- From Equation 1 and Equation 2:
B√3=B+7
- Rearranging gives:
B√3−B=7
- Factoring out B:
B(√3−1)=7
- Solving for B:
B=7√3−1
- Substitute B back into Equation 1:
H=B√3=(7√3−1)√3
- Simplifying:
H=7√3√3−1
- Multiply numerator and denominator by √3+1:
H=7√3(√3+1)(√3−1)(√3+1)=7√3(√3+1)3−1=7√3(√3+1)2
- Therefore, the height of the pole H is:
H=7√32(√3+1)m
The height of the pole is 7√32(√3+1)m.
Topper's Solved these Questions
Similar Questions
AB is a vertical pole with B at the ground level and and A at the top .A man finds that the angle of elevation of the point A from a certain point C on the ground is 60∘ . He moves away from the pole along the line BC to a point D such that CD = 7 m .From D . the angle of elevation of the point A is 45∘ .Find the height of the pole .
View SolutionThe angle of elevation of the top of a tower from a point on the ground is 60∘. which is 25 m away from the foot of the tower. Find the height of the tower
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower is 30∘ . Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View Solution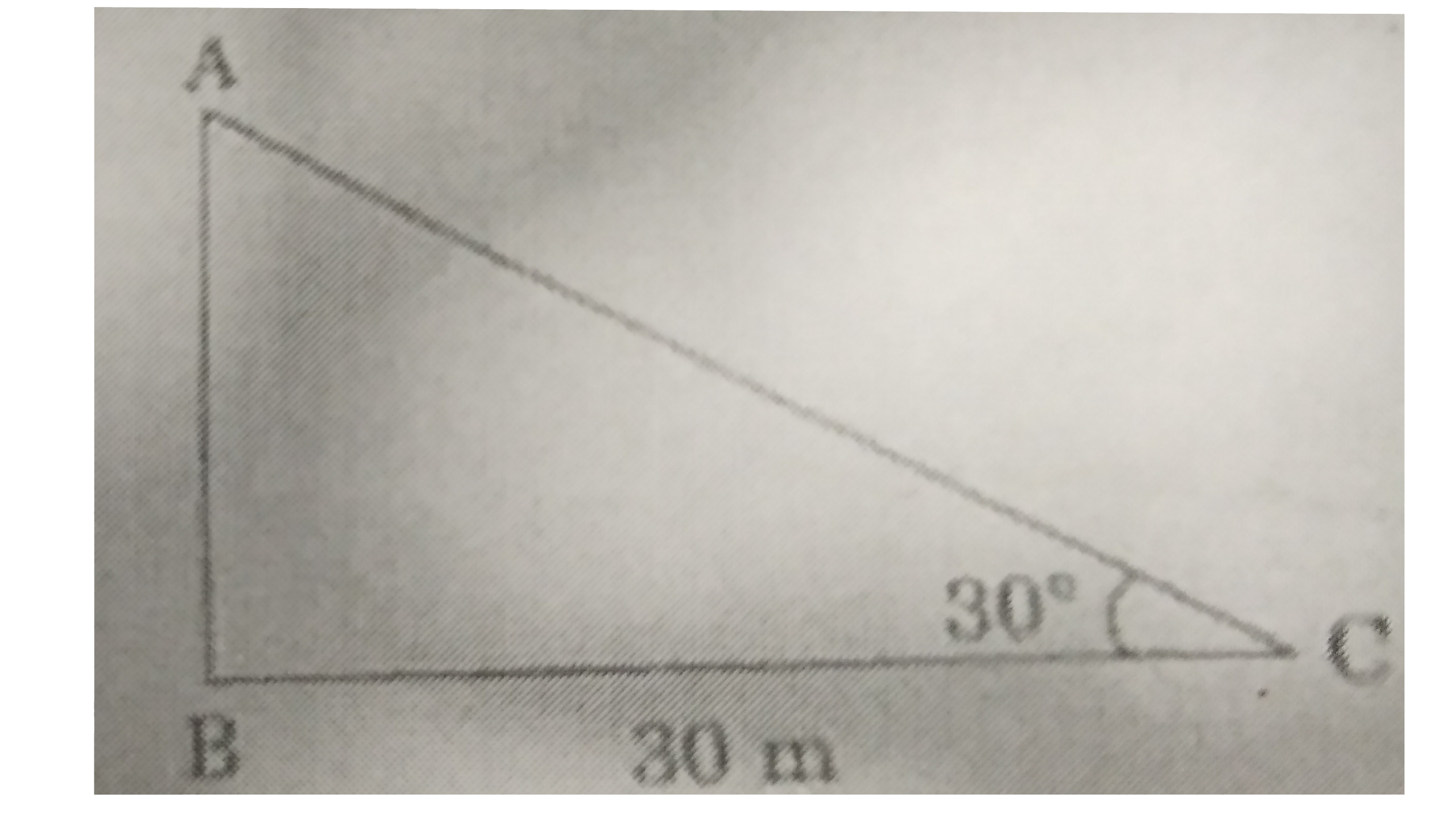
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.
View SolutionThe angle of elevation of the top of a tower at a point on the ground is 30∘ . What will be the angle of elevation, if the height of the tower is tripled?
View SolutionIn figure , the angle of elevation of the top of a tower AC from a point B on the ground is 60∘ . If the height of the tower is 20 m, Find the distance of the point from the foot of the power .
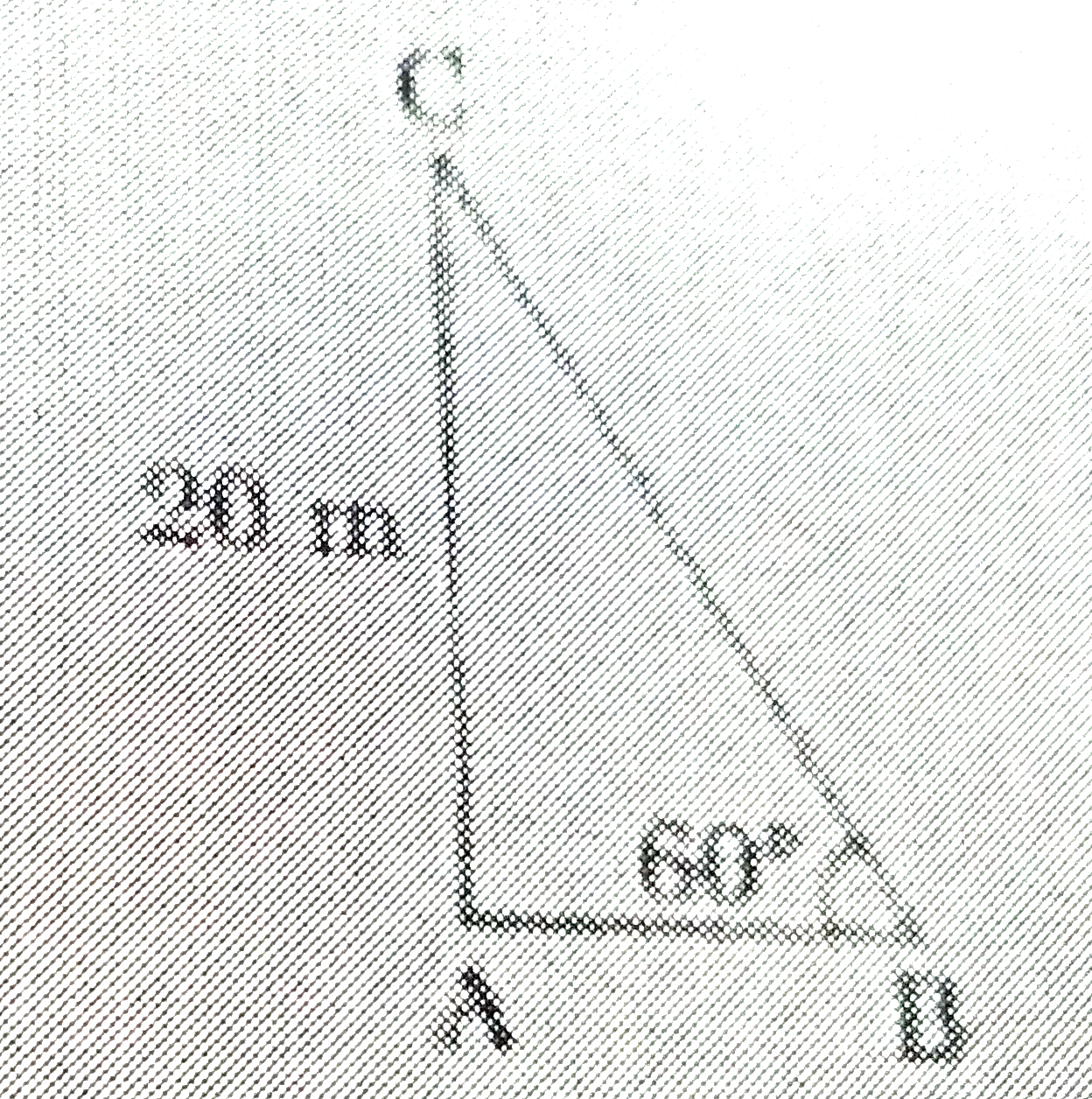
View Solution
