ΔH<ΔU for the reaction(s) :
N2(g)+3H2(g)→2NH3(g)
Ag2O(s)→2Ag(s)+12O2(g)
CO(g)+12O2(g)→CO2(g)
C(s)+O2(g)→CO2(g)
The correct Answer is:A, C
To determine for which reactions ΔH<ΔU, we will use the relationship between enthalpy change (ΔH) and internal energy change (ΔU) given by the equation:
ΔH=ΔU+ΔNgRT
Where:
- ΔNg = change in the number of gaseous moles (moles of products - moles of reactants)
- R = gas constant
- T = temperature
From this equation, we can infer that ΔH<ΔU when ΔNg is negative. This is because if ΔNg is negative, it will subtract from ΔU, making ΔH less than ΔU.
1. Analyze the Reactions: We will analyze each reaction to determine ΔNg.
2. First Reaction: N2+3H2→2NH3
- Moles of gaseous products = 2 (from 2NH3)
- Moles of gaseous reactants = 4 (from N2+3H2)
- ΔNg=2−4=−2
- Since ΔNg is negative, ΔH<ΔU.
3. Second Reaction: Ag2O(s)→2Ag(s)+12O2(g)
- Moles of gaseous products = 12 (from 12O2)
- Moles of gaseous reactants = 0 (no gaseous reactants)
- ΔNg=12−0=12
- Since ΔNg is positive, ΔH>ΔU.
4. Third Reaction: CO(g)+12O2(g)→CO2(g)
- Moles of gaseous products = 1 (from CO2)
- Moles of gaseous reactants = 1+12=32
- ΔNg=1−32=−12
- Since ΔNg is negative, ΔH<ΔU.
5. Fourth Reaction: C(s)+O2(g)→CO2(g)
- Moles of gaseous products = 1 (from CO2)
- Moles of gaseous reactants = 1 (from O2)
- ΔNg=1−1=0
- Since ΔNg is zero, ΔH=ΔU.
Conclusion:
From the analysis, we find that ΔH<ΔU for the first and third reactions. Therefore, the correct options are:
- Option A: N2+3H2→2NH3
- Option C: CO(g)+12O2(g)→CO2(g)
- ΔNg = change in the number of gaseous moles (moles of products - moles of reactants)
- R = gas constant
- T = temperature
- Moles of gaseous products = 2 (from 2NH3)
- Moles of gaseous reactants = 4 (from N2+3H2)
- ΔNg=2−4=−2
- Since ΔNg is negative, ΔH<ΔU.
- Moles of gaseous products = 12 (from 12O2)
- Moles of gaseous reactants = 0 (no gaseous reactants)
- ΔNg=12−0=12
- Since ΔNg is positive, ΔH>ΔU.
- Moles of gaseous products = 1 (from CO2)
- Moles of gaseous reactants = 1+12=32
- ΔNg=1−32=−12
- Since ΔNg is negative, ΔH<ΔU.
- Moles of gaseous products = 1 (from CO2)
- Moles of gaseous reactants = 1 (from O2)
- ΔNg=1−1=0
- Since ΔNg is zero, ΔH=ΔU.
From the analysis, we find that ΔH<ΔU for the first and third reactions. Therefore, the correct options are:
- Option C: CO(g)+12O2(g)→CO2(g)
Topper's Solved these Questions
Similar Questions
Knowledge Check
Rate of SN1 reaction is :
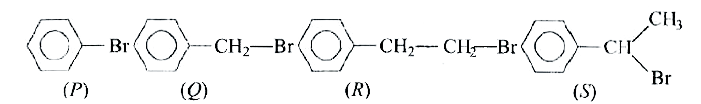 AS>Q>R>PBS>R>P>QCP>Q>R>SDS>R>Q>P
AS>Q>R>PBS>R>P>QCP>Q>R>SDS>R>Q>PRate of SN2 reaction is :
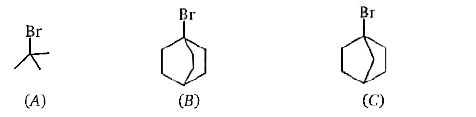 A(B)>(A)>(C)B(C)>(A)>(B)C(A)>(B)>(C)D(A)>(C)>(B)
A(B)>(A)>(C)B(C)>(A)>(B)C(A)>(B)>(C)D(A)>(C)>(B)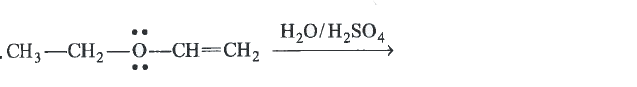
The product/s formed in the reaction is/are :ACH3−CH2−O−CH2−CH2−OHBCH3−CH2−O−OH∣CH−CH3CCH3−CH2−OHandHO−CH=CH2DCH3CH2−OHandCH3−O∣∣C−H
Equation(s) for the reaction(s) to prepare lead sulphate from lead carbonate.
View SolutionThe heat change for the reaction
C(s)+2S(s)→CS2(l) is called the heat of
View SolutionΔS∘ will be highest for the reaction
View SolutionAfter completion of the reactions (I and II) , the organic compounds (s) in the reaction mixtures is :
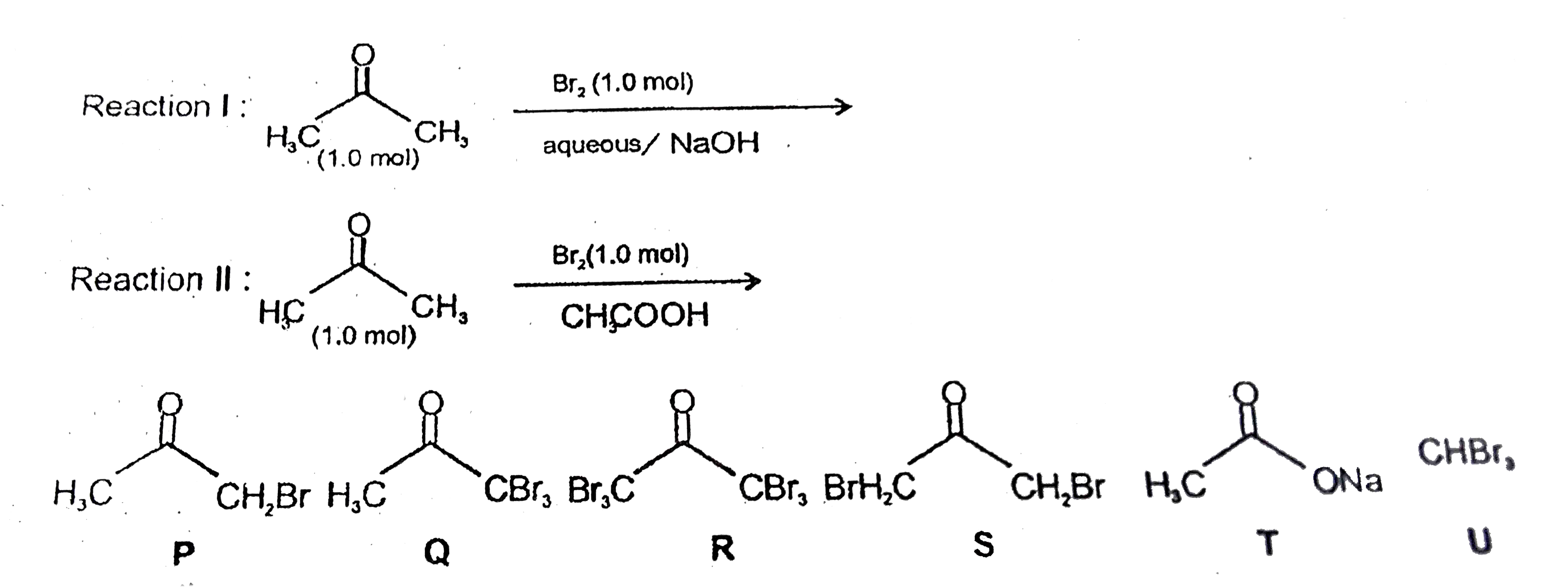
View SolutionWhich statement(s) is/are correct for the given reaction and compounds.
View Solution
