A particle is kept at rest at the origin. A constant force →F starts acting on it at t=0. Find the speed of the particle at time t.
To find the speed of a particle at time t when a constant force →F is applied to it, we can follow these steps:
1. Understanding the Problem:
- A particle is initially at rest at the origin (position = 0).
- A constant force →F starts acting on it at t=0.
- We need to find the speed of the particle at a time t.
2. Using Newton's Second Law:
- According to Newton's second law, the rate of change of momentum dpdt is equal to the applied force →F.
- Since the force is constant, we can write:
dpdt=F
3. Integrating the Equation:
- We can integrate both sides with respect to time:
dp=Fdt
- Integrating from the initial momentum (which is 0 since the particle is at rest) to the final momentum p at time t:
∫p0dp=∫t0Fdt
- This gives us:
p=Ft
4. Relating Momentum to Velocity:
- The momentum p of the particle is also related to its velocity v and mass m by the equation:
p=γm0v
- Here, γ is the Lorentz factor given by:
γ=1√1−v2c2
- m0 is the rest mass of the particle.
5. Setting the Equations Equal:
- From the previous steps, we have:
Ft=γm0v
- Substituting γ:
Ft=m0v√1−v2c2
6. Rearranging the Equation:
- Rearranging gives:
Ft√1−v2c2=m0v
- Squaring both sides leads to:
F2t2(1−v2c2)=m20v2
7. Expanding and Rearranging:
- Expanding the left side:
F2t2−F2t2v2c2=m20v2
- Rearranging gives:
F2t2=m20v2+F2t2v2c2
- Factoring out v2:
F2t2=v2(m20+F2t2c2)
8. Solving for Velocity:
- Finally, we can solve for v2:
v2=F2t2m20+F2t2c2
- Taking the square root gives:
v=Ft√m20+F2t2c2
Final Result:
The speed of the particle at time t is:
v=Ft√m20+F2t2c2
- A particle is initially at rest at the origin (position = 0).
- A constant force →F starts acting on it at t=0.
- We need to find the speed of the particle at a time t.
- According to Newton's second law, the rate of change of momentum dpdt is equal to the applied force →F.
- Since the force is constant, we can write:
dpdt=F
- We can integrate both sides with respect to time:
dp=Fdt
- Integrating from the initial momentum (which is 0 since the particle is at rest) to the final momentum p at time t:
∫p0dp=∫t0Fdt
- This gives us:
p=Ft
- The momentum p of the particle is also related to its velocity v and mass m by the equation:
p=γm0v
- Here, γ is the Lorentz factor given by:
γ=1√1−v2c2
- m0 is the rest mass of the particle.
- From the previous steps, we have:
Ft=γm0v
- Substituting γ:
Ft=m0v√1−v2c2
- Rearranging gives:
Ft√1−v2c2=m0v
- Squaring both sides leads to:
F2t2(1−v2c2)=m20v2
- Expanding the left side:
F2t2−F2t2v2c2=m20v2
- Rearranging gives:
F2t2=m20v2+F2t2v2c2
- Factoring out v2:
F2t2=v2(m20+F2t2c2)
- Finally, we can solve for v2:
v2=F2t2m20+F2t2c2
- Taking the square root gives:
v=Ft√m20+F2t2c2
The speed of the particle at time t is:
v=Ft√m20+F2t2c2
Topper's Solved these Questions
- THE SPECIAL THEORY OF RELATIVITYBOOK - HC VERMA ENGLISHCHAPTER - THE SPECIAL THEORY OF RELATIVITYEXERCISE - worked out example
8 Videos
- THE SPECIAL THEORY OF RELATIVITYBOOK - HC VERMA ENGLISHCHAPTER - THE SPECIAL THEORY OF RELATIVITYEXERCISE - Objective
2 Videos
- THE NUCLEOUSBOOK - HC VERMA ENGLISHCHAPTER - THE NUCLEOUSEXERCISE - Short answer
12 Videos
- THERMAL AND CHEMICAL EFFECT OF ELECTRIC CURRENTBOOK - HC VERMA ENGLISHCHAPTER - THERMAL AND CHEMICAL EFFECT OF ELECTRIC CURRENTEXERCISE - Short Question
9 Videos
Similar Questions
Two particles are shown in figure. At t=0 a constant force F=6N starts acting on 3kg. Find the velocity of circle of mass of these particle at t=5s.
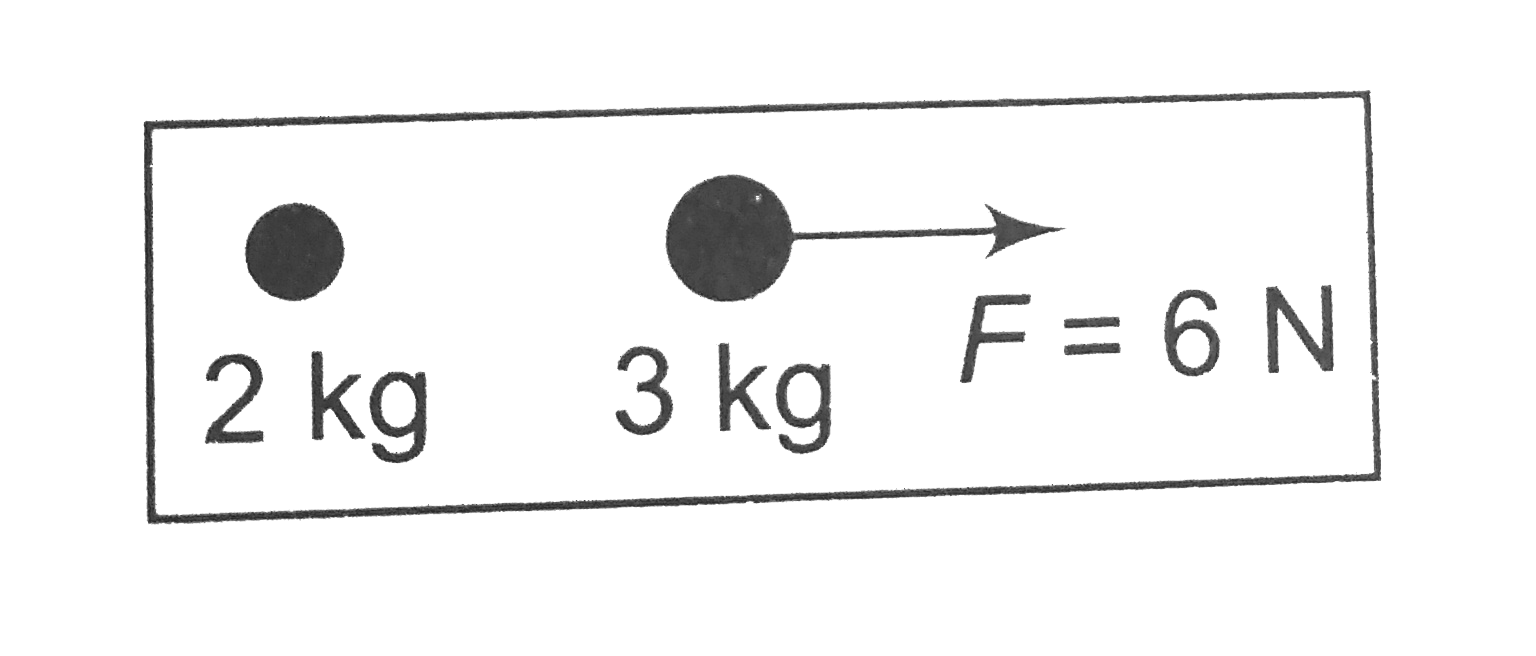 .
.
View SolutionThe acceleration a of a particle starting from rest varies with time according to relation, a=αt+β. Find the velocity of the particle at time instant t.
Strategy : a=dvdt
View SolutionForce acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K/V K is constant then speed of the particle in time t
View SolutionA particle starts from rest at time t=0 and moves on a straight line with acceleration as plotted in fig. The speed of the particle will be maximum after time.

View SolutionA particle starts motion from rest and moves along a straight line. Its velocity-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View SolutionA particle is resting over a smooth horizontal floor. At t=0, a horizontal force starts acting on it. Magnitude of the force increases with time according to law F=αt, Where alpha is a positive constant. From figure, which of the following statements are correct ?
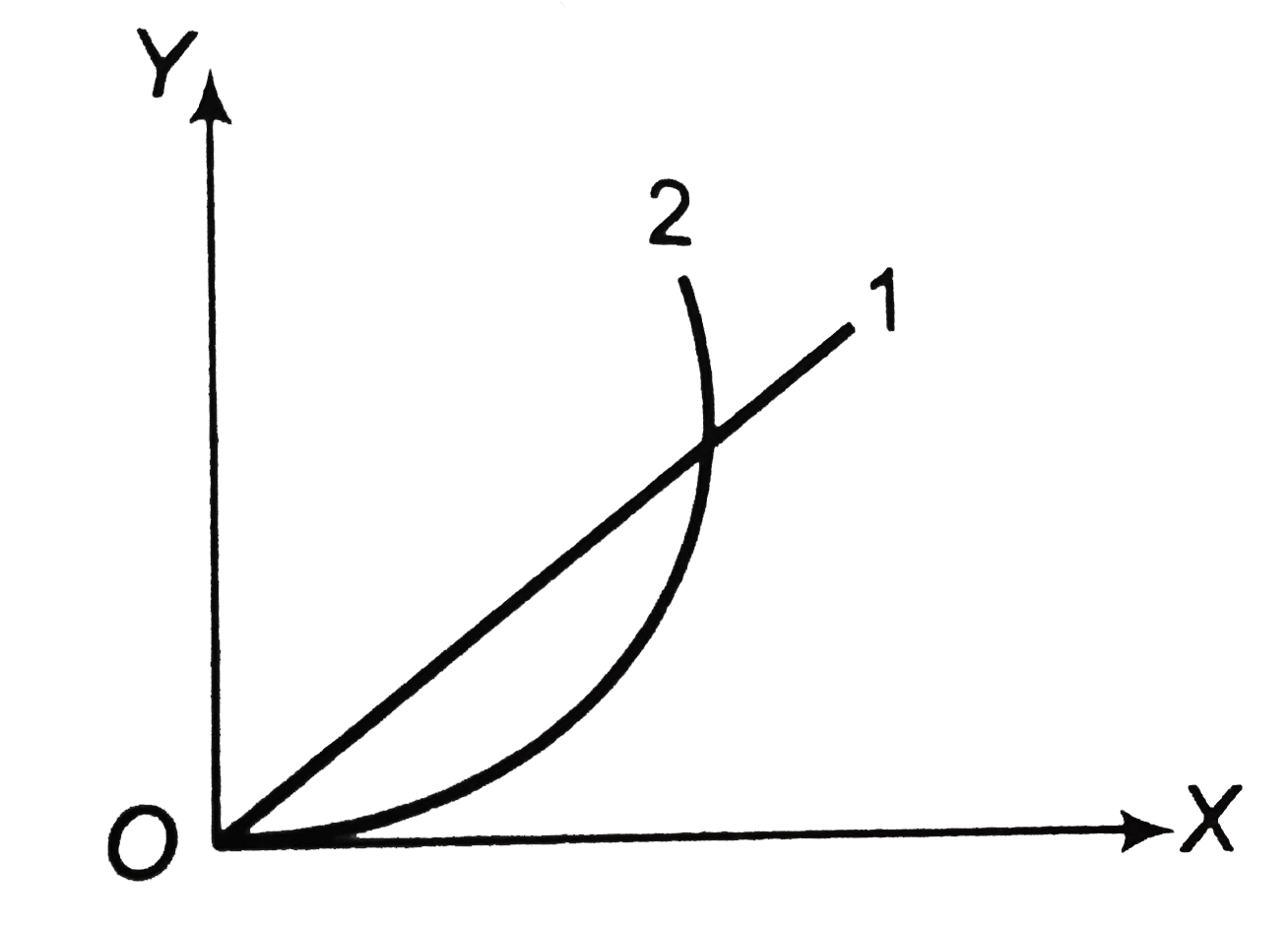
View SolutionA particle of mass m initially moving with speed v.A force acts on the particle f=kx where x is the distance travelled by the particle and k is constant. Find the speed of the particle when the work done by the force equals W.
View SolutionA particle moves rectilinearly with initial velocity u and constant acceleration a. Find the average velocity of the particle in a time interval from t=0 to t=t second of its motion.
View SolutionA graph of acceleration versus time of a particle starting from rest at t=0 is as shown in figure. The speed of the particle at t=14s is
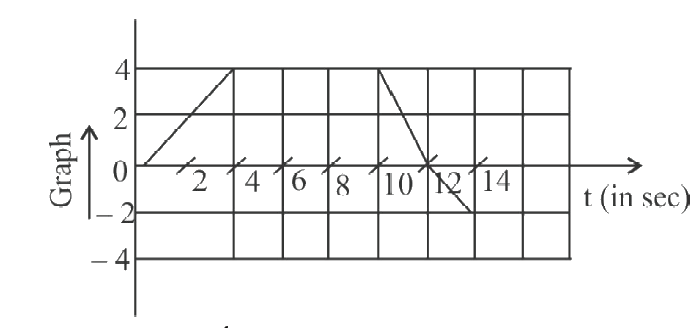
View SolutionA particle starts motion from rest and moves along a straight line. Its acceleration-time graph is shown. Find out speed of particle at t=2s and at t=3s.

View Solution
