Find the binding energy per nucleon of 79^197Au if its atomic mass is 196.96 u.
The correct Answer is:D
To find the binding energy per nucleon of 19779Au (Gold), we will follow these steps:
Step 1: Identify the number of protons and neutrons
The atomic number Z of Gold (Au) is 79, which means it has 79 protons. The mass number A is 197, which is the total number of protons and neutrons.
To find the number of neutrons N:
N=A−Z=197−79=118
Step 2: Calculate the mass of the nucleus
The mass of the nucleus can be calculated using the formula:
m=Z⋅mp+N⋅mn
where:
- mp is the mass of a proton (approximately 1.007276u),
- mn is the mass of a neutron (approximately 1.008665u).
Substituting the values:
m=79⋅1.007276+118⋅1.008665
Calculating each term:
m=79⋅1.007276=79.574784u
m=118⋅1.008665=118.02087u
Now adding these together:
m=79.574784+118.02087=197.595654u
Step 3: Calculate the binding energy
The binding energy BE can be calculated using the formula:
BE=(Z⋅mp+N⋅mn−m)⋅c2
We will convert the binding energy to MeV using the conversion factor 1u≈931.5MeV/c2.
Substituting the values:
BE=(197.595654−196.96)⋅931.5MeV
Calculating the difference:
BE=(197.595654−196.96)=0.635654u
Now converting to MeV:
BE=0.635654⋅931.5≈592.3MeV
Step 4: Calculate the binding energy per nucleon
The binding energy per nucleon BE/A is calculated by dividing the total binding energy by the mass number A:
BE/A=BEA=592.3MeV197
Calculating this gives:
BE/A≈3.0MeV/nucleon
Final Answer
The binding energy per nucleon of 19779Au is approximately 3.0MeV/nucleon.
---
The atomic number Z of Gold (Au) is 79, which means it has 79 protons. The mass number A is 197, which is the total number of protons and neutrons.
N=A−Z=197−79=118
The mass of the nucleus can be calculated using the formula:
m=Z⋅mp+N⋅mn
where:
- mp is the mass of a proton (approximately 1.007276u),
- mn is the mass of a neutron (approximately 1.008665u).
m=79⋅1.007276+118⋅1.008665
Calculating each term:
m=79⋅1.007276=79.574784u
m=118⋅1.008665=118.02087u
Now adding these together:
m=79.574784+118.02087=197.595654u
The binding energy BE can be calculated using the formula:
BE=(Z⋅mp+N⋅mn−m)⋅c2
We will convert the binding energy to MeV using the conversion factor 1u≈931.5MeV/c2.
BE=(197.595654−196.96)⋅931.5MeV
Calculating the difference:
BE=(197.595654−196.96)=0.635654u
Now converting to MeV:
BE=0.635654⋅931.5≈592.3MeV
The binding energy per nucleon BE/A is calculated by dividing the total binding energy by the mass number A:
BE/A=BEA=592.3MeV197
Calculating this gives:
BE/A≈3.0MeV/nucleon
The binding energy per nucleon of 19779Au is approximately 3.0MeV/nucleon.
Topper's Solved these Questions
- THE NUCLEOUSBook:HC VERMA ENGLISHChapter:THE NUCLEOUSExercise:Short answer
Explore 12 Videos
- THE NUCLEOUSBook:HC VERMA ENGLISHChapter:THE NUCLEOUSExercise:Objective 2
Explore 10 Videos
- SPEED OF LIGHTBook:HC VERMA ENGLISHChapter:SPEED OF LIGHTExercise:Question for short Answer
Explore 5 Videos
- THE SPECIAL THEORY OF RELATIVITYBook:HC VERMA ENGLISHChapter:THE SPECIAL THEORY OF RELATIVITYExercise:Short answer
Explore 2 Videos
Similar Questions
The value of binding energy per nucleon is
View SolutionThe binding energy per nucleon is maximum in the case of.
View SolutionThe binding energy per nucleon is maximum in the case of.
View SolutionAssertion The binding energy per nucleon, for nuclei with atomic mass number A>100, decreases with A.
Reason The nuclear forces are weak for heavier nuclei.
View SolutionCalculate the average binding energy per nucleon of .9341Nb having mass 9.2.906 u..
View SolutionFind the average binding energy per nucleon of .7N14 and .8O16. Their atomic masses are 14.008 u and 16.000 u. The mass of .1H1atom is 1.007825 u and the mass of neutron is 1.008665 u. Which is more stable?
View SolutionDraw the graph showing thervariation of binding energy per nucleon with mass number. Give therason for the decrease of binding energy per nucleon for nuclei with high mass numbers.
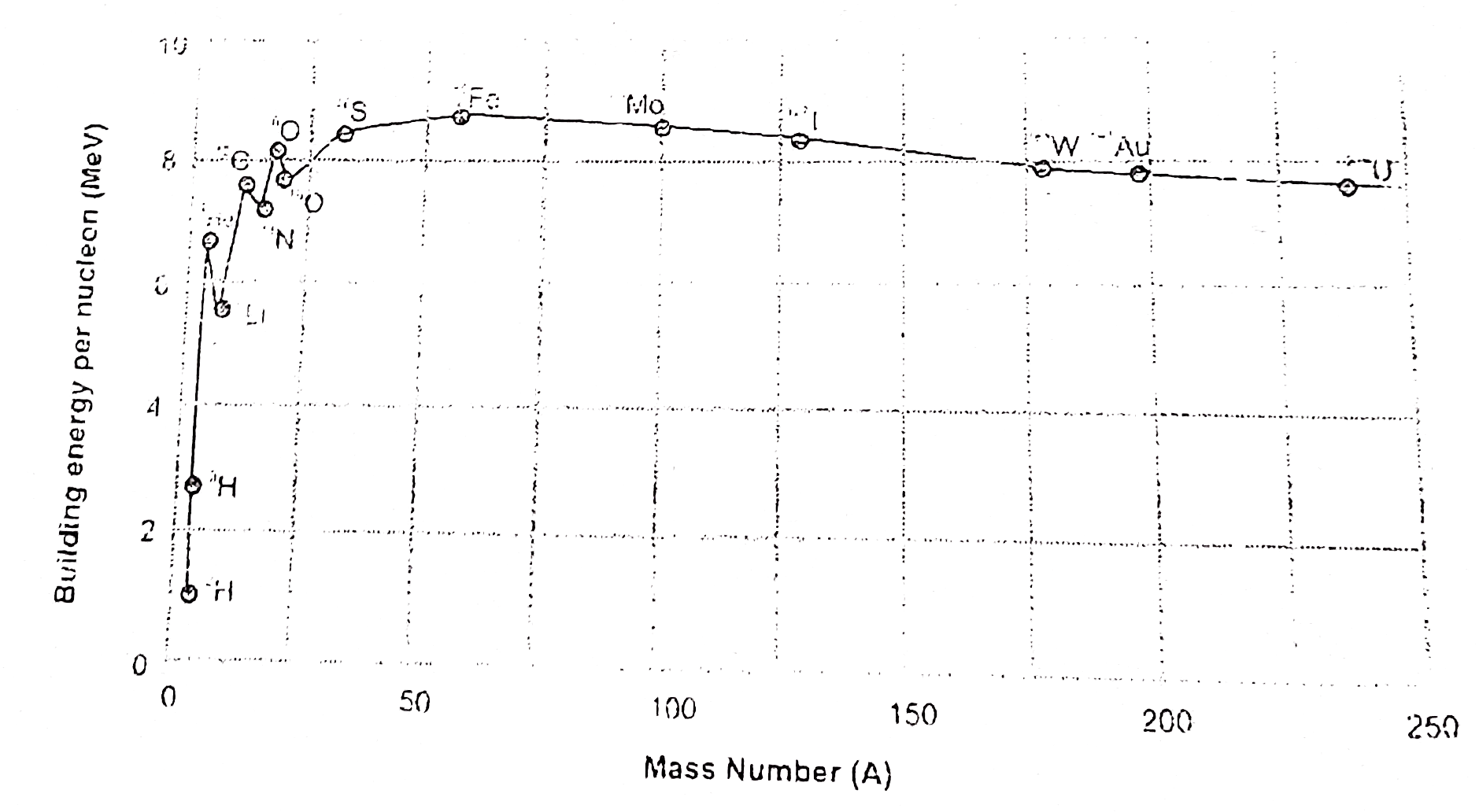
The binding energy per nucleon for nuclei with high mass number is low due to the large coulomb repulsion between the protons inside these nuclei.
View SolutionThe graph between the binding energy per nucleon (E) and atomic mass number (A) is as-
View SolutionAverage binding energy per nucleon over a wide range is
View SolutionCalculate the binding energy per nucleon of Li isotope, which has the isotopic mass of 7.016amu. The individual masses of neutron and proton are 1.008665amu and 1.007277amu, respectively and the mass of electron =0.000548amu.
View Solution