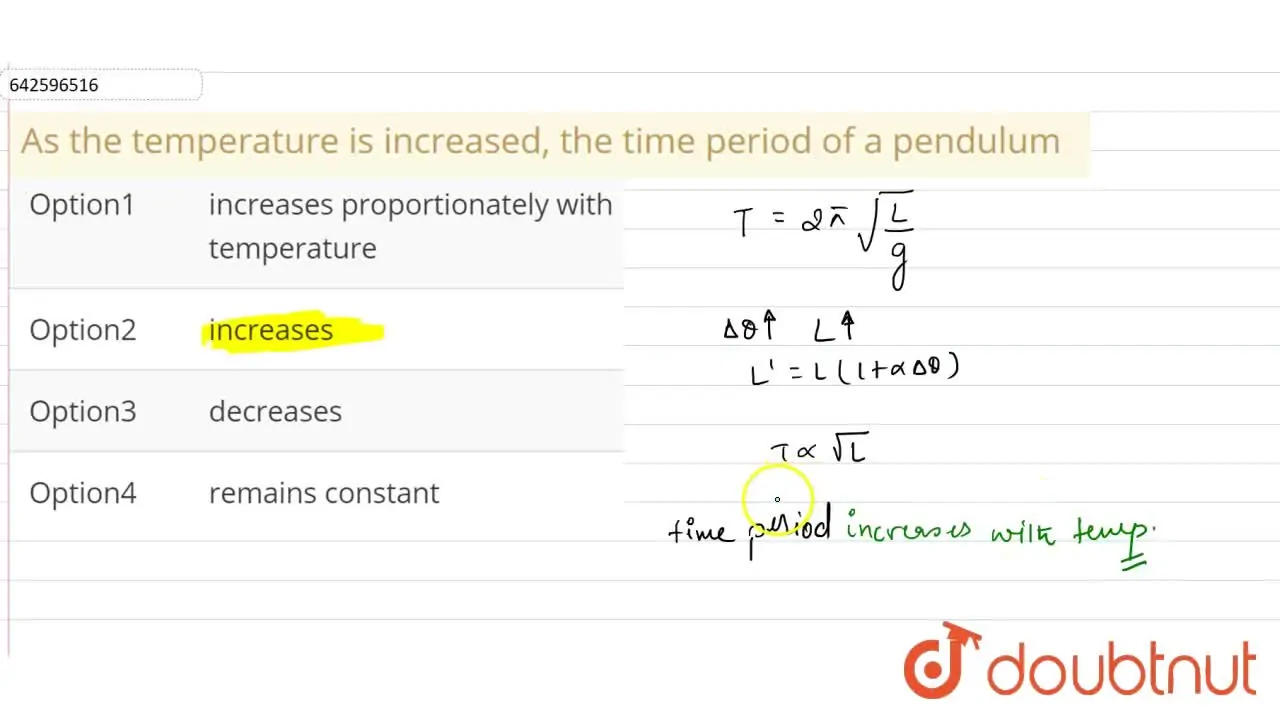
As the temperature is increased, the time period of a pendulum
increases proportionately with temperature
increases
decreases
remains constant
Step by step video & image solution for As the temperature is increased, the time period of a pendulum by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT AND TEMPERATUREEXERCISE - Question for Shor Answer13videos
HEAT AND TEMPERATURE
- BOOK - HC VERMA ENGLISHCHAPTER - GRAVITATIONEXERCISE - Question for short Answers18videos
GRAVITATION
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - QUESTIONS FOR SHORT ANSWER11videos
HEAT TRANSFER
Similar Questions
As the temperature is increased, the period of a pendulum
View SolutionAs the temperature is increased, the period of a pendulum
View SolutionThe time period of a pendulum clock is :
View SolutionIf length of a simple pendulum is increased by 69%, then the percentage increase in its time period is
View SolutionA pendulum suspended from the ceiling of the train has a time period of two seconds when the train is at rest, then the time period of the pendulum, if the train accelerates 10 m/s2 will be (g=10m/s2)
View SolutionThe time period of a seconds' pendulum :
View SolutionTime period of a particle in shm depends on the force constant k and mass m of the particle T=2π√mk A simple pendulum executes shm approximately. Why then is the time period of a pendulum independent of a mass of the pendulum?
View SolutionThe matallic bob of a simple pendulum has the relative density ρ. The time period of this pendulum is T it the metallic bob is immersed in water the new time period is given by
View SolutionThe matallic bob of a simple pendulum has the relative density ρ. The time period of this pendulum is T it the metallic bob is immersed in water the new time period is given by
View SolutionAssertion : The time-period of pendulu, on a satellite orbiting the earth is infinity .
Reason : Time-period of a pendulum is inversely proportional to √g .View Solution