A cylindrical object of outer diameter 10 cm, height 20 cm and density 8000 kgm−3 is supported by a vertical spring and is half dipped in water as shown in figure. a. Find the elongation of the spring in equilibrium condition. b. If the object is slightly depressed and released, find the time period of resulting oscillations of the object. The spring constant =500Nm−1.

The correct Answer is:A, B, C
d=10cm
r=5cm
→h=20cm
ρb=8000kgm3=8gm
k=500Nm=500×x103dy≠cm
a. Here F+U=mg[wheeFkx]
→kx+Vρug=mg
→500×103×(x)+(πr2)×(h2)×1×1000
=πr2xh×ρb×1000<br>rarr 500xx10^3xx(x)br>=pir^2xxhxx1000(rho-1/2)<br>=pixx(5)^2xx20xx1000(rho_b-1/2)<br>rarr 50x=pixx25xx2xx(rho_b-1/2)<br>=x=pi(8-0.5)<br>orx=pixx7.5=23.5cm<br>b.IfX→displacementoftheblockformtheequilibriumposition,<br>Driv∈gforce<br>F=kX+Vrho_wxxg<br>rarr ma=kX+pir^2xx(X)xxrho_wxxg<br>=(k+pir^2xxrho_wxxg)X<br>rarr omega^2xx(X)=((k+pir^2+rho_wxxg))/m xx(X)<br>[because a=w^2XinSHM]<br>rarr T=2i m/(k+pir^2+rho_wxxg) ,<br>=2pi(pixx25xx20xx8)/(500+10^3+pix25xx1xx1000)<br>=0.935s.
Topper's Solved these Questions
Similar Questions
A cylindrical object of outer diameter 20 cm and mass 2 kg floats in water with its axis vertical. If it is slightly depressed and then released, find the time period of the resulting simple harmonic motion of the object.
View SolutionFind the elastic potential energy stored in each spring shown in figure, when the block is in equilibrium. Also find the time period of vertical oscillation of the block.

View SolutionA particle of mass m is attached with three springs A,B and C of equal force constancts k as shown in figure. The particle is pushed slightly against the spring C and released. Find the time period of oscillation.
 .
.
View SolutionA wooden block of mass 0.5 kg and density 800 kgm−3 is fastened to the free end of a vertical spring of spring constant 50 Nm−1 fixed at the bottom. If the entire system is completely immersed in water, find a. the elongation (or compression) of the spring in equilibrium and b. the time period of vertical oscillations of the block when it is slightly depressed and released.
View SolutionA particle of mass m is attached with three springs A,B and C of equal force constancts k as shown in figure. The particle is pushed slightly against the spring C and released. Find the time period of oscillation.
 .
.
View SolutionA rectangular tank having base 15cm×20cm is filled with water (density ρ=1000kg/m3) up to 20cm and force constant K=280N/m is fixed to the bottom of the tank so that the spring remains vertical.
This system is in an elevator moving downwards with acceleration a=2m/s2. A cubical block of side l=10cm and mass m=2kg is gently placed over the spring and released gradually, as shown in the figure.
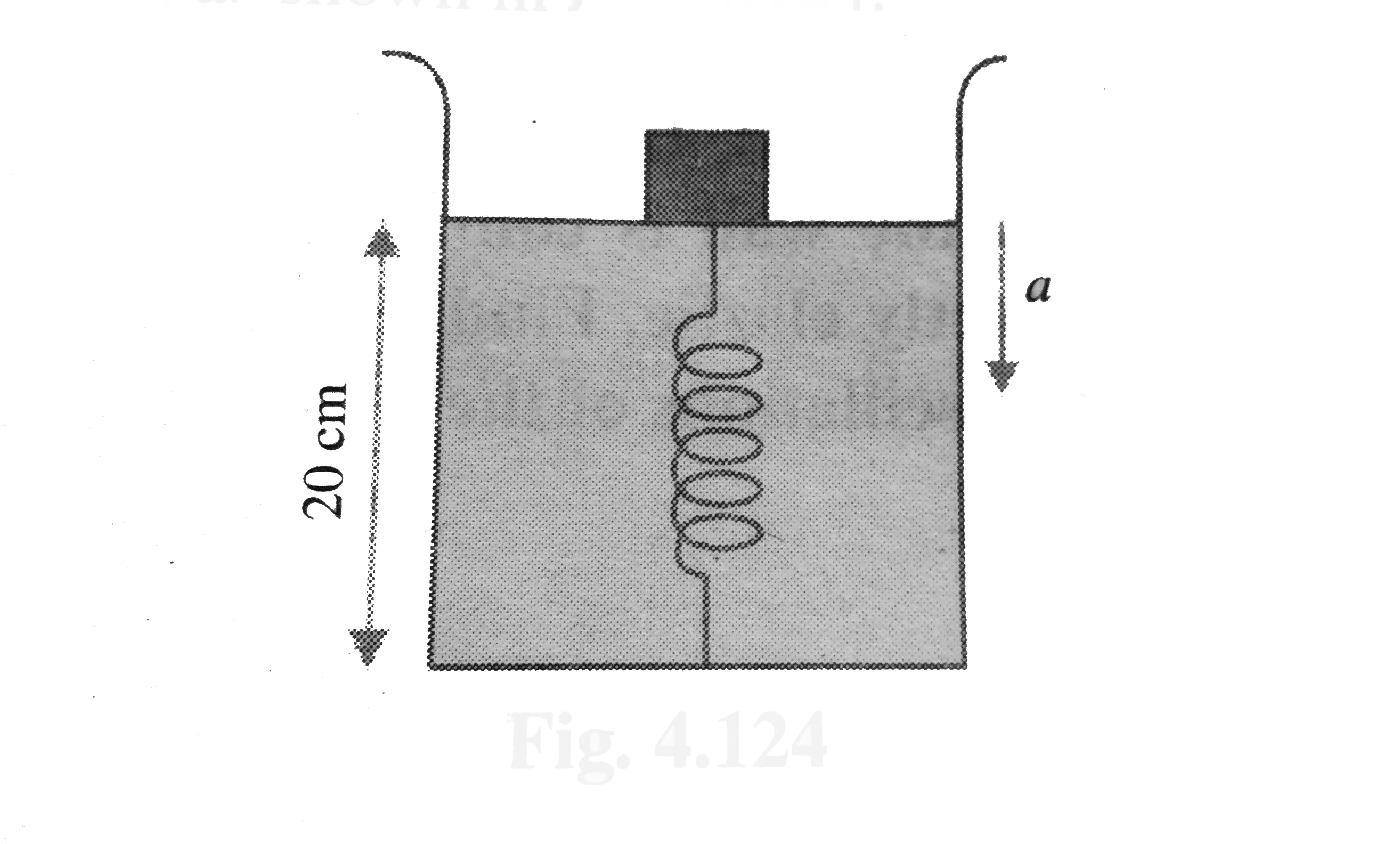
a. Calculate compression of the spring in equilibrium position.
b. If the block is slightly pushed down from equilibrium position and released, calculate frequency of its vertical oscillations.
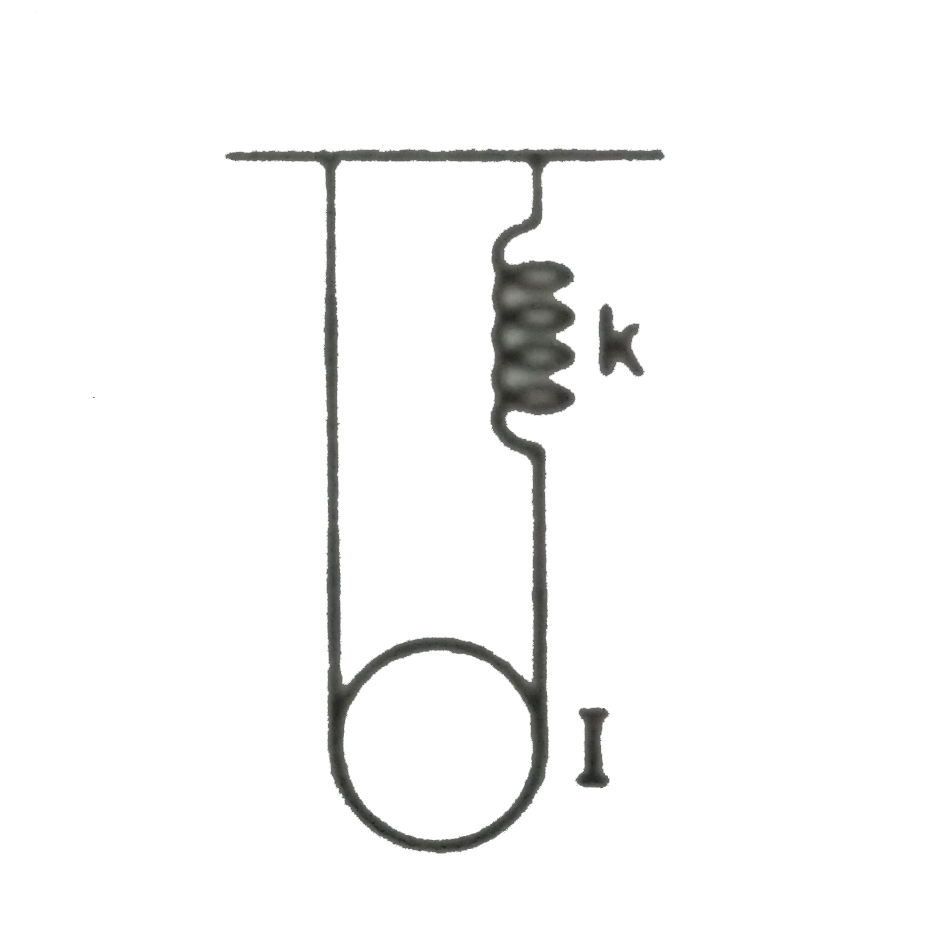
View SolutionThe pulley shown in figure has a moment of inertias I about its axis and mass m. find the time period of vertical oscillation of its centre of mass. The spring has spring constant k and the string does not slip over the pulley.
View SolutionOne end of a 10 cm long silk thread is fixed to a large vertical surface of a charged non-conducting plate and the other end is fastened to a small ball of mass 10 g and charge of 4×10−6C. In equilibrium, thread makes an angle of 60o with the vertical.
(a) Find the tension in the string in equilibrium.
(b) Suppose the ball is slightly pushed aside and released. Find the time period of the small oscillations.
(Use σ=7.5×10−7Cm2)
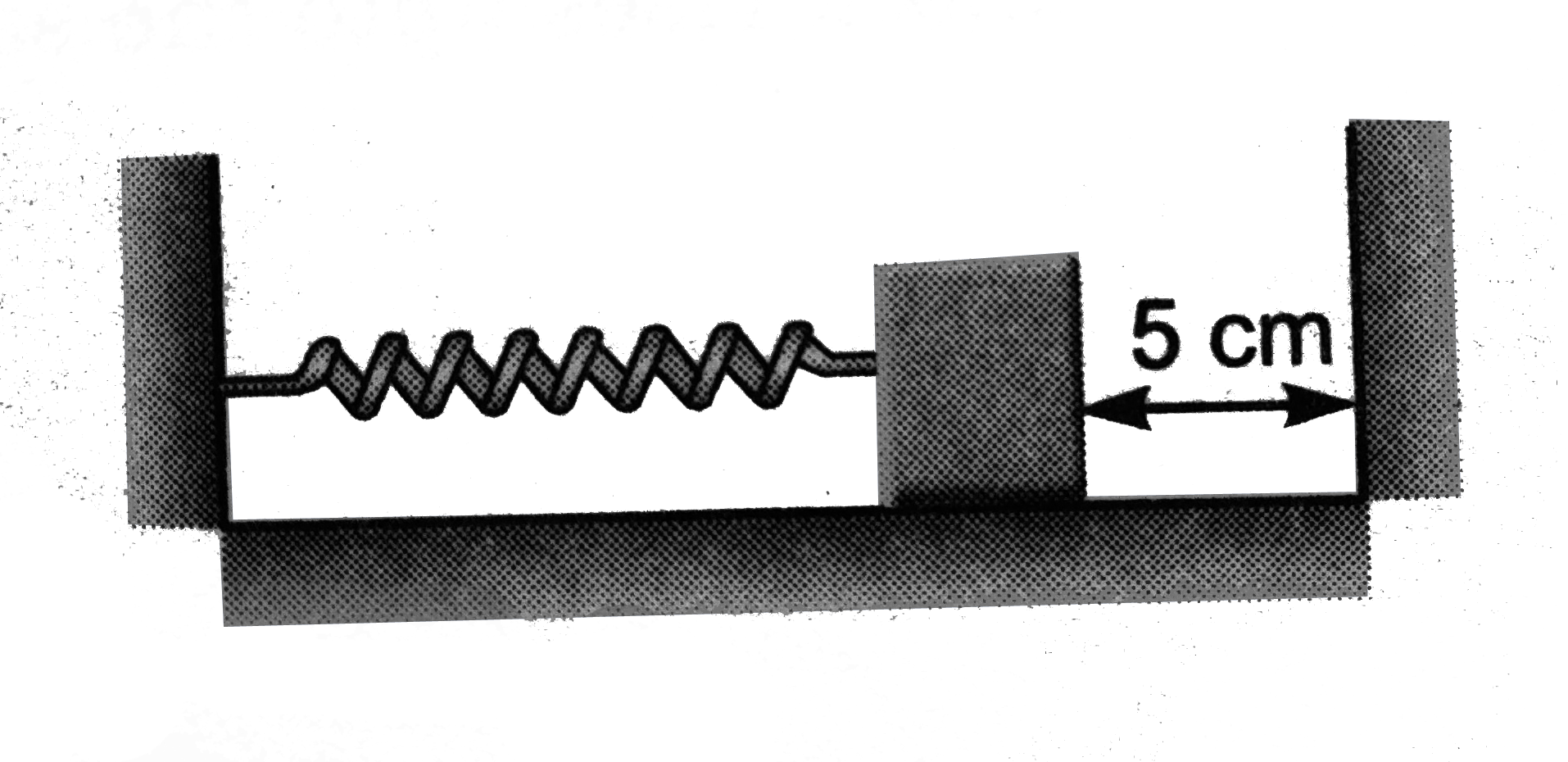
View SolutionA block of mass 100g attached to a spring of stiffness 100N/m is lying on a frictionless floor as shown. The block is moved to compress the spring by 10cm and released. If the collision with the wall is elastic then find the time period of oscillations.
View SolutionA mass of 1.5 kg is connected to two identical springs each of force constant 300Nm−1 as shown in the figure. If the mass is displaced from its equilibrium position by 10cm, then the period of oscillation is

View Solution

