The momentum p of a particle changes with the t according to the relation dpdt=(10N)+(2Ns)t. If the momentum is zero at t=0, what will the momentum be at t=10?.
The correct Answer is:B
To solve the problem, we need to find the momentum p of a particle at t=10 seconds given the rate of change of momentum dpdt=10+2t and the initial condition that p(0)=0.
1. Write down the given equation:
dpdt=10+2t
2. Integrate both sides with respect to time t:
To find p, we need to integrate dpdt:
dp=(10+2t)dt
3. Integrate the right-hand side:
∫dp=∫(10+2t)dt
The integral of dp is simply p. Now we integrate the right-hand side:
p=∫10dt+∫2tdt
p=10t+t2+C
where C is the constant of integration.
4. Use the initial condition to find C:
We know that p(0)=0:
p(0)=10(0)+(0)2+C=0
This implies that C=0.
5. Substitute C back into the equation:
p=10t+t2
6. Calculate p at t=10:
p(10)=10(10)+(10)2
p(10)=100+100=200
7. Final answer:
The momentum at t=10 seconds is:
p(10)=200N⋅s
dpdt=10+2t
To find p, we need to integrate dpdt:
dp=(10+2t)dt
∫dp=∫(10+2t)dt
The integral of dp is simply p. Now we integrate the right-hand side:
p=∫10dt+∫2tdt
p=10t+t2+C
where C is the constant of integration.
We know that p(0)=0:
p(0)=10(0)+(0)2+C=0
This implies that C=0.
p=10t+t2
p(10)=10(10)+(10)2
p(10)=100+100=200
The momentum at t=10 seconds is:
p(10)=200N⋅s
Topper's Solved these Questions
- PHYSICS AND MATHEMATICSBOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Question for short Answer
14 Videos
- PHYSICS AND MATHEMATICSBOOK - HC VERMA ENGLISHCHAPTER - PHYSICS AND MATHEMATICSEXERCISE - Objective 2
5 Videos
- NEWTON'S LAWS OF MOTIONBOOK - HC VERMA ENGLISHCHAPTER - NEWTON'S LAWS OF MOTIONEXERCISE - Questions for short Answer
17 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer
13 Videos
Similar Questions
Knowledge Check
In an inductor of self-inductance L=2 mH, current changes with time according to relation i=t2e−t. At what time emf is zero ?
A4sB3sC2sD1sIn an inductor of self-inductance L=2 mH, current changes with time according to relation i=t2e−t. At what time emf is zero ?
A4sB3sC2sD1s
The momentum P ( in kg ms−1) of a particle is varying with time t ( in second ) as p=2+3t2. The force acting on the particle at t=3s will be
View SolutionThe linear momentum of a particle varies with times as p=a0+at+bt2. What s force and time relation ?
View SolutionThe momentum of a particle of mass of m moving with a velocity v is p= mv. What is the rate of change of momentum ? Assume that m is constant .
View SolutionThe momentum p of an object varies with time (t) as shown in figure. The corresponding force (F)- time (t) graph is -
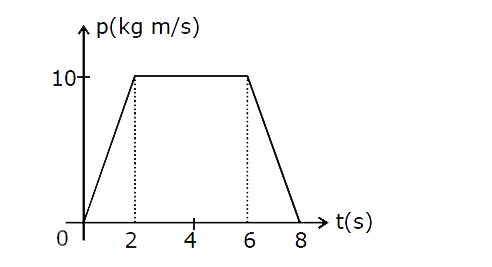
View SolutionThe linear momentum p of a particle is given as a function of time t as p=At2+Bt+C.The dimensions of constant B are
View SolutionThe current in a wire varies with time according to the relation i = 2.0A+(0.6t2)A/s2. How many coulomb of charge pass a cross-section of the wire in the time interval between t = 0 and t = 10s?
View Solution
