Two vectors having equal magnitudes A make an angle θ with eahc other. Find the magnitude and direction of the resultant.
To find the magnitude and direction of the resultant of two vectors having equal magnitudes A that make an angle θ with each other, we can follow these steps:
Step 1: Understand the Problem
We have two vectors →A1 and →A2 with equal magnitudes A and an angle θ between them. We need to find the resultant vector →R in terms of its magnitude and direction.
Hint: Recall the law of cosines for vector addition.
Step 2: Use the Law of Cosines
The magnitude of the resultant vector R can be calculated using the formula:
R=√A2+A2+2A⋅A⋅cos(θ)
Since both vectors have the same magnitude A, we can simplify this to:
R=√2A2+2A2cos(θ)
Hint: Factor out common terms to simplify the expression.
Step 3: Simplify the Expression
Factor out 2A2:
R=√2A2(1+cos(θ))
This can be simplified further:
R=A√2(1+cos(θ))
Hint: Use the trigonometric identity 1+cos(θ)=2cos2(θ2).
Step 4: Apply the Trigonometric Identity
Using the identity:
1+cos(θ)=2cos2(θ2)
we can substitute this into our equation:
R=A√2⋅2cos2(θ2)=A√4cos2(θ2)=2Acos(θ2)
Hint: This gives us the magnitude of the resultant vector.
Step 5: Find the Direction of the Resultant
The direction α of the resultant vector can be found using the tangent function:
tan(α)=Asin(θ)A+Acos(θ)=sin(θ)1+cos(θ)
Hint: Simplify using the identity sin(θ)=2sin(θ2)cos(θ2) and 1+cos(θ)=2cos2(θ2).
Step 6: Simplify the Direction Expression
Substituting the identities:
tan(α)=2sin(θ2)cos(θ2)2cos2(θ2)=tan(θ2)
Thus, we find:
α=θ2
Final Result
The magnitude of the resultant vector is:
R=2Acos(θ2)
And the direction of the resultant vector is:
α=θ2
We have two vectors →A1 and →A2 with equal magnitudes A and an angle θ between them. We need to find the resultant vector →R in terms of its magnitude and direction.
The magnitude of the resultant vector R can be calculated using the formula:
R=√A2+A2+2A⋅A⋅cos(θ)
Since both vectors have the same magnitude A, we can simplify this to:
R=√2A2+2A2cos(θ)
Factor out 2A2:
R=√2A2(1+cos(θ))
This can be simplified further:
R=A√2(1+cos(θ))
Using the identity:
1+cos(θ)=2cos2(θ2)
we can substitute this into our equation:
R=A√2⋅2cos2(θ2)=A√4cos2(θ2)=2Acos(θ2)
The direction α of the resultant vector can be found using the tangent function:
tan(α)=Asin(θ)A+Acos(θ)=sin(θ)1+cos(θ)
Substituting the identities:
tan(α)=2sin(θ2)cos(θ2)2cos2(θ2)=tan(θ2)
Thus, we find:
α=θ2
The magnitude of the resultant vector is:
R=2Acos(θ2)
And the direction of the resultant vector is:
α=θ2
Topper's Solved these Questions
- PHYSICS AND MATHEMATICSBook:HC VERMA ENGLISHChapter:PHYSICS AND MATHEMATICSExercise:work out Example
Explore 18 Videos
- PHYSICS AND MATHEMATICSBook:HC VERMA ENGLISHChapter:PHYSICS AND MATHEMATICSExercise:Objective 1
Explore 6 Videos
- NEWTON'S LAWS OF MOTIONBook:HC VERMA ENGLISHChapter:NEWTON'S LAWS OF MOTIONExercise:Questions for short Answer
Explore 17 Videos
- REST AND MOTION : KINEMATICSBook:HC VERMA ENGLISHChapter:REST AND MOTION : KINEMATICSExercise:Question for short Answer
Explore 13 Videos
Similar Questions
Two vectors having equal magnitudes A make an angle θ with each other. Find the magnitude and direction of the resultant.
View SolutionThe resultant of two vectors , having equal magnitudes E make an angle of 120∘ with each other . What is the magnitude and direction of the resultant ?
View SolutionFind the magnitude and direction of the resultant of given vectors
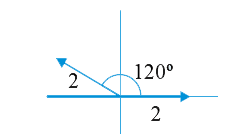
View SolutionTwo vectors having equal magnitude of 5 units, have an angle of 60∘ between them. Find the magnitude of their resultant vector and its angle from one of the vectors.

View SolutionTwo vectors 3 units and 5 units are acting at 60∘ to each other .What is the magnitude and direction of the resultant ?
View SolutionTwo vectors of equal magnitude of 5 unit have an angle 600 between them. Find the magnitude of (a) the sum of the vectors and (b) the difference of the vectors.
 .
.  .
.
View SolutionA car makes a displacement of 100 m towards east and then 200 m towards north. Find the magnitude and direction of the resultant.
View SolutionTwo vectors having magnitude 12 and 13 are inclined at and angle45∘ to each other.find their resultant vector.
View SolutionTwo vectors have magnitudes 6 and 8 units, respectively. Find the magnitude of the resultant vector if the angle between vectors is (a) 60∘ (b) 90∘ and ( c ) 120∘.
View SolutionTwo vectors of equal magnitude are acting through a point. The magnitude of resultant is equal to the magnitude of either vectoe. Find the angle between the vectors.
View Solution