A barometer reads 75cm if mercury. When 2.0cm3 of air at atmospheric pressure is introduced into space above the mercury level, the volume of this space cecones 50cm3. find the length by which the mercury column desecds.
To solve the problem, we will use Boyle's Law, which states that the pressure of a gas multiplied by its volume is a constant when the temperature is held constant. The formula is given by:
P1V1=P2V2
Where:
- P1 = initial pressure
- V1 = initial volume
- P2 = final pressure
- V2 = final volume
1. Identify the Initial Conditions:
- The initial pressure P1 is the atmospheric pressure, which corresponds to the height of the mercury column in the barometer:
P1=75cm of Hg
- The initial volume V1 of the air introduced is:
V1=2cm3
2. Identify the Final Conditions:
- The final volume V2 after introducing the air into the space above the mercury is:
V2=50cm3
- We need to find the final pressure P2.
3. Apply Boyle's Law:
Using Boyle's Law, we can set up the equation:
P1V1=P2V2
Substituting the known values:
75cm Hg×2cm3=P2×50cm3
4. Solve for P2:
Rearranging the equation to solve for P2:
P2=75×250
P2=15050=3cm Hg
5. Determine the Change in Mercury Column Height:
The initial pressure was 75 cm of Hg, and the final pressure is now 3 cm of Hg. The change in height of the mercury column can be calculated as:
Change in height=P1−P2
Change in height=75cm Hg−3cm Hg=72cm Hg
6. Conclusion:
The length by which the mercury column descends is:
Length descended=72cm
Final Answer:
The mercury column descends by 72 cm.
- P1 = initial pressure
- V1 = initial volume
- P2 = final pressure
- V2 = final volume
- The initial pressure P1 is the atmospheric pressure, which corresponds to the height of the mercury column in the barometer:
P1=75cm of Hg
- The initial volume V1 of the air introduced is:
V1=2cm3
- The final volume V2 after introducing the air into the space above the mercury is:
V2=50cm3
- We need to find the final pressure P2.
Using Boyle's Law, we can set up the equation:
P1V1=P2V2
Substituting the known values:
75cm Hg×2cm3=P2×50cm3
Rearranging the equation to solve for P2:
P2=75×250
P2=15050=3cm Hg
The initial pressure was 75 cm of Hg, and the final pressure is now 3 cm of Hg. The change in height of the mercury column can be calculated as:
Change in height=P1−P2
Change in height=75cm Hg−3cm Hg=72cm Hg
The length by which the mercury column descends is:
Length descended=72cm
The mercury column descends by 72 cm.
Topper's Solved these Questions
Similar Questions
A mercury barometer reads 75cm. Now 3cm3 of atmospheric air is introduced into the tube. The mercury falls to a height of 65 cm and the length of air column above the mercury is found to be 15 cm Calculate the cross-sectional area of the barometer tube.
View SolutionA barometer tube is 1 m long and 2cm2 in cross section. Mercury stands to a height of 75cm in the tube. When a small amount of oxygen is introduced in the space above the mercury level, the level falls by 5cm. Calculate the mass of the oxygen is introduced. Room temperature=27∘C, g=10 m s_(-2)anddensityifmercury=13600kg m^(-3).
View SolutionThe mercury manometer consists if two unequal arms of equal cross section 1 cm^(2) and lenghths 100cm and 50cm. The two open ends are sealed with air in the tube at a pressure of 80cm of mercurey. Some amount of mercury is now introduced in the manometer through the stopcock connected to it. If mercury rises in the shorter tube to a lenght 10cm in steady state, find the length of the mercury column risen in the longer tube.
View SolutionA faulty barometer contains certains amount of air and saturated water vapour . It reads 74.0cm when the atmospheric pressure is 76.0cm of mercury and reads 72.10cm when the atmospheric pressure is 74.0cm of mercury.Saturation vapour pressure at air temperature =1.0cm of mercury. Find the length of the baromerer tube above the mercury level in the reservoir.
View SolutionA barometer tube reads 76 cm of mercury. If the tube is gradually inclined keeping the open end immersed in the mercury reservoir, will the length of mercury column be 76 cm, more than 76 cm or less than 76 cm?
View SolutionA barometer tube reads 76 cm of mercury. If the tube is gradually inclined at an angle of 60 with vertical, keeping the open end immersed in the mercury reservoir, the length of the mercury column will be
View SolutionA barometer correctly reads the atmospheric pressure as 76cm of mercury. Water droplets are slowly introduced into the barometer tube by a dropper. The height of the mercury column first decreases and then become constant. If the saturation vapour pressure at the atmospheric temperature is 0.80cm of mercury, find the height if the mercury column when it reaches its minimum value.
View SolutionA barometer tube is 80 cm long (above the mercury reservoir). It reads 76 cm in a particular day. A small amount of water is introduced in the tube and the reading drops to 75.4 cm. Find the relative humidity in the space above the mercury column if the saturation vapor pressure at the room temperature is 1.0cm.
View SolutionA column of mercury is in the middle of horizontal tube of uniform cross section closed at both ends. The pressure of the enclosed air column on either side of the mercury is 75 cm of mercury column. When the tuve is placed vertical the length air below the mercury column is 35 th of that above it. if length of mercury column is 10n cm. find n.
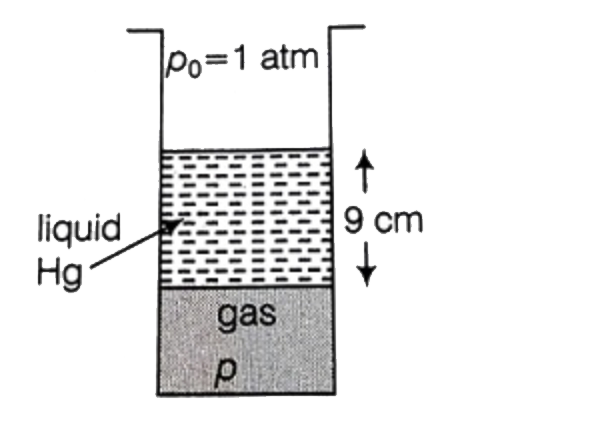
View SolutionIn the given figure, atmospheric pressure p0=1 atm and mercury column length is 9 cm. Pressure p of the gas enclosed in the tube is
View Solution
