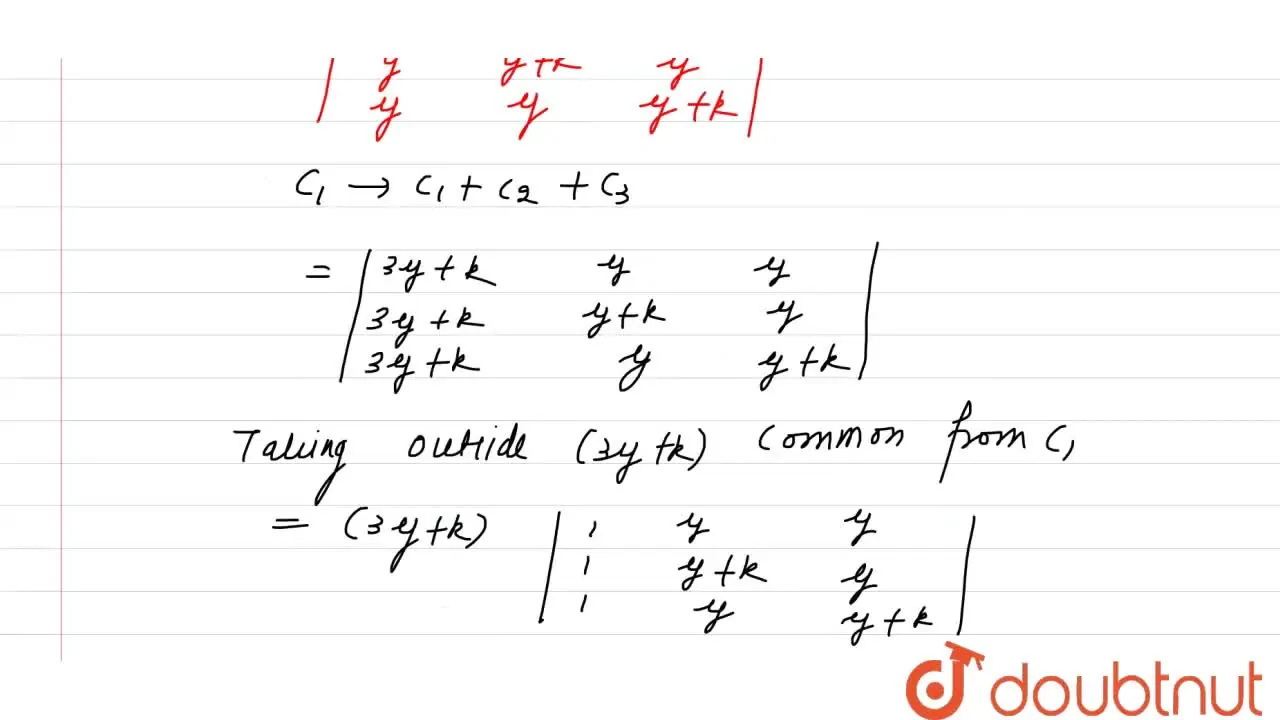
show that ∣∣ ∣ ∣∣y+kyyyy+kyyyy+k∣∣ ∣ ∣∣=k2(3y+k)
Step by step video & image solution for show that |{:(y+k,y,y),(y,y+k,y),(y,y,y+k):}|=k^(2)(3y+k) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
By using properties of determinants. Show that:
(i) ∣∣ ∣∣x+42x2x2xx+42x2x2xx+4∣∣ ∣∣=(5x−4)(4−x)2
(ii) ∣∣ ∣ ∣∣y+kyyyy+kyyyy+k∣∣ ∣ ∣∣=k2(3y+k)View SolutionBy using properties of determinants. Show that: (i) ∣∣ ∣∣x+42x2x2xx+42x2x2xx+4∣∣ ∣∣=(5x−4)(4−x)2 (ii) ∣∣ ∣ ∣∣y+kyyyy+kyyyy+k∣∣ ∣ ∣∣=k2(3y+k)
View SolutionProve, using properties of determinants: ∣∣ ∣ ∣∣y+kyyyy+kyyyy+k∣∣ ∣ ∣∣=k2(3y+k)
View SolutionIf ∣∣ ∣ ∣∣x+yy+zz+xy+zz+xx+yz+xx+yy+z∣∣ ∣ ∣∣=k∣∣ ∣ ∣∣xzyyxzzyx∣∣ ∣ ∣∣, then k is equal to
View SolutionIf ∣∣ ∣ ∣∣xkxk+2xk+3ykyk+2yk+3zkzk+2zk+3∣∣ ∣ ∣∣=(x−y)(y−z)(z−x){1x+1y+1z} then k=
View SolutionBy using properties of determinants. Show that: (i) |x+42x2x2×+42x2x2×+4|=(5x−4)(4−x)2 (ii) |y+kyyyy+kyyyy+k|=k2(2y.k)2
View SolutionIf y2=4ax, then d2ydx2=ka2y3,wherek2=
View SolutionThe differential equation of the family of curves y=k1x2+k2 is given by (where, k1andk2 are arbitrary constants and y1=dydx,y2=d2ydx2)
View SolutionIf x=sint,y=sinKt then show that (1−x2)y2−xy1+K2y=0.
View SolutionThe value of k so that x2+y2+kx+4y+2=0 and 2(x2+y2)−4x−3y+k=0 cut orthogonally, is
View Solution