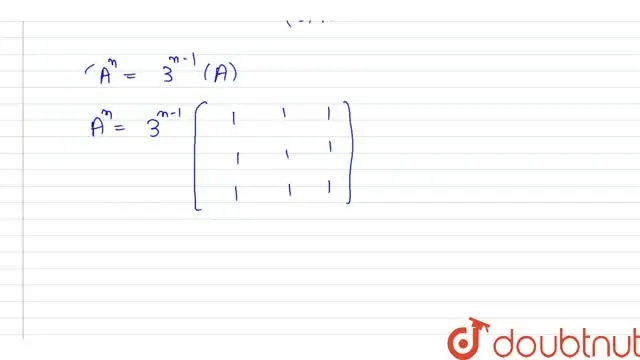
If A=⎡⎢⎣111111111⎤⎥⎦ , then prove that An=∣∣ ∣ ∣∣3n−13n−13n−13n−13n−13n−13n−13n−13n−1∣∣ ∣ ∣∣ for every positive integer n.
Step by step video & image solution for If A=[(1 1 1),( 1 1 1),( 1 1 1)] , then prove that A^n=|(3^(n-1)3^(n-1)3^(n-1)),(3^(n-1)3^(n-1)3^(n-1)),(3^(n-1)3^(n-1)3^(n-1))| for every positive integer ndot by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
If A=⎡⎢⎣111111111⎤⎥⎦ then show that An=⎡⎢ ⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥ ⎥⎦.
View SolutionIf A=⎡⎢⎣111111111⎤⎥⎦, prove that An=⎡⎢ ⎢⎣3n−13n−13n−13n−13n−13n−13n−13n−13n−1⎤⎥ ⎥⎦,n∈N.
View SolutionProve that n∑k=0(−1)k.3nCk=(−1)n.3n−1Cn
View SolutionProve that
11!(n−1)!+13!(n−3)!+15!(n−5)!+…….=2n−1n!View SolutionProve that :1+2+3++n=n(n+1)2
View SolutionIf A=[ab01] , prove that An=[anb(an−1a−1)01] for every positive integer n .
View SolutionIf n ≥ 1 is a positive integer, then prove that 3n≥2n+n.6n−12
View SolutionProve that .n−1C3+.n−1C4>.nC3 if n>7.
View SolutionProve that :12+22+32++n2=n(n+1)(2n+1)6
View SolutionProve that: (2n)!n!={1.3.5(2n−1)}2n.
View Solution