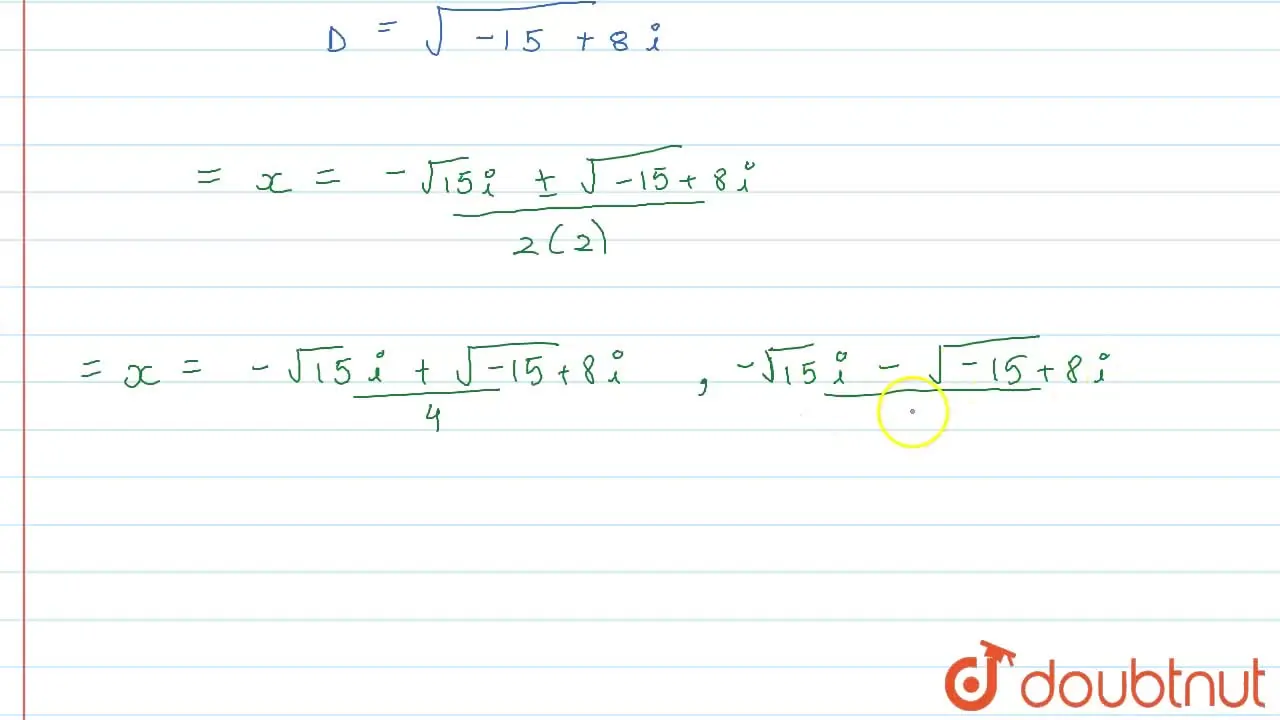
Solve the following quadratic equation: 2x2+√15ix−i=0
Video Solution
|
Answer
Step by step video & image solution for Solve the following quadratic equation: 2x^2+sqrt(15)i x-i=0 by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023
Related Playlists
Similar Questions
Solve the following quadratic equation: x2−(3√2−2i)x−√2i=0
View SolutionSolve the following quadratic equation: x2−(2+i)x−(1−7i)=0
View SolutionSolve the following quadratic equation: x2−(5−i)x+(18+i)=0
View SolutionSolve the following quadratic equation: (2+i)x2−(5−i)x+2(1−i)=0
View SolutionSolve the following quadratic equations.
x2−15x=−26View SolutionSolve the following quadratic equation: x2+4ix−4=0
View SolutionSolve the following quadratic equation: ix2−4x−4i=0
View SolutionSolve the following quadratic equation: ix2−x+12i=0
View SolutionSolve the following quadratic equations.
x2+2x−35=0View SolutionSolve the following quadratic equation:
(3x−5)(2x+3)=0View Solution