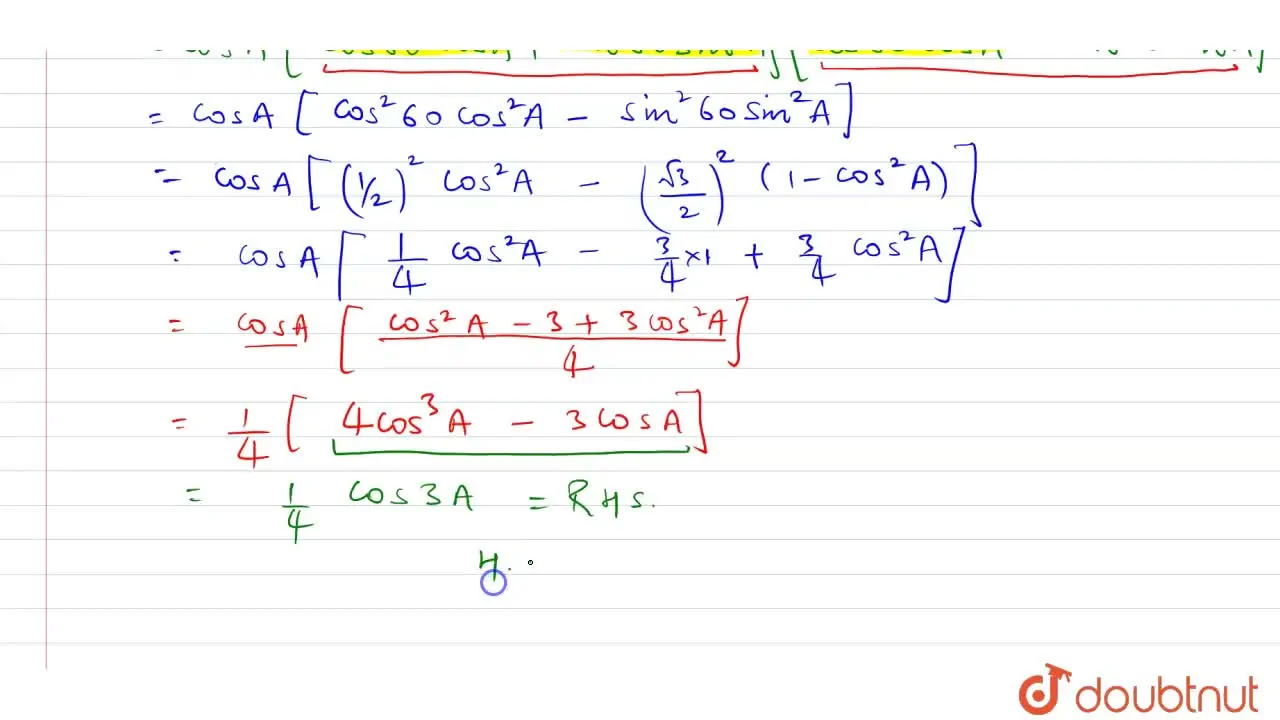
Prove that :cosAcos(60−A)cos(60+A)=14cos3A
Video Solution
|
Answer
Step by step video & image solution for Prove that : cos Acos(60-A)cos(60+A)=1/4cos3A by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023
Related Playlists
Similar Questions
Prove that: cosAcos(600−A)cos(600+A)=14cos3A
View SolutionIf A=30∘, show that :
4cosAcos(60∘−A)⋅cos(60∘+A)=cos3AView SolutionProve that: cos3A+cos5A+cos7A+cos15A=4cos4Acos5Acos6A
View SolutionProve that: cosA+cos3A+cos5A+cos7A=4cosAcos2Acos4A
View SolutionProve that: cos3A+2cos5A+cos7AcosA+2cos3A+cos5A=cos5Acos3A
View SolutionProve that: cos(60∘−A)+sin(30∘−A)cos(30∘−A)−sin(60∘−A)=cotA
View SolutionProve that:
cos(60∘−A)+sin(30∘−A)cos(30∘−A)−sin(60∘−A)=cotAView SolutionProve that: cos4A=1−8cos2 A+8cos4 A
View SolutionProve that: cos4A+cos3A+cos2Asin4A+sin3A+sin2A=cot3A
View SolutionProve that: cos8Acos5A−cos12Acos9Asin8Acos5A+cos12Asin9A=tan4A
View Solution