Find the mean deviation about the median of the following frequency distribution: Class:, 0-6, 6-12, 12-18, 18-24, 24-30 Frequency:, 8, 10, 12, 9, 5
To find the mean deviation about the median for the given frequency distribution, we will follow these steps:
Step 1: Create a Frequency Distribution Table
We have the following classes and frequencies:
| Class | Frequency (f) |
|---------|---------------|
| 0 - 6 | 8 |
| 6 - 12 | 10 |
| 12 - 18 | 12 |
| 18 - 24 | 9 |
| 24 - 30 | 5 |
Step 2: Calculate Mid Values
The mid value (X_i) for each class is calculated as follows:
- For class 0-6: (0 + 6) / 2 = 3
- For class 6-12: (6 + 12) / 2 = 9
- For class 12-18: (12 + 18) / 2 = 15
- For class 18-24: (18 + 24) / 2 = 21
- For class 24-30: (24 + 30) / 2 = 27
| Class | Frequency (f) | Mid Value (X_i) |
|---------|---------------|------------------|
| 0 - 6 | 8 | 3 |
| 6 - 12 | 10 | 9 |
| 12 - 18 | 12 | 15 |
| 18 - 24 | 9 | 21 |
| 24 - 30 | 5 | 27 |
Step 3: Calculate Cumulative Frequency
Now, we will calculate the cumulative frequency (CF):
| Class | Frequency (f) | Cumulative Frequency (CF) |
|---------|---------------|----------------------------|
| 0 - 6 | 8 | 8 |
| 6 - 12 | 10 | 18 |
| 12 - 18 | 12 | 30 |
| 18 - 24 | 9 | 39 |
| 24 - 30 | 5 | 44 |
Step 4: Find the Median
To find the median, we use the formula:
Median=L+N/2−Ff×H
Where:
- L = lower limit of the median class
- N = total frequency
- F = cumulative frequency of the class before the median class
- f = frequency of the median class
- H = width of the class interval
Here, N=44 and N/2=22. The cumulative frequency just greater than 22 is 30, which corresponds to the class 12-18.
Thus:
- L=12
- F=18 (cumulative frequency of the class before the median class)
- f=12 (frequency of the median class)
- H=6 (width of the class interval)
Now substituting the values:
Median=12+22−1812×6
Median=12+412×6
Median=12+2=14
Step 5: Calculate Mean Deviation
The formula for mean deviation about the median is:
Mean Deviation=1N∑(fi|Xi−Median|)
Now, we calculate |Xi−Median| for each mid value:
- For X1=3: |3 - 14| = 11
- For X2=9: |9 - 14| = 5
- For X3=15: |15 - 14| = 1
- For X4=21: |21 - 14| = 7
- For X5=27: |27 - 14| = 13
Now we calculate fi|Xi−Median|:
- For class 0-6: 8×11=88
- For class 6-12: 10×5=50
- For class 12-18: 12×1=12
- For class 18-24: 9×7=63
- For class 24-30: 5×13=65
Now, sum these products:
∑(fi|Xi−Median|)=88+50+12+63+65=278
Finally, substituting into the mean deviation formula:
Mean Deviation=144×278=6.318
Final Answer
The mean deviation about the median is approximately 6.318.
---
We have the following classes and frequencies:
|---------|---------------|
| 0 - 6 | 8 |
| 6 - 12 | 10 |
| 12 - 18 | 12 |
| 18 - 24 | 9 |
| 24 - 30 | 5 |
The mid value (X_i) for each class is calculated as follows:
- For class 6-12: (6 + 12) / 2 = 9
- For class 12-18: (12 + 18) / 2 = 15
- For class 18-24: (18 + 24) / 2 = 21
- For class 24-30: (24 + 30) / 2 = 27
|---------|---------------|------------------|
| 0 - 6 | 8 | 3 |
| 6 - 12 | 10 | 9 |
| 12 - 18 | 12 | 15 |
| 18 - 24 | 9 | 21 |
| 24 - 30 | 5 | 27 |
Now, we will calculate the cumulative frequency (CF):
|---------|---------------|----------------------------|
| 0 - 6 | 8 | 8 |
| 6 - 12 | 10 | 18 |
| 12 - 18 | 12 | 30 |
| 18 - 24 | 9 | 39 |
| 24 - 30 | 5 | 44 |
To find the median, we use the formula:
Median=L+N/2−Ff×H
Where:
- L = lower limit of the median class
- N = total frequency
- F = cumulative frequency of the class before the median class
- f = frequency of the median class
- H = width of the class interval
- L=12
- F=18 (cumulative frequency of the class before the median class)
- f=12 (frequency of the median class)
- H=6 (width of the class interval)
Median=12+22−1812×6
Median=12+412×6
Median=12+2=14
The formula for mean deviation about the median is:
Mean Deviation=1N∑(fi|Xi−Median|)
- For X1=3: |3 - 14| = 11
- For X2=9: |9 - 14| = 5
- For X3=15: |15 - 14| = 1
- For X4=21: |21 - 14| = 7
- For X5=27: |27 - 14| = 13
- For class 0-6: 8×11=88
- For class 6-12: 10×5=50
- For class 12-18: 12×1=12
- For class 18-24: 9×7=63
- For class 24-30: 5×13=65
∑(fi|Xi−Median|)=88+50+12+63+65=278
Mean Deviation=144×278=6.318
The mean deviation about the median is approximately 6.318.
Topper's Solved these Questions
Similar Questions
Find the mean deviation about the median for the following frequency distribution.

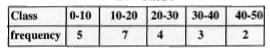
View SolutionFind the mean deviation from the median for following frequency distribution :
View SolutionFind the mean of the following frequency distributions: Class interval: 0-6 6-12 12-18 18-24 24-30 Frequency: 7 5 10 12 6
View SolutionFind the mean of the following frequency distributions: Class interval: 0-6 6-12 12-18 18-24 24-30 Frequency: 6 8 10 9 7
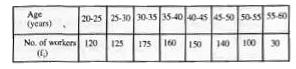
View SolutionFind the mean deviation about median for the following continuous frequency distribution data
View SolutionCalculate the mean deviation about the mean for the following frequency distribution Class interval 0−4,4−8,8−12,12−16,16−20 Frequency 4,6,8,5,2
View SolutionFind the mean of the following frequency distributions: Class interval: 0-8 8-16 16-24 24-32 32-40 Frequency: 6 7 10 8 9
View SolutionFind the mean of the following frequency distributions: Classinterval: 0−8 8−16 16−24 24−32 32−40 Frequency: 5 9 10 8 8
View SolutionCalculate mean deviation from the median of the following data: Class interval: 0-6 6-12 12-18 18-24 24-30 Frequency 4 5 3 6 2
View SolutionCalculate mean deviation from the median of the following data: Class interval: 0-6 6-12 12-18 18-24 24-30 Frequency 4 5 3 6 2
View Solution
