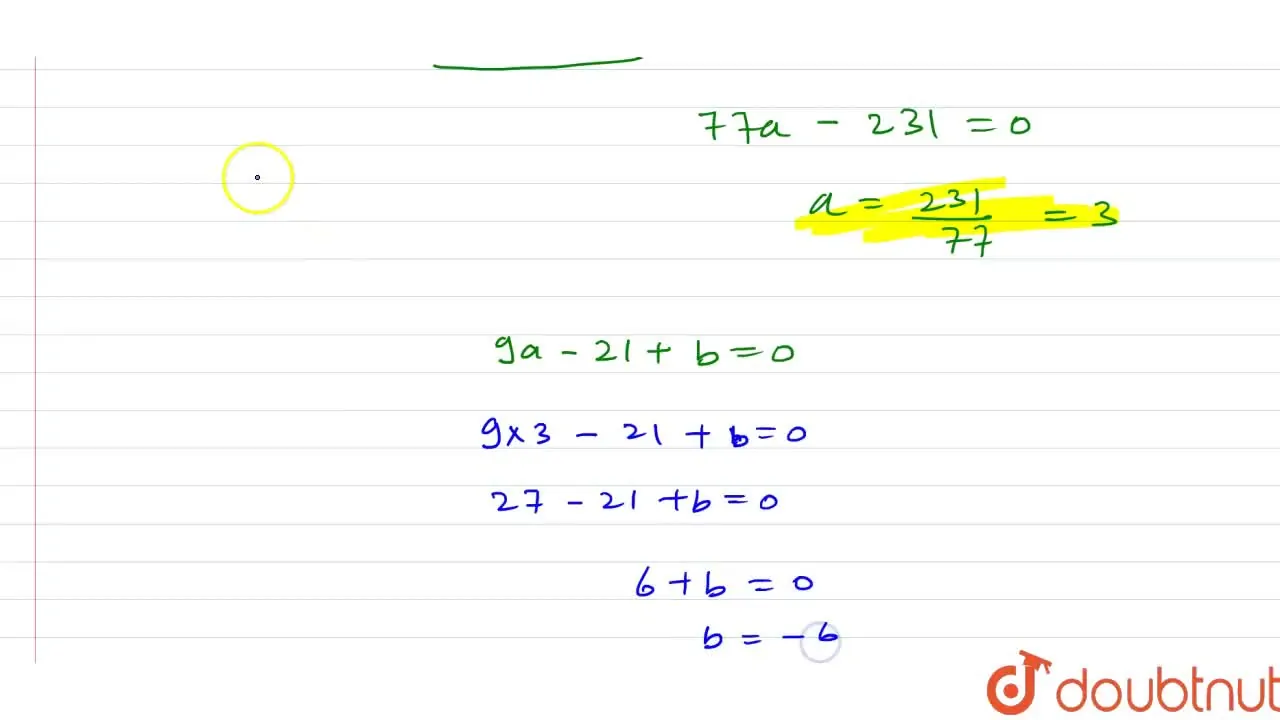
If x=23 and x=−3 are the roots of the equation ax2+7x+b=0, find the values of aandb.
Step by step video & image solution for If x=2/3 and x=-3 are the roots of the equation a x^2+7x+b=0, find the values of a and b dot by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Related Playlists
Similar Questions
Find the roots of the equation x2+7x−1=0
View SolutionFind the roots of the equation 3x2+6x+3=0
View SolutionFind the roots of the equation x2+7x+10=0.
View SolutionIf x=2 andx=3 are roots of the equation 3x2−2kx+2m=0, find the value of k and m.
View SolutionIf x=2 and x=3 are roots of the equation 3x2−2mx+2n=0, find the values of m and n.
View SolutionIf x=2 and x=3 are roots of the equation 3x2−mx+2n=0, then find the values of m and m.
View SolutionIf x=0 and x=−1 are the roots of the polynomial f(x)=2x3−3x2+ax+b, find the value of a and b .
View SolutionIf x=0 and x=−1 are the roots of the polynomial f(x)=2x3−3x2+ax+b, find the value of a and b .
View SolutionFind the roots of the equations.
Q. 2x2+x−3=0View SolutionIf aandb(≠0) are the roots of the equation x2+ax+b=0, then find the least value of x2+ax+b(x∈R).
View Solution